Gases tend to behave non-ideally at low temperatures and high pressures. The deviation from ideal behaviour can be explained by considering two types of corrections. They are volume correction and pressure correction.
Following represents equation of state for n moles of real gas [P+n2aV2][V−nb]=nRT. Select incorrect statement for a real gas
Constant 'a' is a measure of force of attraction among gas molecules
a is expressed in atm L2mol−2 b is expressed in L mol−1
At high pressure, compression factor is (1+PbRT)
n2aV2 is also called internal volume.
The correct Answer is:D
To solve the question regarding the incorrect statement for a real gas based on the Van der Waals equation, we will analyze each statement step by step.
1. Understanding the Van der Waals Equation:
The Van der Waals equation for n moles of a real gas is given by:
(P+n2aV2)(V−nb)=nRT
Here, a and b are constants specific to each gas, where a accounts for the attractive forces between molecules and b accounts for the volume occupied by the gas molecules themselves.
2. Analyzing the First Statement:
- Statement: "Constant A is a measure of force of attraction among gas molecules."
- Evaluation: This statement is correct because the constant a in the equation represents the strength of intermolecular forces.
3. Analyzing the Second Statement:
- Statement: "A is expressed in atmosphere liter square per mole square and B is expressed in liter per mole."
- Evaluation: This statement is also correct. The unit of a can be derived as follows:
Units of a=Pressure×Volume2/Moles2=atm⋅L2/mol2
And for b:
Units of b=Volume/Moles=L/mol
4. Analyzing the Third Statement:
- Statement: "At high pressure, compression factor is 1+PbRT."
- Evaluation: This statement is correct. At high pressures, the term n2aV2 becomes negligible, and the equation simplifies to the ideal gas law, allowing us to express the compressibility factor Z correctly.
5. Analyzing the Fourth Statement:
- Statement: "n square A by V square is called internal volume."
- Evaluation: This statement is incorrect. The term n2aV2 is a pressure correction term, not an internal volume. It accounts for the attractive forces between molecules, which affect the pressure exerted by the gas.
Conclusion:
The incorrect statement is the fourth one: "n square A by V square is called internal volume."
---
The Van der Waals equation for n moles of a real gas is given by:
(P+n2aV2)(V−nb)=nRT
Here, a and b are constants specific to each gas, where a accounts for the attractive forces between molecules and b accounts for the volume occupied by the gas molecules themselves.
- Statement: "Constant A is a measure of force of attraction among gas molecules."
- Evaluation: This statement is correct because the constant a in the equation represents the strength of intermolecular forces.
- Statement: "A is expressed in atmosphere liter square per mole square and B is expressed in liter per mole."
- Evaluation: This statement is also correct. The unit of a can be derived as follows:
Units of a=Pressure×Volume2/Moles2=atm⋅L2/mol2
And for b:
Units of b=Volume/Moles=L/mol
- Statement: "At high pressure, compression factor is 1+PbRT."
- Evaluation: This statement is correct. At high pressures, the term n2aV2 becomes negligible, and the equation simplifies to the ideal gas law, allowing us to express the compressibility factor Z correctly.
- Statement: "n square A by V square is called internal volume."
- Evaluation: This statement is incorrect. The term n2aV2 is a pressure correction term, not an internal volume. It accounts for the attractive forces between molecules, which affect the pressure exerted by the gas.
The incorrect statement is the fourth one: "n square A by V square is called internal volume."
Similar Questions
Gases tend to behave non-ideally at low temperatures and high pressures. The deviation from ideal behaviour can be explained by considering two types of corrections. They are volume correction and pressure correction.
Select incorrect statement(s) :
View SolutionStatement-1: A real gas behaves as an ideal gas at high temperature and low pressure .
Statement-2: Liquid state of an ideal gas is impossible.
View Solution
Knowledge Check
In the corrections made to the ideal gas equation for real gases, the reduction in pressure due to attractive forces is directly proportional to :
AnVBn2V2CV - nbDnbIf P,V,andT represent pressure, volume and temperature of the gas, the correct representation of Boyle's law is
AV∝1T (P constant)BV∝1P (T constant)CPV = RTDPV = nRT
V vs T curves at different pressures P1 and P2 for an ideal gas are shown below:
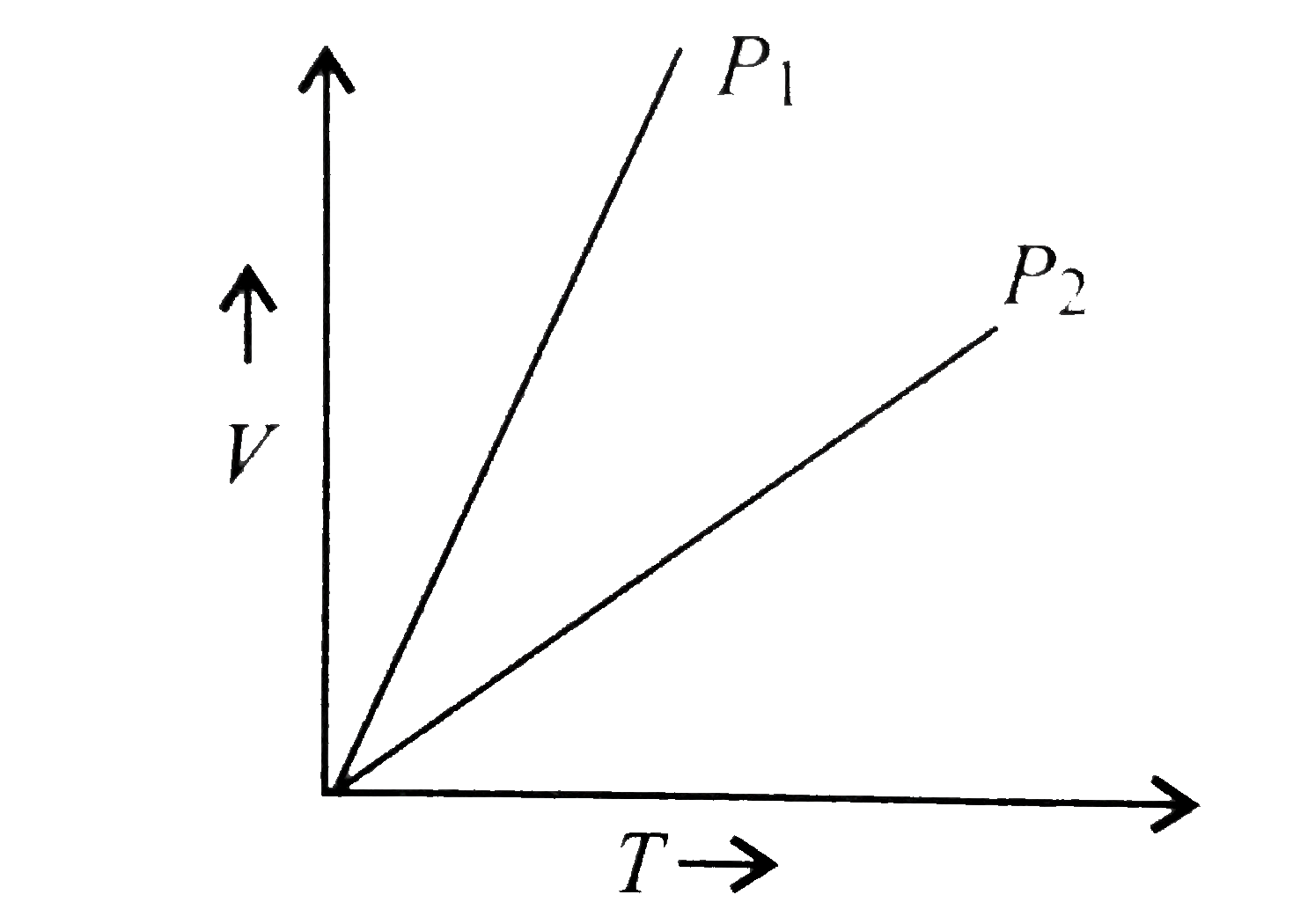
Which one of the following is correct?
View SolutionStatement-1 : At low pressure and high temperature real gas approaches the ideal gas behaviour.
and
Statement-2 : At low prossure and high temperature the molecules are negligible.
View SolutionAssertion An actual gas behaves as an ideal gas most closely at low pressure and high temperature.
Reason At low pressure and high temperature, real gases obey the gasl laws.
View SolutionA: A real gas will approach ideal behaviour at high temperature and low pressure.
R: At low pressure and high temperature z = 1, for all gases
View SolutionWhich of the following volume (V) –temperature (T) plots represents the behaviour of one mole of an ideal gas at one atmospheric pressure?
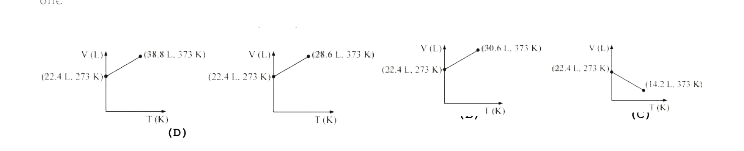
View SolutionAn ideal gas has pressure P, volume V and temperature T. Select the correct option.
View Solution
