P and Q are two arbitrarily chosen intensive variables then
is extensive property
is an intensive variable
PQ is an intensive variable
= intensive variable
To solve the question regarding the intensive variables P and Q, we will analyze each of the provided options step by step.
1. Understanding Intensive and Extensive Properties:
- Intensive Properties: These are properties that do not depend on the amount of substance present. Examples include temperature, pressure, and density.
- Extensive Properties: These properties depend on the amount of substance. Examples include mass, volume, and total energy.
2. Analyzing the Options:
- Option A: P + Q is an extensive property:
- Since both P and Q are intensive properties, their sum (P + Q) will also be an intensive property. Therefore, this statement is false.
- Option B: P/Q is an intensive variable:
- The ratio of two intensive properties is also an intensive property. Thus, P/Q is an intensive variable. Therefore, this statement is true.
- Option C: P * Q is an intensive variable:
- The product of two intensive properties is not necessarily an intensive property. It can be extensive depending on the context. Therefore, this statement is false.
- Option D: DP/DQ is an intensive variable:
- The derivative of two intensive properties (DP/DQ) is also an intensive property. Therefore, this statement is true.
3. Conclusion:
- The correct statements are:
- P/Q is an intensive variable (Option B).
- DP/DQ is an intensive variable (Option D).
Final Answer:
The correct options are B and D.
---
- Intensive Properties: These are properties that do not depend on the amount of substance present. Examples include temperature, pressure, and density.
- Extensive Properties: These properties depend on the amount of substance. Examples include mass, volume, and total energy.
- Option A: P + Q is an extensive property:
- Since both P and Q are intensive properties, their sum (P + Q) will also be an intensive property. Therefore, this statement is false.
- The ratio of two intensive properties is also an intensive property. Thus, P/Q is an intensive variable. Therefore, this statement is true.
- The product of two intensive properties is not necessarily an intensive property. It can be extensive depending on the context. Therefore, this statement is false.
- The derivative of two intensive properties (DP/DQ) is also an intensive property. Therefore, this statement is true.
- The correct statements are:
- P/Q is an intensive variable (Option B).
- DP/DQ is an intensive variable (Option D).
The correct options are B and D.
Similar Questions
If x and y are arbitrary intensive variables, then
View SolutionIf x and y are arbitrary intensive variables, then
View SolutionA point Plies inside the circles x2+y2−4=0andx2+y2−8x+7=0. The poirt P starts moving such that it is always inside the circles, its path enclosus greatest possible area and it is at a fixeddistance from an arbitrarily chosen point in its region. The locus of P is.
View SolutionIf A and B are two fixed points and P is a variable point such that PA+PB=4, the locus of P is
View SolutionLinear equation in two variable
View SolutionIf p and q are two propositions, then ~(p↔q) is
View SolutionS1 and S2 are two equally intense coherent point second sources vibrating in phase. The resultant intensity at P1 is 80SI units then resultant intensity at P2 in SI units will be
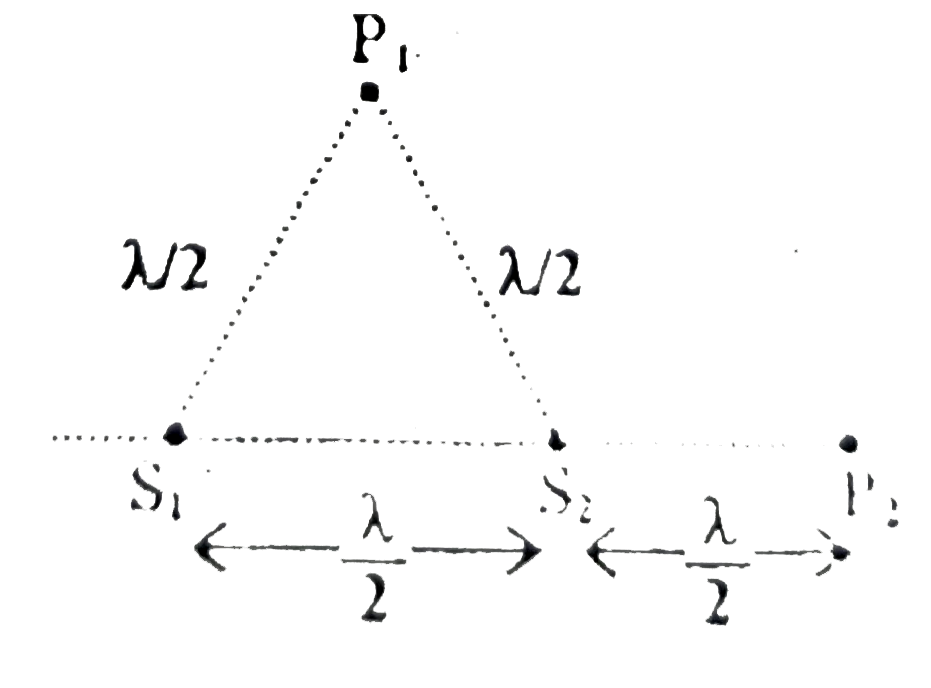
View SolutionG is the geometric mean and p and q are two arithmetic means between two numbers a and b, prove that :
G2=(2p−q)(2q−p)
View SolutionLet p and be two propositions. Then the contrapositive of the implication p→q is
View SolutionIf TP and TQ are the two tangents to a circle with centre O so that ∠POQ=110∘, then ∠PTQ is equal to
View Solution
