A body is projected with a velocity of 30m/s to have to horizontal range of 45m. Find the angle of projection .
To solve the problem of finding the angle of projection for a body projected with a velocity of 30 m/s to achieve a horizontal range of 45 m, we can follow these steps:
Step 1: Understand the Range Formula
The horizontal range R of a projectile is given by the formula:
R=u2sin(2θ)g
where:
- R is the horizontal range,
- u is the initial velocity,
- θ is the angle of projection,
- g is the acceleration due to gravity (approximately 10m/s2).
Step 2: Substitute Known Values
We know:
- R=45m
- u=30m/s
- g=10m/s2
Substituting these values into the range formula gives:
45=302sin(2θ)10
Step 3: Simplify the Equation
First, calculate 302:
302=900
Now substituting this back into the equation:
45=900sin(2θ)10
This simplifies to:
45=90sin(2θ)
Step 4: Solve for sin(2θ)
Rearranging the equation to isolate sin(2θ):
sin(2θ)=4590=12
Step 5: Find 2θ
Now, we need to find 2θ using the inverse sine function:
2θ=sin−1(12)
We know that:
sin−1(12)=30∘
Thus:
2θ=30∘
Step 6: Calculate θ
Now, divide by 2 to find θ:
θ=30∘2=15∘
Final Answer
The angle of projection θ is:
θ=15∘
---
The horizontal range R of a projectile is given by the formula:
R=u2sin(2θ)g
where:
- R is the horizontal range,
- u is the initial velocity,
- θ is the angle of projection,
- g is the acceleration due to gravity (approximately 10m/s2).
We know:
- R=45m
- u=30m/s
- g=10m/s2
45=302sin(2θ)10
First, calculate 302:
302=900
Now substituting this back into the equation:
45=900sin(2θ)10
This simplifies to:
45=90sin(2θ)
Rearranging the equation to isolate sin(2θ):
sin(2θ)=4590=12
Now, we need to find 2θ using the inverse sine function:
2θ=sin−1(12)
We know that:
sin−1(12)=30∘
Thus:
2θ=30∘
Now, divide by 2 to find θ:
θ=30∘2=15∘
The angle of projection θ is:
θ=15∘
Topper's Solved these Questions
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - NUMERICAL EXERCISE (LEVEL -2)
19 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - QUESTION FOR DESCRIPTIVE ANSWER
7 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - VERY SHORT ANSWER TYPE QUESTIONS
10 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - EXERCISE-3 (Circular Motion)
7 Videos
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - very Short answer type question
15 Videos
Similar Questions
A particle is projected with a velocity 10 m//s at an angle 37∘ to the horizontal. Find the location at which the particle is at a height 1 m from point of projection.
View SolutionA body is projected with a velocity u at an angle of 60∘ to the horizontal. The time interval after which it will be moving in a direction of 30∘ to the horizontal is
View Solution
Knowledge Check
If a body is projected with an angle θ to the horizontal, then
Aits velocity is always perpendicular to its acceleration thenBits velocity becomes zero at its maximum height.Cits velocity makes zero angle with the horizontal at its maximum height.Dthe body just before hitting the ground, the direction of velocity coincides with the acceleration.
A body projected at 45∘ with a velocity of 20 m/s has a range of 10m. The decrease in range due to air resistance is (g=10ms−1)
View SolutionA ball of mass 200g is projected with a density of 30m/s at 30∘ from horizontal. Using the concept of impulse, find change in velocity in 2s. Take g=10m/s2.
View SolutionA body is projected with an initial Velocity 20 m/s at 60° to the horizontal. Its velocity after 1 sec is
View SolutionA body is projected with velocity u such that in horizontal range and maximum vertical heights are same.The maximum height is
View SolutionA particle A is projected with an initial velocity of 60m/s at an angle 30∘ to the horizontal. At the same time a second particle B is projected in opposite direction with initial speed of 50m/s from a point at a distance of 100 m from A. If the particles collide in air, find (a)the angle of projection α of particle B, (b) time when the collision takes place and (c) the distance of P from A, where collision occurs. (g=10m/s2)
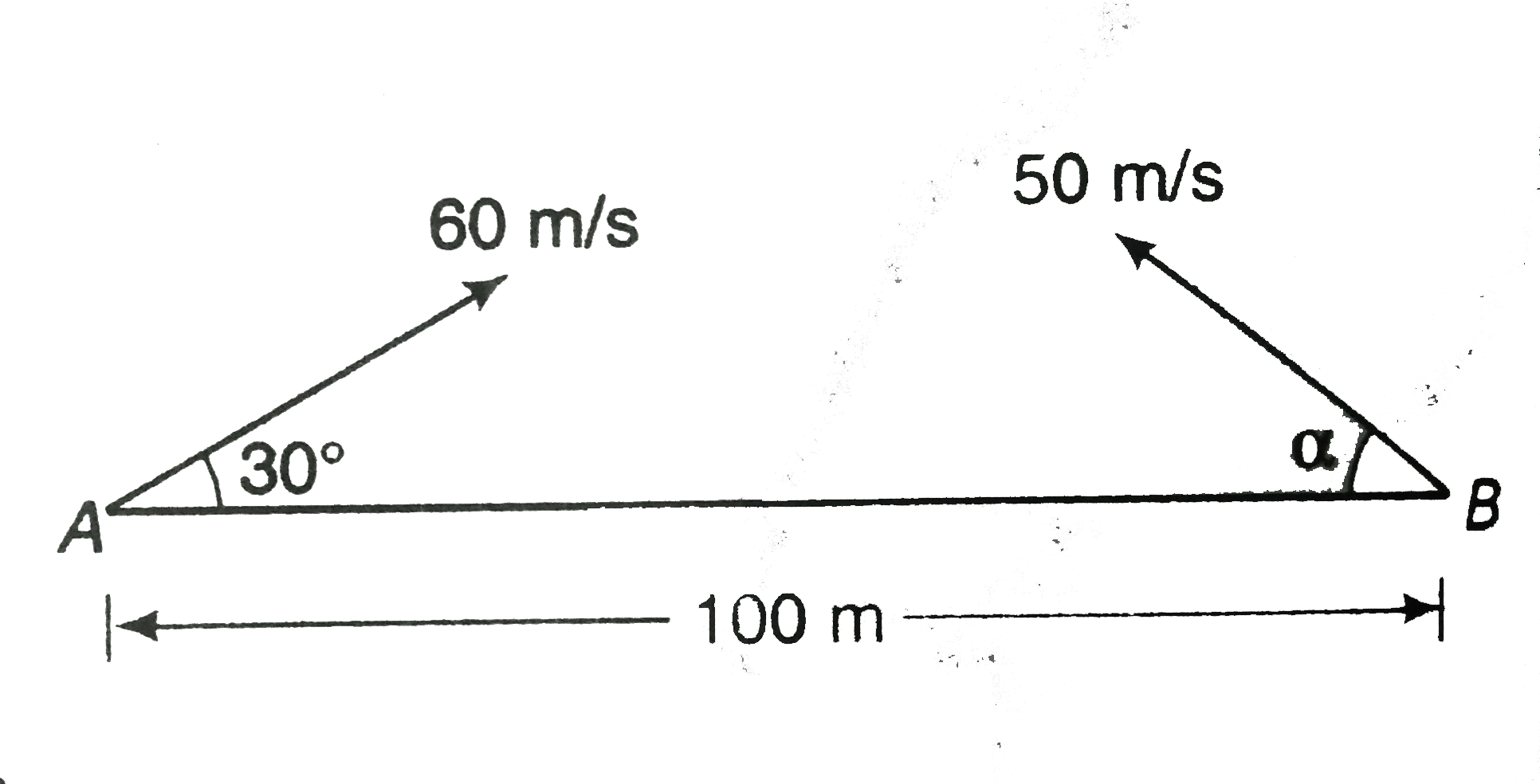
View SolutionAn object is projected with a velocity of 30ms−1 at an angle of 60∘ with the horizontal. Determine the horizontal range covered by the object.
View SolutionA body is projected with velocity 60m/sec at 30 degree horizontal, its initial velocity vector is ? In the above problem velocity after 3 seconds is-
View Solution
