A body is projected with an initial elocity 20m/s at 60° to the horizontal. The displacement after 2 s is
20[i+(√3−1)i]
20[1−(√3−1)j]
10[i+(√3+1)j]
10[1+(√3−1)]
The correct Answer is:A
To solve the problem step by step, we need to find the displacement of a body projected with an initial velocity of 20 m/s at an angle of 60° to the horizontal after 2 seconds.
Step 1: Determine the initial velocity components
The initial velocity U is given as 20 m/s. We need to find the horizontal and vertical components of this velocity.
- Horizontal component UH:
UH=Ucos(60°)=20cos(60°)=20×12=10m/s
- Vertical component UV:
UV=Usin(60°)=20sin(60°)=20×√32=10√3m/s
Step 2: Calculate horizontal displacement after 2 seconds
Since there is no acceleration in the horizontal direction, the horizontal displacement SH can be calculated using the formula:
SH=UH×T
Substituting the values:
SH=10m/s×2s=20m
Step 3: Calculate vertical displacement after 2 seconds
For vertical displacement SV, we use the equation:
SV=UV×T−12gT2
Where g (acceleration due to gravity) is approximately 10m/s2. Substituting the values:
SV=(10√3m/s)×2s−12×10m/s2×(2s)2
Calculating each term:
SV=20√3−12×10×4
SV=20√3−20
Step 4: Combine horizontal and vertical displacements
Now we can express the total displacement vector →S in terms of its components:
→S=SH^i+SV^j
Substituting the values we found:
→S=20^i+(20√3−20)^j
Step 5: Final expression for displacement
Thus, the displacement after 2 seconds can be written as:
→S=20^i+(20√3−20)^j
The initial velocity U is given as 20 m/s. We need to find the horizontal and vertical components of this velocity.
UH=Ucos(60°)=20cos(60°)=20×12=10m/s
UV=Usin(60°)=20sin(60°)=20×√32=10√3m/s
Since there is no acceleration in the horizontal direction, the horizontal displacement SH can be calculated using the formula:
SH=UH×T
Substituting the values:
SH=10m/s×2s=20m
For vertical displacement SV, we use the equation:
SV=UV×T−12gT2
Where g (acceleration due to gravity) is approximately 10m/s2. Substituting the values:
SV=(10√3m/s)×2s−12×10m/s2×(2s)2
Calculating each term:
SV=20√3−12×10×4
SV=20√3−20
Now we can express the total displacement vector →S in terms of its components:
→S=SH^i+SV^j
Substituting the values we found:
→S=20^i+(20√3−20)^j
Thus, the displacement after 2 seconds can be written as:
→S=20^i+(20√3−20)^j
Similar Questions
A body is projected with an initial Velocity 20 m/s at 60° to the horizontal. Its velocity after 1 sec is
View SolutionA body is projected with an initial Velocity 20 m/s at 60° to the horizontal. Its initial velocity vector is- (g=10 m/s2)
View SolutionA particle A is projected with an initial velocity of 60m/s at an angle 30∘ to the horizontal. At the same time a second particle B is projected in opposite direction with initial speed of 50m/s from a point at a distance of 100 m from A. If the particles collide in air, find (a)the angle of projection α of particle B, (b) time when the collision takes place and (c) the distance of P from A, where collision occurs. (g=10m/s2)
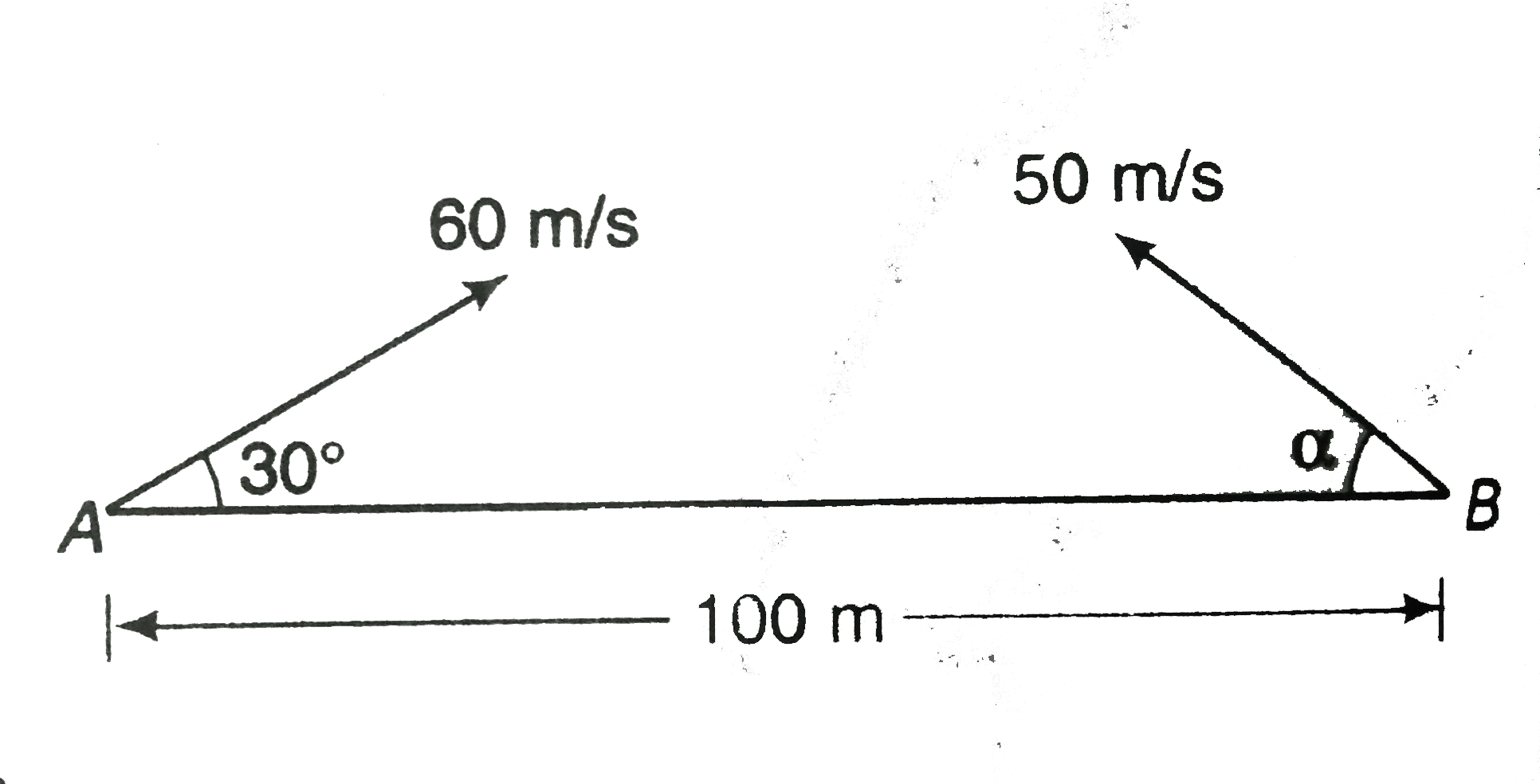
View SolutionA body is projected with a velocity u at an angle of 60∘ to the horizontal. The time interval after which it will be moving in a direction of 30∘ to the horizontal is
View SolutionA body is projected with an initial velocity of 58.8 m/s at angle 60° with the vertical. The vertical component of velocity after 2 sec is
View SolutionA body is projected with a initial velocity of (8ˆi+6ˆj)ms−1 . The horizontal range is (g=10ms−2)
View SolutionA particle is projected with a velocity of 50 m/s at 37∘ with horizontal. Find velocity, displacement and co-ordinates of the particle (w.r.t. the starting point) after 2 s.
Given, g=10m/s2,sin37∘=0.6andcos37∘=0.8.
View SolutionA body is projected with a velocity 60ms−1at30∘ to horizontal . Its initial velocity vector is
View SolutionA particle is projected from ground with velocity 50m/s at 37∘ from horizontal. Find velocity and displacement after 2 s. sin37∘=35.
View SolutionA particle is projected with speed 10m/s at angle 60∘ with the horizontal. Then the time after which its speed becomes half of initial.
View Solution
