A body is projected with an initial Velocity 20 m/s at 60° to the horizontal. Its initial velocity vector is- (g=10 )
The correct Answer is:C
To solve the problem of a body projected with an initial velocity of 20 m/s at an angle of 60° to the horizontal, we need to break down the initial velocity into its horizontal (x) and vertical (y) components.
1. Identify the Given Values:
- Initial velocity (u) = 20 m/s
- Angle of projection (θ) = 60°
- Acceleration due to gravity (g) = 10 m/s² (though we won't need it for finding the initial velocity components)
2. Calculate the Horizontal Component of Velocity (u_x):
- The horizontal component can be calculated using the cosine function:
ux=u⋅cos(θ)
- Substitute the values:
ux=20⋅cos(60°)
- Since cos(60°)=12:
ux=20⋅12=10 m/s
3. Calculate the Vertical Component of Velocity (u_y):
- The vertical component can be calculated using the sine function:
uy=u⋅sin(θ)
- Substitute the values:
uy=20⋅sin(60°)
- Since sin(60°)=√32:
uy=20⋅√32=10√3 m/s
4. Form the Initial Velocity Vector:
- The initial velocity vector can be expressed in terms of its components:
→u=ux^i+uy^j
- Substitute the values of ux and uy:
→u=10^i+10√3^j m/s
5. Final Answer:
- The initial velocity vector is:
→u=10^i+10√3^j m/s
- Initial velocity (u) = 20 m/s
- Angle of projection (θ) = 60°
- Acceleration due to gravity (g) = 10 m/s² (though we won't need it for finding the initial velocity components)
- The horizontal component can be calculated using the cosine function:
ux=u⋅cos(θ)
- Substitute the values:
ux=20⋅cos(60°)
- Since cos(60°)=12:
ux=20⋅12=10 m/s
- The vertical component can be calculated using the sine function:
uy=u⋅sin(θ)
- Substitute the values:
uy=20⋅sin(60°)
- Since sin(60°)=√32:
uy=20⋅√32=10√3 m/s
- The initial velocity vector can be expressed in terms of its components:
→u=ux^i+uy^j
- Substitute the values of ux and uy:
→u=10^i+10√3^j m/s
- The initial velocity vector is:
→u=10^i+10√3^j m/s
Similar Questions
A body is projected with an initial Velocity 20 m/s at 60° to the horizontal. Its velocity after 1 sec is
View SolutionA body is projected with a velocity 60ms−1at30∘ to horizontal . Its initial velocity vector is
View SolutionA body is projected with an initial elocity 20m/s at 60° to the horizontal. The displacement after 2 s is
View SolutionA body is projected with velocity 60m/sec at 30 degree horizontal, its initial velocity vector is ? In the above problem velocity after 3 seconds is-
View SolutionA body is projected with an initial velocity of 58.8 m/s at angle 60° with the vertical. The vertical component of velocity after 2 sec is
View SolutionA particle A is projected with an initial velocity of 60m/s at an angle 30∘ to the horizontal. At the same time a second particle B is projected in opposite direction with initial speed of 50m/s from a point at a distance of 100 m from A. If the particles collide in air, find (a)the angle of projection α of particle B, (b) time when the collision takes place and (c) the distance of P from A, where collision occurs. (g=10m/s2)
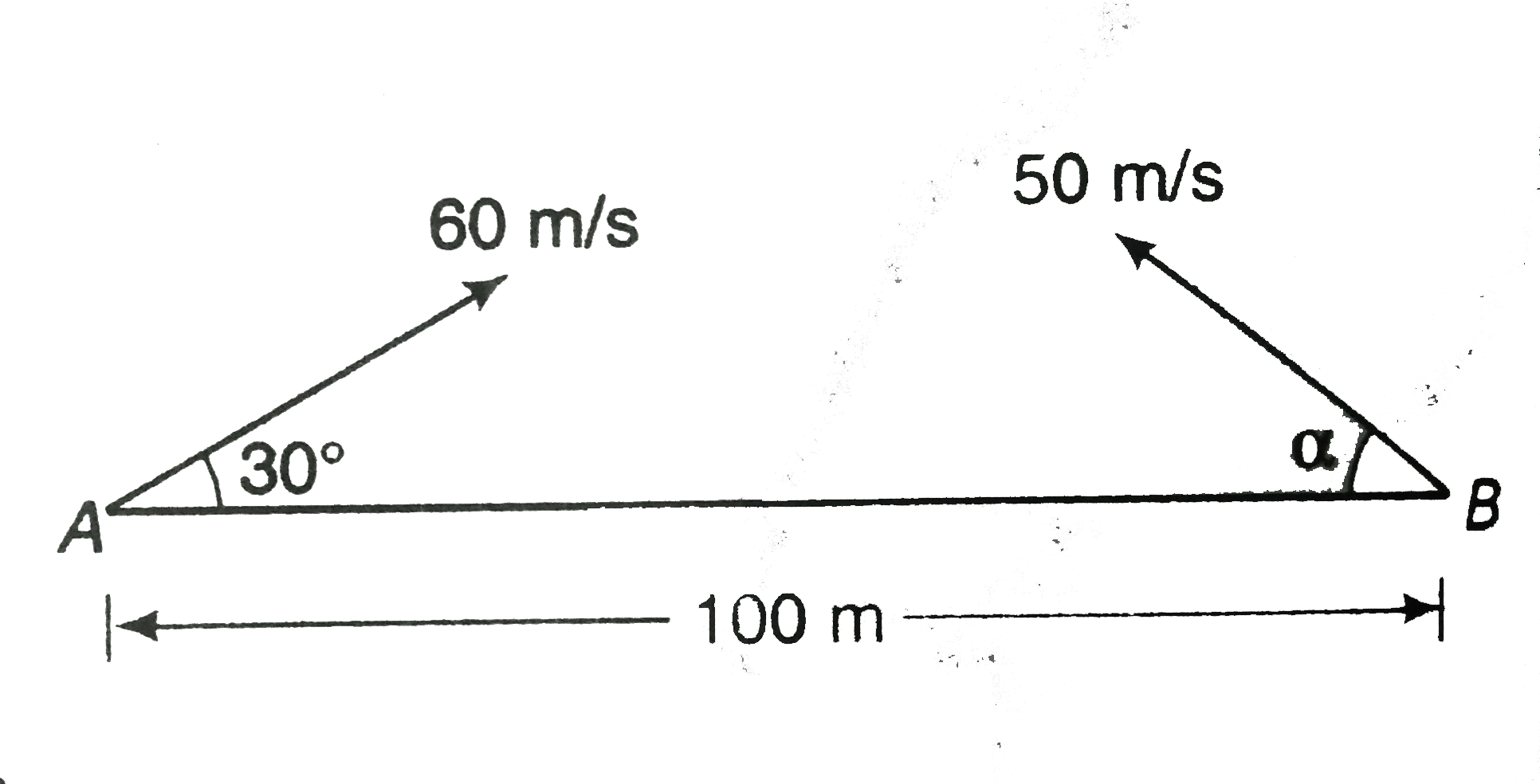
View SolutionA body is projected with a initial velocity of (8ˆi+6ˆj)ms−1 . The horizontal range is (g=10ms−2)
View SolutionA body is projected with a velocity of 30m/s to have to horizontal range of 45m. Find the angle of projection .
View SolutionAn electric field is acting vertically upwards . A particle of mass 1 mg and charge −1μC is projected with a velocity 20m/s at an angle 45∘ with the horizontal . Its horizontal range is 10 m, then the intensity of electric field is (g=10m/s2)
View SolutionA ball is projected with a velocity 20√3ms−1 at angle 60∘ to the horizontal. The time interval after which the velocity vector will make an angle 30∘ to the horizontal is (Take, g=10ms−2)
View Solution
