A shower of rain appears to fall vertically downwards with a velocity of 12 kmph on a person walking west wards with a velocity of 5 kmph. The actual velocity and direction of the rain are
7.5 kmph, clockwise to vertical
13 kmph, anti clockwise to vertical
13 kmph, clockwise to vertical
17 kmph, clockwise to vertical
The correct Answer is:C
Step by step video & image solution for A shower of rain appears to fall vertically downwards with a velocity of 12 kmph on a person walking west wards with a velocity of 5 kmph. The actual velocity and direction of the rain are by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
Rain is falling vertically downwards with a velocity of 2m//s. A person is runing to the North with a velocity of 2√3m/s. Find out the velocity and direction of rain as appeared to the person.
View SolutionA man moves on a cycle with a velocity of 4 km/hr. The rain appears to fall on him with a velocity of 3 km/hr vertically. The actual velocity of the rain is
View SolutionRain is falling vertically downwards with a speed of 4kmh−1. A girl moves on a straight road with a velocity of 3kmh−1. The apparent velocity of rain with respect to the girl is.
View SolutionRain is falling vertically with a velocity of 3kmh−1. A man walks in the rain with a velocity of 4kmh−1. The rain drops will fall on the man with a velocity of
View SolutionRain is falling vertically with a velocity of 3kmh−1. A man walks in the rain with a velocity of 4kmh−1. The rain drops will fall on the man with a velocity of
View SolutionAn object is falling vertically downwards with velocity 10m/s.In terms ofˆiandˆj find the image velocity. M
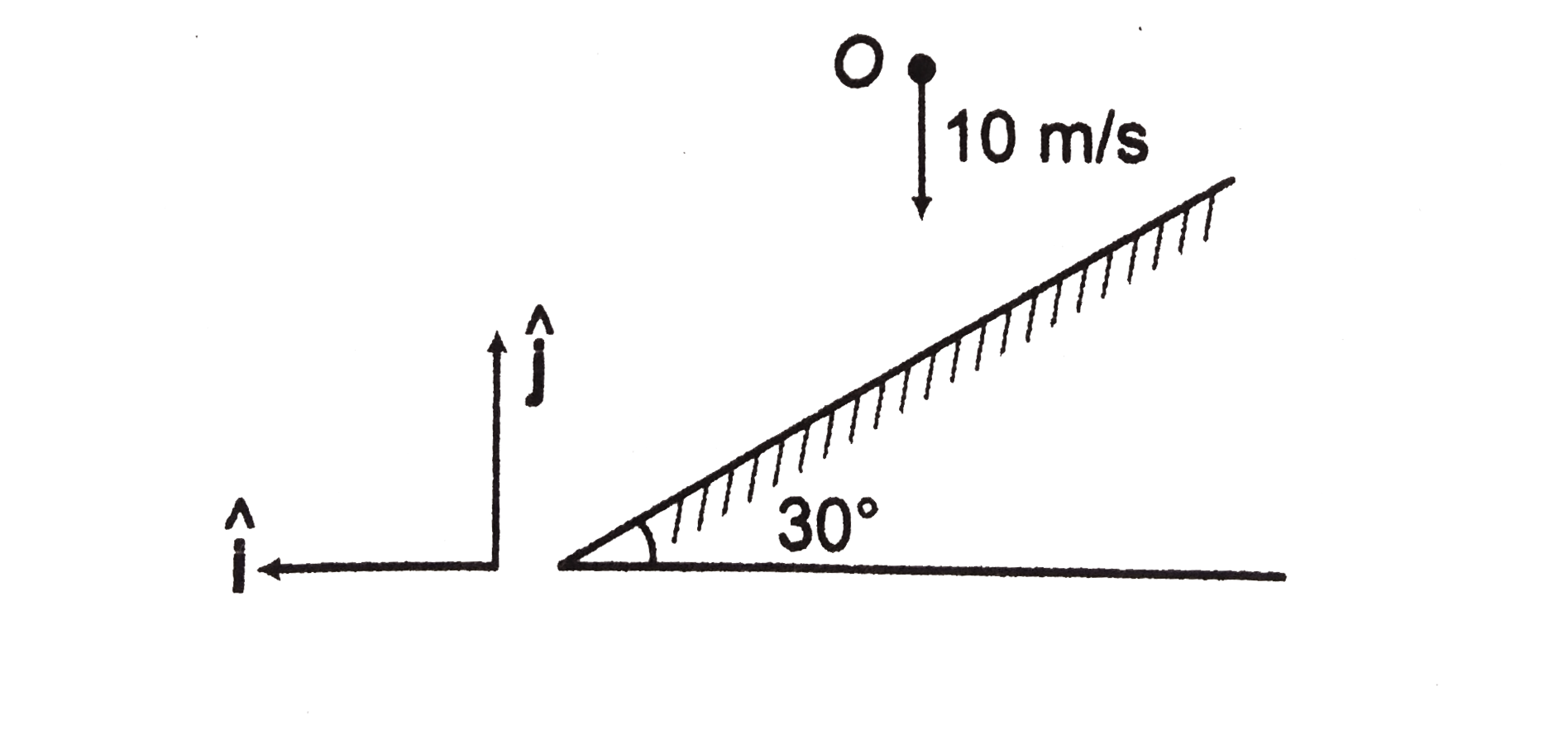 View Solution
View SolutionRain is falling vertically downward with velocity 4m/s. A man is moving horizontally with velocity 3m/s, the velocity of rain with respect to man is
View SolutionAn observer is travelling in west with velocity of 5 km/h and observes that wind is blowing in north with the velocity of 5√3 km/h. Find out the actual velocity of wind.
View SolutionRain is falling vertically with a velocity of 4 km hr−1 . A cyclist is going along a horizontal road with a velocity of 3 km hr−1 towards cast. Calculate the relative velocity of the rain with respect to the cyclist. In what direction must the cyclist hold his umbrella to save himself from the falling rain ?
View SolutionA man running on the horizontal road at 8kmh−1 find the rain appears to be falling vertically. He incresases his speed to 12kmh−1 and find that the drops make angle 302 with the vertical. Find the speed and direction of the rain with respedt to the road.
View Solution
