Rain is falling vertically downwards with a velocity of 2m//s. A person is runing to the North with a velocity of 2√3m/s. Find out the velocity and direction of rain as appeared to the person.
The correct Answer is:4 m/s In south direction, at 60∘ with vertical
To solve the problem of finding the velocity and direction of rain as perceived by a person running to the north, we can follow these steps:
Step 1: Define the velocities
1. The velocity of the rain, →Vrain, is given as 2m/s vertically downwards. In vector form, this can be represented as:
→Vrain=0^i+0^j−2^km/s
(where ^k is the unit vector in the vertical direction).
2. The velocity of the person running to the north is given as 2√3m/s. In vector form, this can be represented as:
→Vperson=0^i+2√3^j+0^km/s
(where ^j is the unit vector in the north direction).
Step 2: Calculate the relative velocity of the rain with respect to the person
To find the velocity of the rain as perceived by the person, we need to subtract the person's velocity from the rain's velocity:
→Vrain, relative=→Vrain−→Vperson
Substituting the values:
→Vrain, relative=(0^i+0^j−2^k)−(0^i+2√3^j+0^k)
This simplifies to:
→Vrain, relative=0^i+(−2−2√3)^j+(−2)^k
Thus, we have:
→Vrain, relative=0^i+(−2√3)^j−2^k
Step 3: Calculate the magnitude of the relative velocity
The magnitude of the relative velocity can be calculated using the Pythagorean theorem:
|→Vrain, relative|=√(0)2+(−2√3)2+(−2)2
Calculating the squares:
|→Vrain, relative|=√0+12+4=√16=4m/s
Step 4: Determine the direction of the relative velocity
To find the direction, we can express the relative velocity vector in terms of its components:
→Vrain, relative=0^i+(−2√3)^j−2^k
The direction can be described in terms of angles or as a vector in the j−k plane. The angle θ with respect to the vertical can be calculated using:
tan(θ)=|vertical component||horizontal component|
Since the horizontal component is zero, the angle is simply downward.
Final Answer
The velocity of the rain as perceived by the person is 4m/s directed at an angle downward towards the vertical.
---
1. The velocity of the rain, →Vrain, is given as 2m/s vertically downwards. In vector form, this can be represented as:
→Vrain=0^i+0^j−2^km/s
(where ^k is the unit vector in the vertical direction).
→Vperson=0^i+2√3^j+0^km/s
(where ^j is the unit vector in the north direction).
To find the velocity of the rain as perceived by the person, we need to subtract the person's velocity from the rain's velocity:
→Vrain, relative=→Vrain−→Vperson
Substituting the values:
→Vrain, relative=(0^i+0^j−2^k)−(0^i+2√3^j+0^k)
This simplifies to:
→Vrain, relative=0^i+(−2−2√3)^j+(−2)^k
Thus, we have:
→Vrain, relative=0^i+(−2√3)^j−2^k
The magnitude of the relative velocity can be calculated using the Pythagorean theorem:
|→Vrain, relative|=√(0)2+(−2√3)2+(−2)2
Calculating the squares:
|→Vrain, relative|=√0+12+4=√16=4m/s
To find the direction, we can express the relative velocity vector in terms of its components:
→Vrain, relative=0^i+(−2√3)^j−2^k
The direction can be described in terms of angles or as a vector in the j−k plane. The angle θ with respect to the vertical can be calculated using:
tan(θ)=|vertical component||horizontal component|
Since the horizontal component is zero, the angle is simply downward.
The velocity of the rain as perceived by the person is 4m/s directed at an angle downward towards the vertical.
Topper's Solved these Questions
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (NEWTONS LAWS OF MOTION)
46 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (FRICTION)
14 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Units, Dimensions & Measurements)
30 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
Similar Questions
Rain is falling vertically downward with velocity 4m/s. A man is moving horizontally with velocity 3m/s, the velocity of rain with respect to man is
View SolutionA shower of rain appears to fall vertically downwards with a velocity of 12 kmph on a person walking west wards with a velocity of 5 kmph. The actual velocity and direction of the rain are
View SolutionAn object is falling vertically downwards with velocity 10m/s.In terms ofˆiandˆj find the image velocity. M
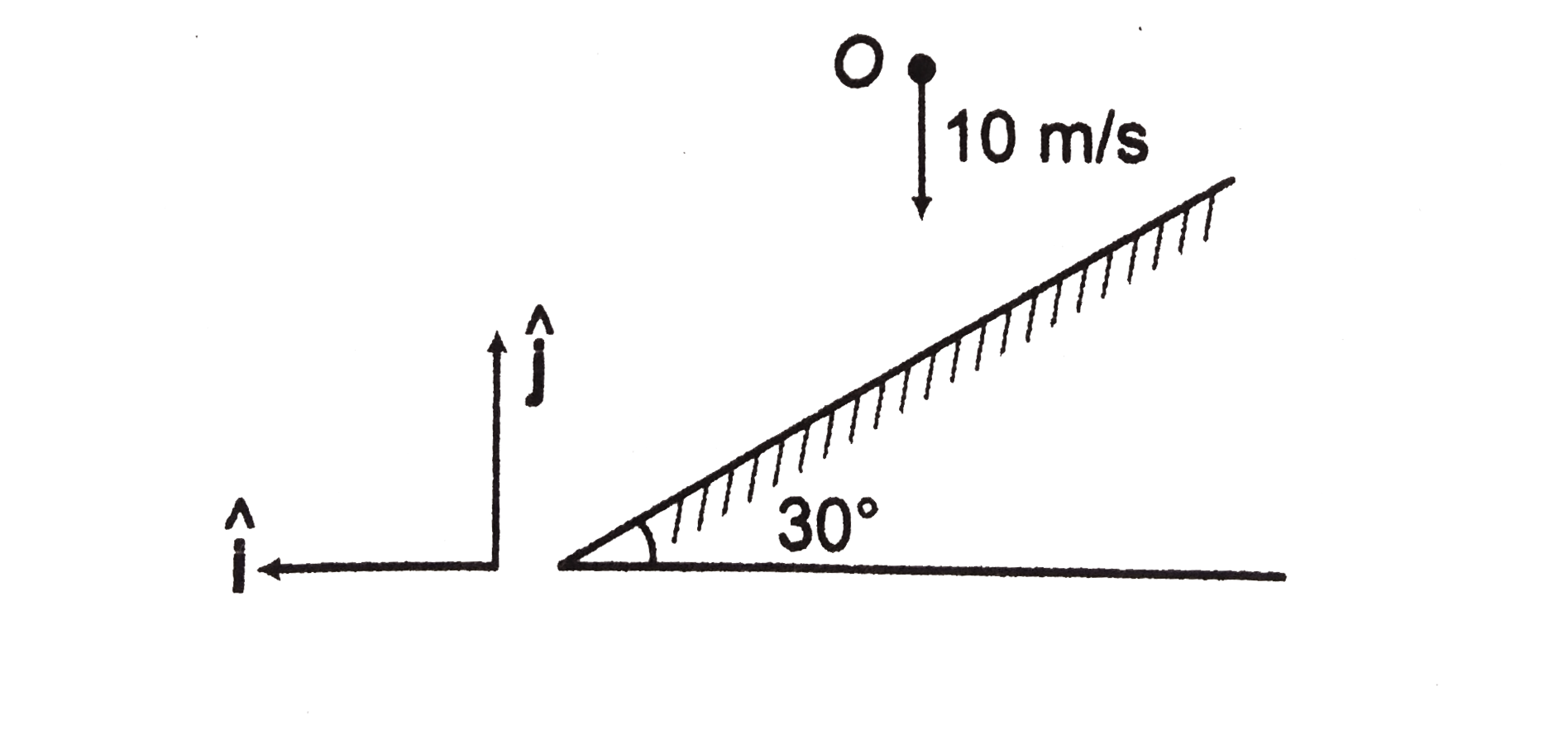
View SolutionRain is falling vertically with a velocity of 3kmh−1. A man walks in the rain with a velocity of 4kmh−1. The rain drops will fall on the man with a velocity of
View SolutionRain is falling vertically with a velocity of 3kmh−1. A man walks in the rain with a velocity of 4kmh−1. The rain drops will fall on the man with a velocity of
View SolutionRain is falling vertically downwards with a speed of 4kmh−1. A girl moves on a straight road with a velocity of 3kmh−1. The apparent velocity of rain with respect to the girl is.
View SolutionRain is falling vertically with a speed of 20ms−1., A person is running in the rain with a velocity of 5ms−1 and a wind is also blowing with a speed of 15ms−1 (both from the west) The angle with the vertical at which the person should hold his umbrella so that he may not get drenched is:
View SolutionRain droplets are falling in vertically downward direction with velocity 5m/s. A cyclist is moving in northward direction with velocity 10 m/s. The rain droplets will appear to the cyclist to be coming from
View SolutionAn observer is travelling in east with a velocity of 2 m/s and observes that wind is blowing in north with a velocity of 2m/s. If observer doubles his velocity then find out the velocity of wind appears to observer ?
View SolutionA small ball falling vertically downward with constant velocity 2m/s strikes elastically an inclined plane moving with velocity 2m/s as shown in figure. The velocity of rebound of the ball with respect ground is
(A)4m/s (B)2√5m/s (C)2√2m/s (D)2m/s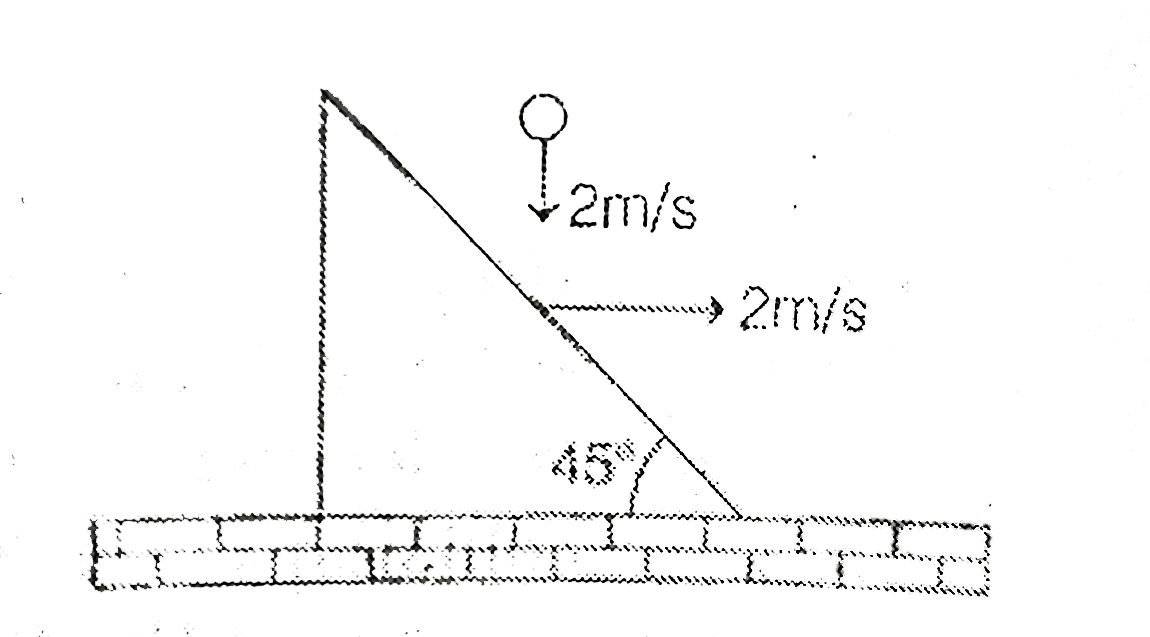
View Solution
