A ship is moving due east with a velocity of 12 m/ sec, a truck is moving across on the ship with velocity 4 m/sec. A monkey is climbing the vertical pole mounted on the truck with a velocity of 3m/sec. Find the velocity of the monkey as observed by the man on the shore
10 m/sec
15 m/sec
13 m/sec
20 m/sec
The correct Answer is:C
To find the velocity of the monkey as observed by the man on the shore, we need to consider the velocities of the ship, truck, and monkey in a three-dimensional coordinate system. Let's break down the problem step by step:
Step 1: Define the Directions and Velocities
- The ship is moving due east with a velocity of vx=12m/s.
- The truck is moving across the ship (let's assume this is in the north direction) with a velocity of vy=4m/s.
- The monkey is climbing the vertical pole on the truck with a velocity of vz=3m/s.
Step 2: Identify the Components of Velocity
We have the following components of the velocity:
- vx=12m/s (east direction)
- vy=4m/s (north direction)
- vz=3m/s (upward direction)
Step 3: Calculate the Resultant Velocity
To find the resultant velocity of the monkey as observed from the shore, we will use the Pythagorean theorem in three dimensions. The resultant velocity v can be calculated using the formula:
v=√v2x+v2y+v2z
Step 4: Substitute the Values
Now, substitute the values into the equation:
v=√(12)2+(4)2+(3)2
Calculating each term:
- (12)2=144
- (4)2=16
- (3)2=9
Step 5: Sum the Squares
Now, sum these values:
v=√144+16+9=√169
Step 6: Calculate the Square Root
Finally, calculate the square root:
v=13m/s
Conclusion
The velocity of the monkey as observed by the man on the shore is 13m/s.
---
- The ship is moving due east with a velocity of vx=12m/s.
- The truck is moving across the ship (let's assume this is in the north direction) with a velocity of vy=4m/s.
- The monkey is climbing the vertical pole on the truck with a velocity of vz=3m/s.
We have the following components of the velocity:
- vx=12m/s (east direction)
- vy=4m/s (north direction)
- vz=3m/s (upward direction)
To find the resultant velocity of the monkey as observed from the shore, we will use the Pythagorean theorem in three dimensions. The resultant velocity v can be calculated using the formula:
v=√v2x+v2y+v2z
Now, substitute the values into the equation:
v=√(12)2+(4)2+(3)2
Calculating each term:
- (12)2=144
- (4)2=16
- (3)2=9
Now, sum these values:
v=√144+16+9=√169
Finally, calculate the square root:
v=13m/s
The velocity of the monkey as observed by the man on the shore is 13m/s.
Topper's Solved these Questions
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - EXERCISE-3 (A boat in a river)
3 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - EXERCISE-3 (Oblique projection )
25 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - EXERCISE-3 (Motion in a plane)
5 Videos
- MAGNETISMBOOK - AAKASH SERIESCHAPTER - MAGNETISMEXERCISE - ADDITIONAL EXERCISE
22 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - QUESTION FOR DESCRIPTIVE ANSWER
7 Videos
Similar Questions
Anoop is moving due east with a velocity of 1m/s and Dhyani is moving due west with a velocity of 2m/s. what is the velocity of Anoop with respect to Dhyani?
View SolutionA ship is sailing towards the north at a speed of 1.25 m/s. The current is taking it towards the east at the rate of 1 m/s and a sailor is climbing a vertical pole on the ship at the rate of 0.5 m/s. Find the velocity of the sailor in space.
View SolutionA ship is sailing towards the north at a speed of 1.25 m/s. The current is taking it towards the east at the rate of 1 m/s and a sailor is climbing a vertical pole on the ship at the rate of 0.5 m/s. Find the velocity of the sailor in space.
View SolutionA bus is moving rightward with a velocity of 15m/sec and on the bus a monkey is renning oppositely with a velocity of 5m/sec( with respect to the bus ). Nearby a helicopter is rising vertically up with a velocity of 10m/sec.

Find out the direction of the bus as seen by the helicopter's pilot.
View SolutionA bus is moving rightward with a velocity of 15m/sec and on the bus a monkey is renning oppositely with a velocity of 5m/sec( with respect to the bus ). Nearby a helicopter is rising vertically up with a velocity of 10m/sec.
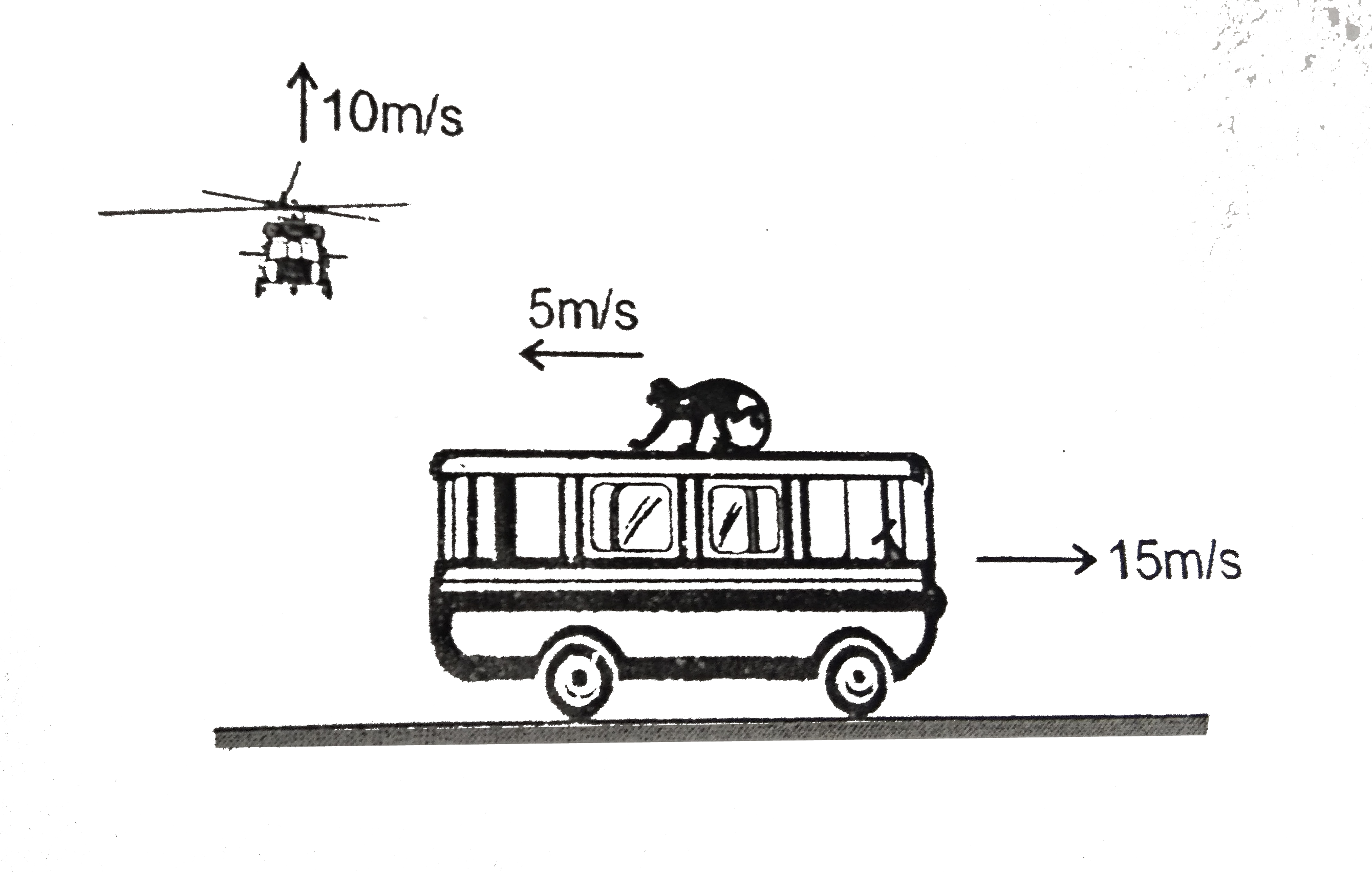
Find out the direction of the helicopter as seen by the monkey.
View SolutionA turck is moving on the ground with a velocity v=12m/s and a box is moving on the truck with respect to it with a velocity u=5m/s as shown in the figure. What is the velocity of the box with respect to the ground?

View SolutionRain is falling vertically downward with velocity 4m/s. A man is moving horizontally with velocity 3m/s, the velocity of rain with respect to man is
View SolutionAn observer is travelling in east with a velocity of 2 m/s and observes that wind is blowing in north with a velocity of 2m/s. If observer doubles his velocity then find out the velocity of wind appears to observer ?
View SolutionA man moves on a cycle with a velocity of 4 km/hr. The rain appears to fall on him with a velocity of 3 km/hr vertically. The actual velocity of the rain is
View SolutionA mass m moving horizontal (along the x-axis) with velocity v collides and sticks to mass of 3m moving vertically upward (along the y-axis) with velocity 2v. The final velocity of the combination is
View Solution
