(A) : The time of ascent for a body projected to move up a rough inclined plane is less than the time of descent.
(R ) : The retardation for upward motion is more than the acceleration for down motion on rough inclined plane.
Both (A) and (R) are true and (R) is the correct explanation of (A)
Both (A) and (R) are true and (R) is not the
(A) is true but ( R) is false
Both (A) and (R ) are false
The correct Answer is:A
To solve the question, we need to analyze the assertion and reason provided.
Assertion (A):
The time of ascent for a body projected to move up a rough inclined plane is less than the time of descent.
Reason (R):
The retardation for upward motion is more than the acceleration for downward motion on a rough inclined plane.
1. Understanding Forces on the Inclined Plane:
- When a body is projected upwards on a rough inclined plane, it experiences two main forces acting against its motion:
- The gravitational force component acting down the incline, which is mgsinθ.
- The frictional force acting down the incline, which is μmgcosθ (where μ is the coefficient of friction).
2. Calculating Retardation During Ascent:
- The total retarding force when moving upwards is:
Fretard=mgsinθ+μmgcosθ
- The retarding acceleration ar during ascent can be calculated as:
ar=gsinθ+μgcosθ
3. Time of Ascent:
- Using the kinematic equation for distance s=12at2, where s is the distance traveled (length of the incline L), we can express the time of ascent ta:
L=12art2a⟹ta=√2Lar=√2Lgsinθ+μgcosθ
4. Calculating Acceleration During Descent:
- When the body is moving downwards, the frictional force acts in the opposite direction:
Fnet=mgsinθ−μmgcosθ
- The acceleration ad during descent is:
ad=gsinθ−μgcosθ
5. Time of Descent:
- Similarly, the time of descent td can be expressed as:
L=12adt2d⟹td=√2Lad=√2Lgsinθ−μgcosθ
6. Comparing Times of Ascent and Descent:
- To compare ta and td, we observe that:
- The denominator for ta (which is gsinθ+μgcosθ) is greater than the denominator for td (which is gsinθ−μgcosθ).
- Since ta has a larger denominator, it follows that:
ta<td
- Therefore, the assertion (A) is true.
7. Verifying the Reason:
- The reason states that the retardation for upward motion is more than the acceleration for downward motion.
- We can see that:
ar=gsinθ+μgcosθ>gsinθ−μgcosθ=ad
- Thus, the reason (R) is also true.
Conclusion:
Both the assertion (A) and the reason (R) are true, and the reason correctly explains the assertion.
The time of ascent for a body projected to move up a rough inclined plane is less than the time of descent.
The retardation for upward motion is more than the acceleration for downward motion on a rough inclined plane.
- When a body is projected upwards on a rough inclined plane, it experiences two main forces acting against its motion:
- The gravitational force component acting down the incline, which is mgsinθ.
- The frictional force acting down the incline, which is μmgcosθ (where μ is the coefficient of friction).
- The total retarding force when moving upwards is:
Fretard=mgsinθ+μmgcosθ
- The retarding acceleration ar during ascent can be calculated as:
ar=gsinθ+μgcosθ
- Using the kinematic equation for distance s=12at2, where s is the distance traveled (length of the incline L), we can express the time of ascent ta:
L=12art2a⟹ta=√2Lar=√2Lgsinθ+μgcosθ
- When the body is moving downwards, the frictional force acts in the opposite direction:
Fnet=mgsinθ−μmgcosθ
- The acceleration ad during descent is:
ad=gsinθ−μgcosθ
- Similarly, the time of descent td can be expressed as:
L=12adt2d⟹td=√2Lad=√2Lgsinθ−μgcosθ
- To compare ta and td, we observe that:
- The denominator for ta (which is gsinθ+μgcosθ) is greater than the denominator for td (which is gsinθ−μgcosθ).
- Since ta has a larger denominator, it follows that:
ta<td
- Therefore, the assertion (A) is true.
- The reason states that the retardation for upward motion is more than the acceleration for downward motion.
- We can see that:
ar=gsinθ+μgcosθ>gsinθ−μgcosθ=ad
- Thus, the reason (R) is also true.
Both the assertion (A) and the reason (R) are true, and the reason correctly explains the assertion.
Similar Questions
Knowledge Check
The time of flight of projectile on an upward inclined plane depends upon.
Aangle of projectionBangle of inclination of the planeCair resistanceDa and b both
A block is projected upwards on a rough inclined plane at 20 m/s. let the time in going up, then is equal to
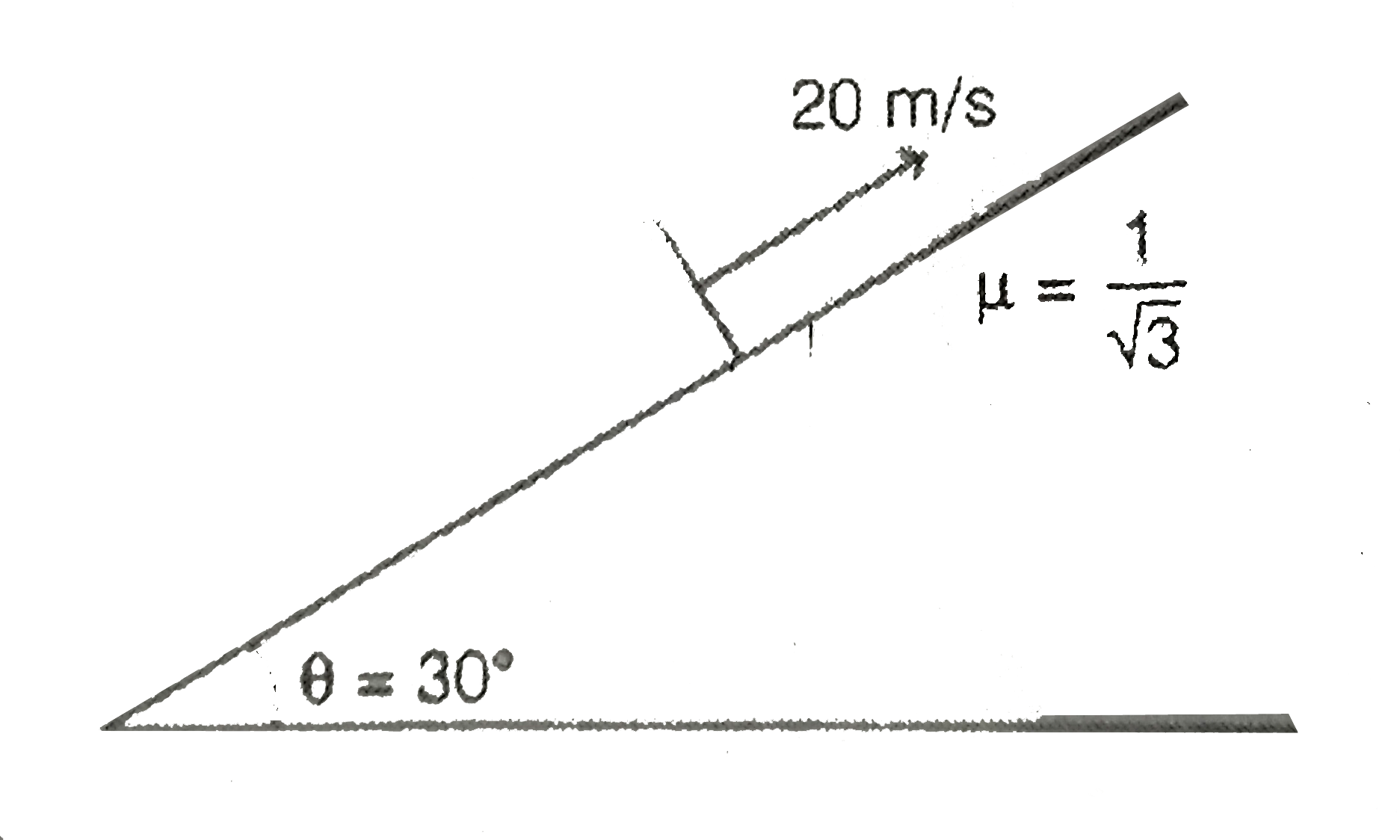
View SolutionA block is pushed up a rough inclined plane of 45°. If the time of descent is twice the time of ascent, the coefficient of friction is
View SolutionA block of mass m slides down a rough inclined plane with an acceleration g2

View SolutionThe force required to move a body up a rough inclined plane is double the force required to prevent the body from sliding down the plane. The coefficient of friction when the angle of inclination of the plane is 60∘ is .
View SolutionThe acceleration of a body rolling down on an inclined plane does not depend upon
View SolutionA hollow sphere and a solid sphere having same mass and same radii are rolled down a rough inclined plane.
View SolutionA : In the presence of air resistance, if the ball is thrown vertically upwards then time of ascent is less than the time of descent.
R : Force due to air friction always acts opposite to the motion of the body.
View Solution
