A block slides down a slope of angle with constant velocity. It is then projected up with a velocity of & = 30°. The maximum distance it can go up the plane before coming to stop is
10 m
5 m
4 mg
15 m
The correct Answer is:B
To solve the problem step by step, we will analyze the motion of the block on the slope and use the principles of physics to find the maximum distance it can travel up the slope before coming to a stop.
Step 1: Understand the Forces Acting on the Block
When the block slides down the slope with constant velocity, the net force acting on it is zero. This means that the force of gravity acting down the slope is balanced by the frictional force acting up the slope.
- The gravitational force component acting down the slope is given by:
Fgravity=mgsinθ
- The frictional force acting up the slope is given by:
Ffriction=μmgcosθ
- Since the block is moving with constant velocity, we have:
mgsinθ=μmgcosθ
Step 2: Find the Coefficient of Friction
From the equation mgsinθ=μmgcosθ, we can cancel mg (assuming m≠0):
sinθ=μcosθ
Thus, the coefficient of friction μ can be expressed as:
μ=tanθ
Step 3: Analyze the Block When Projected Upward
When the block is projected upward with an initial velocity v=10m/s, it will experience a deceleration due to gravity and friction. The total acceleration a when moving up the slope can be expressed as:
a=gsinθ+μgcosθ
Substituting μ:
a=gsinθ+tanθ⋅gcosθ
This simplifies to:
a=gsinθ+gsinθ=2gsinθ
Step 4: Calculate the Maximum Distance Up the Slope
Using the kinematic equation:
v2=u2+2as
where:
- v=0 (final velocity when the block comes to rest),
- u=10m/s (initial velocity),
- a=−2gsinθ (deceleration),
- s is the distance traveled up the slope.
Rearranging gives:
0=(10)2+2(−2gsinθ)s
100=4gsinθ⋅s
Thus:
s=1004gsinθ
Step 5: Substitute Values
Given g=10m/s2 and θ=30∘ (where sin30∘=12):
s=1004⋅10⋅12=10020=5m
Conclusion
The maximum distance the block can travel up the slope before coming to a stop is:
5m
When the block slides down the slope with constant velocity, the net force acting on it is zero. This means that the force of gravity acting down the slope is balanced by the frictional force acting up the slope.
Fgravity=mgsinθ
- The frictional force acting up the slope is given by:
Ffriction=μmgcosθ
- Since the block is moving with constant velocity, we have:
mgsinθ=μmgcosθ
From the equation mgsinθ=μmgcosθ, we can cancel mg (assuming m≠0):
sinθ=μcosθ
Thus, the coefficient of friction μ can be expressed as:
μ=tanθ
When the block is projected upward with an initial velocity v=10m/s, it will experience a deceleration due to gravity and friction. The total acceleration a when moving up the slope can be expressed as:
a=gsinθ+μgcosθ
Substituting μ:
a=gsinθ+tanθ⋅gcosθ
This simplifies to:
a=gsinθ+gsinθ=2gsinθ
Using the kinematic equation:
v2=u2+2as
where:
- v=0 (final velocity when the block comes to rest),
- u=10m/s (initial velocity),
- a=−2gsinθ (deceleration),
- s is the distance traveled up the slope.
0=(10)2+2(−2gsinθ)s
100=4gsinθ⋅s
Thus:
s=1004gsinθ
Given g=10m/s2 and θ=30∘ (where sin30∘=12):
s=1004⋅10⋅12=10020=5m
The maximum distance the block can travel up the slope before coming to a stop is:
5m
Similar Questions
A block slides down a rough inclined plane of slope angle θ with a constnat velocity. It is then projected up the same plane with an intial velocity v the distance travelled by the block up the plane coming to rest is .
View SolutionA particle is projected at an angle θ=30∘ with the horizontal, with a velocity of 10ms−1. Then
View SolutionA body is projected at an angle 30° with a velocity 42 ms−1 Its maximum height is
View SolutionA block kept on a inclined plane slides down the plane with constant velocity when the slope angle of the plane is θ. It is then projected up with an initial velocity u. How far up the incline will it move before coming to rest ? Will it slide down again ?
View SolutionA ball is thrown vertically upwards with a velocity of 10 ms−1. It returns to the ground with a velocity of 9 ms−1. If g=9.8 ms−2 , then the maximum height attained by the ball is nearly (assume air resistance to be uniform)
View SolutionA body projected with velocity 30 ms−1 reaches its maximum height in 1.5 s. Its range is (g=10ms−2)
View SolutionA body is projected from the ground with a velocity v=(3ˆi+10ˆj)ms−1. The maximum height attained and the range of the body respectively are (given g=10ms−2)
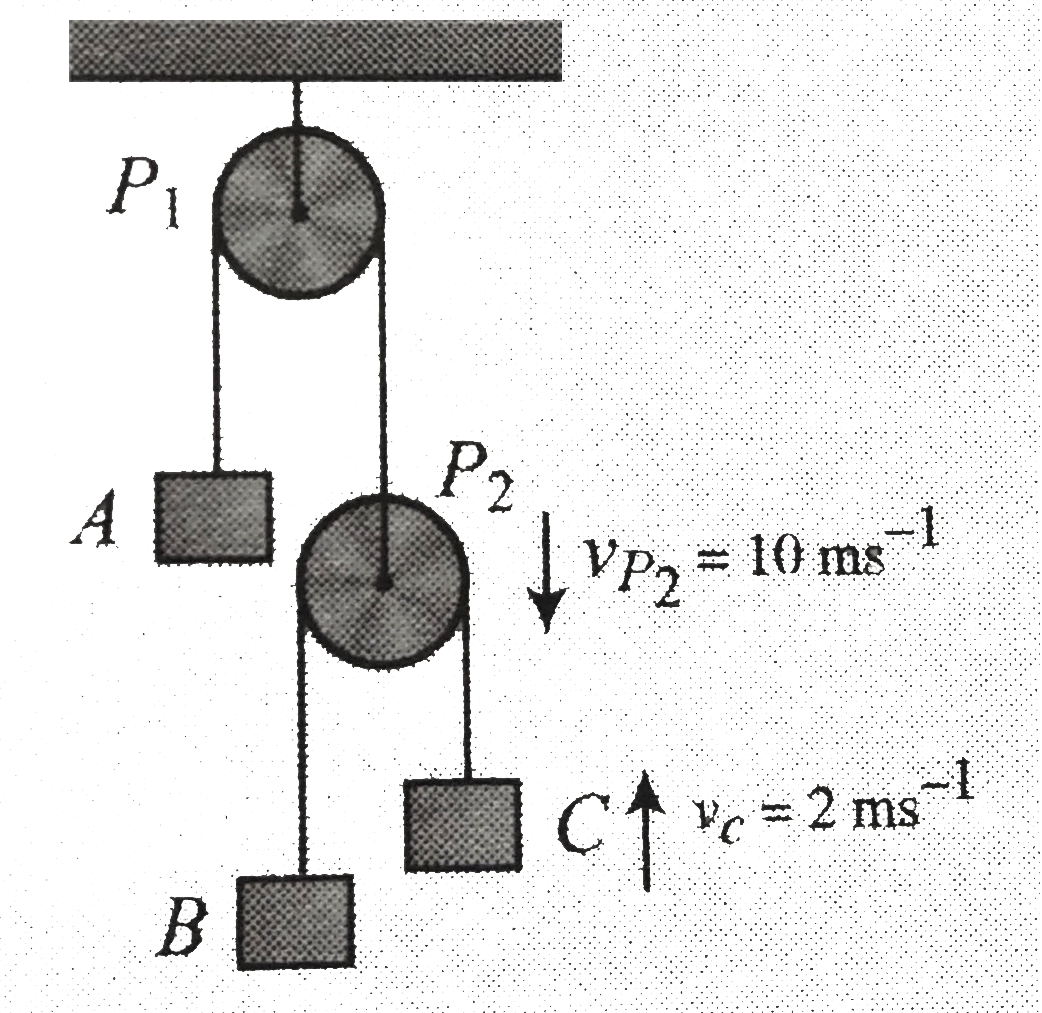
View SolutionThe three block shown in fig. move with constant velocities. Find the velocity of blocks A and B. given VP2=10ms−1⏐↓,VC=2ms−1↑⏐.
View SolutionA body is projected with a velocity →v=(3ˆi+4ˆj)ms−1 The maximum height attained by the body is: (g=10ms−2)
View SolutionAt what angle of elevation , should a projectile be projected with velocity with velocity 20ms−1 , so as to reach a maximum height of 10 m ?
View Solution
