The incidence of an occupation disease to the workers of a factory is found to be 1/5000. If there are 10000 workers in a factory then the probability that none of them will get the disease is
e
e−2
e−3
e4
The correct Answer is:B
To solve the problem step by step, we need to find the probability that none of the 10,000 workers in a factory will get an occupational disease, given that the incidence rate is 15000.
Step 1: Identify the given values
- The incidence rate of the disease p=15000
- The total number of workers n=10000
Step 2: Calculate the value of λ
The parameter λ for a Poisson distribution can be calculated using the formula:
λ=n×p
Substituting the known values:
λ=10000×15000=2
Step 3: Use the Poisson probability formula
The probability of observing x occurrences (in this case, the number of workers getting the disease) in a Poisson distribution is given by:
P(X=x)=e−λλxx!
We need to find the probability that none of the workers (i.e., x=0) will get the disease.
Step 4: Substitute x=0 into the formula
Substituting λ=2 and x=0:
P(X=0)=e−2⋅200!
Step 5: Simplify the expression
We know that:
- 20=1
- 0!=1
Thus, the equation simplifies to:
P(X=0)=e−2⋅11=e−2
Step 6: Conclusion
The probability that none of the 10,000 workers will get the disease is:
P(X=0)=e−2
Final Answer
The required probability is e−2.
---
- The incidence rate of the disease p=15000
- The total number of workers n=10000
The parameter λ for a Poisson distribution can be calculated using the formula:
λ=n×p
Substituting the known values:
λ=10000×15000=2
The probability of observing x occurrences (in this case, the number of workers getting the disease) in a Poisson distribution is given by:
P(X=x)=e−λλxx!
We need to find the probability that none of the workers (i.e., x=0) will get the disease.
Substituting λ=2 and x=0:
P(X=0)=e−2⋅200!
We know that:
- 20=1
- 0!=1
P(X=0)=e−2⋅11=e−2
The probability that none of the 10,000 workers will get the disease is:
P(X=0)=e−2
The required probability is e−2.
Similar Questions
find the mean salary of 60 workers of a factory from the given table
View SolutionThe following table gives the weekly wage of workers in a factory:
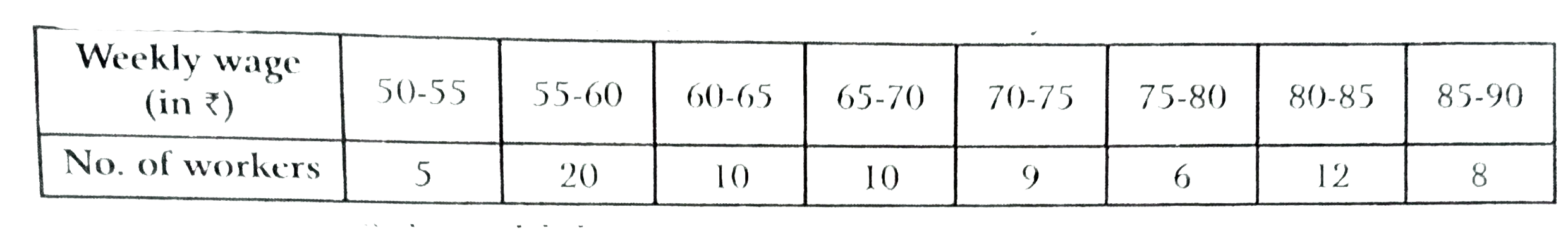
Find the mean
View SolutionFind the mean salary of 60 workers of a factory from the following table
View SolutionA recent survey found that the edges ages of workers in a factory as follow.
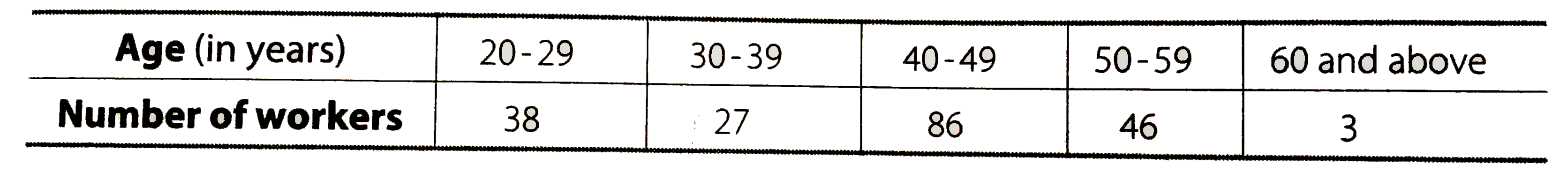
If a person is selected at random, find the probability that the person is
View SolutionRatio of the number of male and female workers in a factory is 5:3. If there are 115 male workers, determine the number of female workers in the factory.
View SolutionConsider the following distribution of daily wages of 50 workers of a factory.Find the mean daily wages of the workers of the factor}- by using an appropriate method.
View SolutionA man and a woman appear in an interview for two vacancies in the same post. The probability of man’s selection of 1/4 and that the woman’s selection is 1/3. What is the probability that none of them will be selected?
View SolutionA man and a woman appear in an interview for two vacancies in the same post. The probability of mans selection of 1/4 and that the womans selection is 1/3. What is the probability that none of them will be selected?
View SolutionA man and a woman appear in an interview for two vacancies in the same post. The probability of man’s selection of 1/4 and that the woman’s selection is 1/3. What is the probability that none of them will be selected?
View SolutionGiven below is distribution of daily earnings of 60 workers in a factory

Find 45th percentile.
View Solution
