From a frequency distribution, c = 3, l = 65 . 5 f = 42, m = 23, N = 102 then median is (l = lower limit of the median class, m= cumulative frequency of the class preceeding the median class, N = total frequency, f = frequency of the median class, c = width of the median class)
65.5
67.50
66.93
66.43
The correct Answer is:B
Step by step video & image solution for From a frequency distribution, c = 3, l = 65 . 5 f = 42, m = 23, N = 102 then median is (l = lower limit of the median class, m= cumulative frequency of the class preceeding the median class, N = total frequency, f = frequency of the median class, c = width of the median class) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
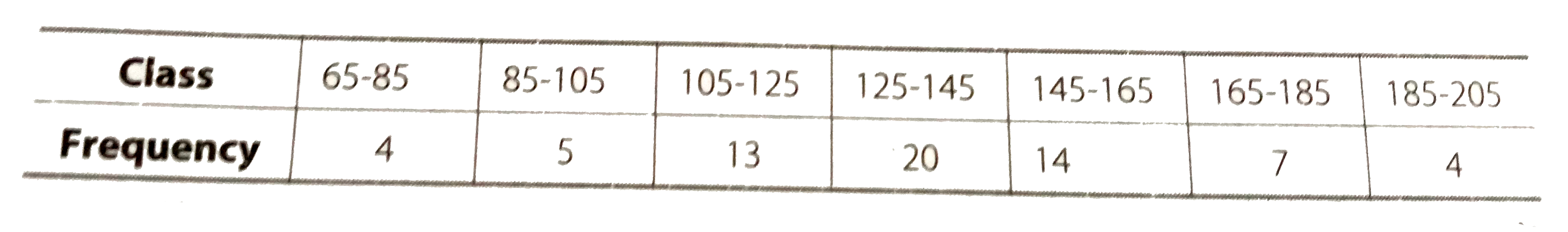
The cumulative frequency proceeding to the median class for the following data is
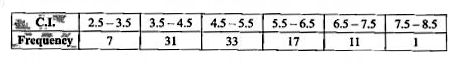 A71B88C38D40
A71B88C38D40
For the following distribution
the sum of lower limits of the median class and modal class is
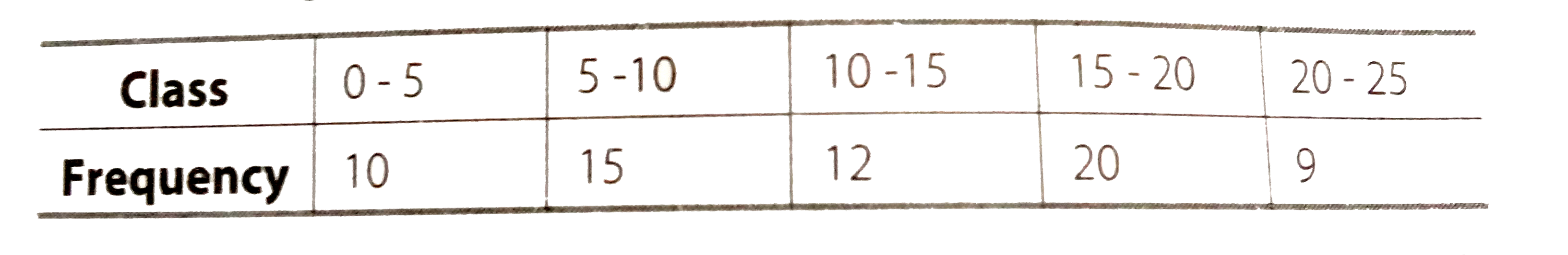
View SolutionConsider the following frequency distribution

The upper limit of the median class in
View SolutionIf m is the mid-point and l is the upper limit of a class in a continuous frequency distribution, then lower class limit of the class is
View SolutionWhile calculating mode the following observation are found. Lower limit of modal class =40, frequency of modal class =20, frequency of previous class =12 , frequency of preceding class =11 and width of the class =10, find mode .
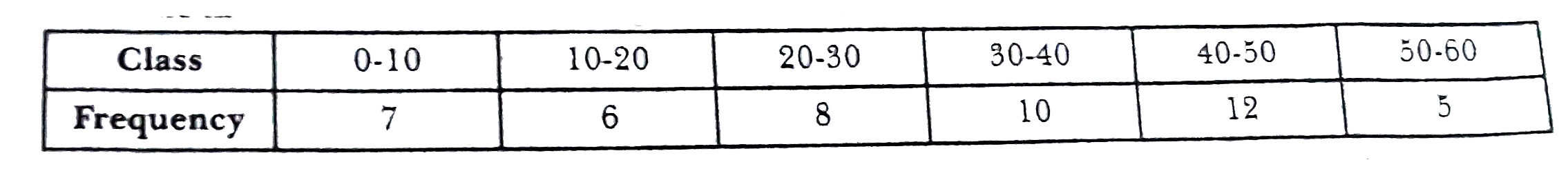
View SolutionFor the following frequency distribution

The upper limit of median class is
View SolutionWrite the median class of the following distribution:
View SolutionThe mean of the following distribution is 18. Find the frequency f of the class 19−21.
View Solution