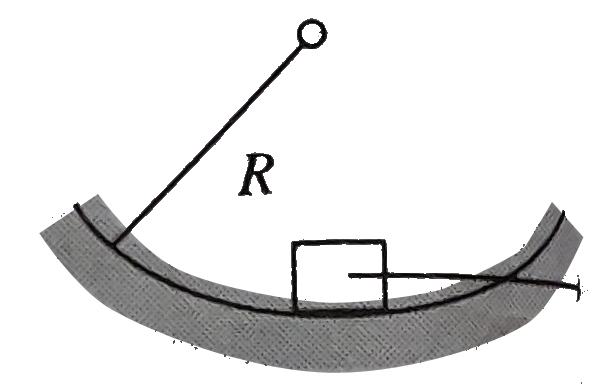
A particle is placed at rest inside a hollow hemisphere of radius . The coefficient of friction between the particle and the hemisphere is . The maximum height up to which the particle can remain stationary is
The correct Answer is:B
Step by step video & image solution for A particle is placed at rest inside a hollow hemisphere of radius R. The coefficient of friction between the particle and the hemisphere is mu = (1)/sqrt(3). The maximum height up to which the particle can remain stationary is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- LAWS OF MOTIONBOOK - AAKASH SERIESCHAPTER - LAWS OF MOTIONEXERCISE - PRACTICE EXERCISE
106 Videos
- LAWS OF MOTIONBOOK - AAKASH SERIESCHAPTER - LAWS OF MOTIONEXERCISE - EXERCISE - I
157 Videos
- GEOMETRICAL OPTICSBOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL-II PRACTICE SHEET (ADVANCED) INTEGER TYPE QUESTIONS)
10 Videos
- LOGIC GATESBOOK - AAKASH SERIESCHAPTER - LOGIC GATESEXERCISE - Exercise (Very Short Answer)
10 Videos
Similar Questions
If the coefficient of friction between an insect and bowl is μ and the radius of the bowl is r, find the maximum height to which the insect can crawl in the bowl.
View SolutionIf the coefficient of friction between an insect and bowl is μ and the radius of the bowl is r, find the maximum height to which the insect can crawl in the bowl.
View SolutionA rough horizontal plate rotates with angular velocity ω about a fixed vertical axis. A particle or mass m lies on the plate at a distance 5a4 from this axis. The coefficient of friction between the plate and the particle is 13 . The largest value of ω2 for which the particle will continue to be at rest on the revolving plate is
View SolutionIf the coefficient of friction between M and the inclined surfaces is μ=1/√3 find the minimum mass m of the rod so that the of mass M=10kg remain stationary on the inclined plane
View SolutionA particle is projected with a speed v0=√gR. The coefficient of friction the particle and the hemi- spherical plane is μ=0.5 Then , the acceleration of the partical is
View SolutionA car is taking turn on a circular path of radius R. If the coefficient of friction between the tyres and road is μ, the maximum velocity for no slipping is
View SolutionA particle of mass 0.1kg is launched at an angle of 53∘ with the horizontal . The particle enters a fixed rough hollow tube whose length is slightly less than 12.5m and which is inclined at an angle of 37∘ with the horizontal as shown in figure. It is known that the velocity of ball when it enters the tube is parallel to the axis of the tube. The coefficient of friction betweent the particle and tube inside the tube is μ=38[ Take g=10m/g2]

The velocity of the particle as it enters the tube is :
View SolutionA positively charged particle of mass m and charge q is projected on a rough horizontal x-y plane surface with z-axis in the vertically upward direction. Both electric and magnetic fields are acting in the region and given by →E=−E0ˆkand→B=−B0ˆk , respectively. The particle enters into the field at (a0,0,0) with velocity →v=v0ˆj. The particle starts moving in some curved path on the plane. If the coefficient of friction between the particle and the plane is μ. Then calculate the
(a) time when the particle will come to rest
(b) distance travelled by the particle when it comes to rest.

View SolutionA positively charged particle of mass m and charge q is projected on a rough horizontal x-y plane surface with z-axis in the vertically upward direction. Both electric and magnetic fields are acting in the region and given by →E=−E0ˆkand→B=−B0ˆk , respectively. The particle enters into the field at (a0,0,0) with velocity →v=v0ˆj. The particle starts moving in some curved path on the plane. If the coefficient of friction between the particle and the plane is μ. Then calculate the
(a) time when the particle will come to rest
(b) distance travelled by the particle when it comes to rest.

View SolutionA particle of mass 0.1kg is launched at an angle of 53∘ with the horizontal . The particle enters a fixed rough hollow tube whose length is slightly less than 12.5m and which is inclined at an angle of 37∘ with the horizontal as shown in figure. It is known that the velocity of ball when it enters the tube is parallel to the axis of the tube. The coefficient of friction betweent the particle and tube inside the tube is μ=38[ Take g=10m/g2]

The distance from the point of projection where the particle will land on the horizontal plane after coming out from the tube is approximately equal to :
View Solution
