Question

A particle of mass $0.1kg$ is launched at an angle of ${53}^{\circ }$ with the horizontal . The particle enters a fixed rough hollow tube whose length is slightly less than $12.5m$ and which is inclined at an angle of ${37}^{\circ }$ with the horizontal as shown in figure. It is known that the velocity of ball when it enters the tube is parallel to the axis of the tube. The coefficient of friction betweent the particle and tube inside the tube is $\mu =\frac{3}{8}[$ Take $g=10m/g^2]$

The distance from the point of projection where the particle will land on the horizontal plane after coming out from the tube is approximately equal to $:$

Options

A. `8.4m`
B. `10m`
C. `18.4m`
D. `36.8m`

Answer

C

Explanation

Time taken by projectile to reach the bottom of tube from point of projection is

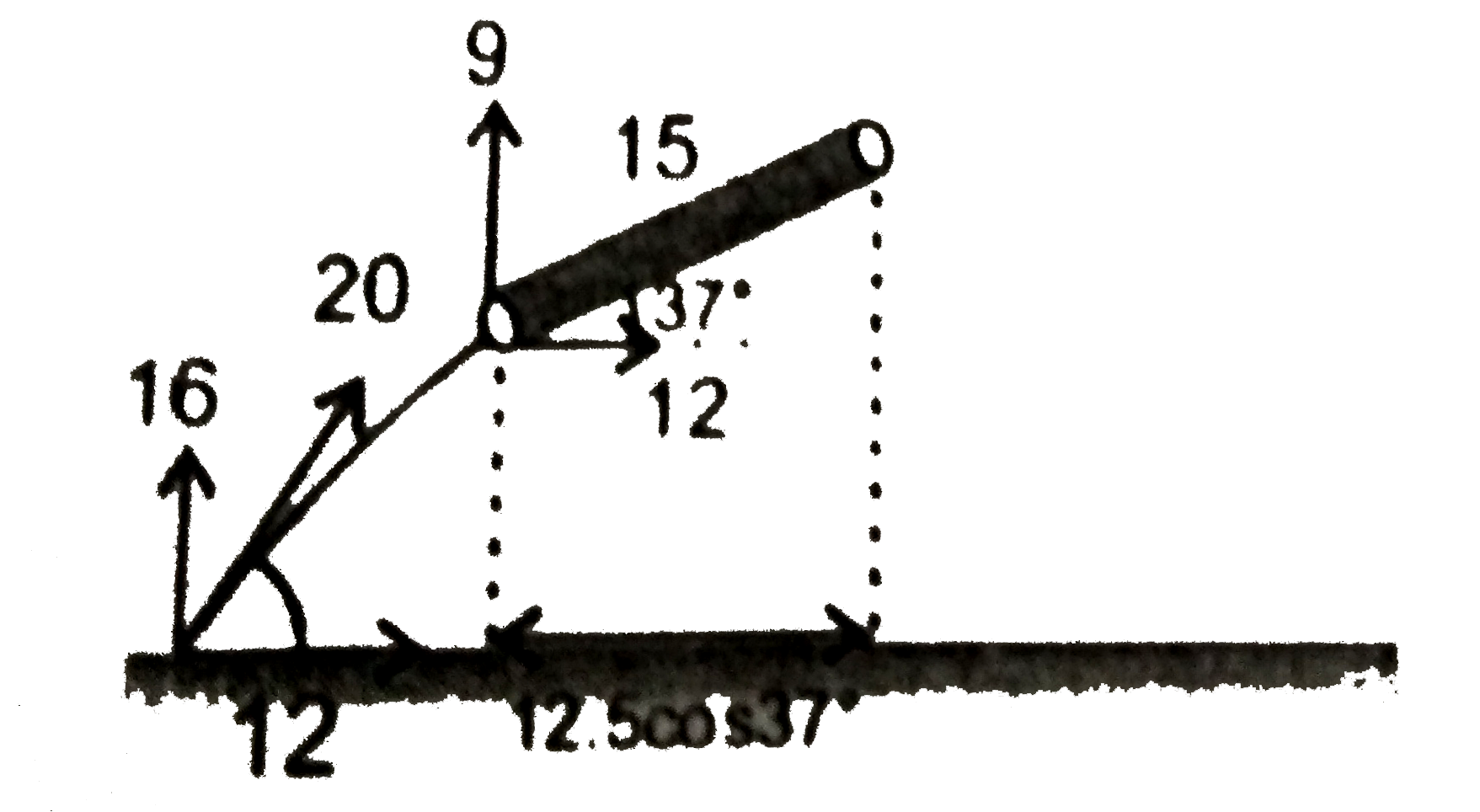
`9-16-g t :. T=(7)/(10)=0.7 sec. `
During this time particle travels `12xx0.7m`
`=8.4m ` horizontally.
`:. ` Take distance `=8.4m + 12.5 cos 37^(@)`
`=.4+10=18.4m.`