A car travelling with a speed 125 KMPH along a straight line comes to rest after travelling a distance 245 m.The time taken by the car to come to rest,in second is
11
12
16
14
The correct Answer is:D
To solve the problem step by step, we will follow the principles of motion in a straight line.
Step 1: Convert the speed from km/h to m/s
The initial speed of the car is given as 125 km/h. To convert this to meters per second (m/s), we use the conversion factor:
Speed in m/s=Speed in km/h×518
Calculating this:
Initial speed(U)=125×518=34.72m/s
Step 2: Identify the final speed
Since the car comes to rest, the final speed (V) is:
V=0m/s
Step 3: Use the first equation of motion
We can use the first equation of motion to find the acceleration (a):
V=U+at
Substituting the known values:
0=34.72+a⋅t
Rearranging gives:
a⋅t=−34.72(1)
Step 4: Use the third equation of motion
Next, we will use the third equation of motion to relate distance (s), initial speed (U), time (t), and acceleration (a):
s=Ut+12at2
Substituting the known values:
245=34.72⋅t+12at2(2)
Step 5: Substitute acceleration from equation (1) into equation (2)
From equation (1), we can express acceleration (a) in terms of time (t):
a=−34.72t
Substituting this into equation (2):
245=34.72⋅t+12(−34.72t)t2
This simplifies to:
245=34.72t−34.722t
245=34.72t−17.36t
245=17.36t
Step 6: Solve for time (t)
Now, we can solve for t:
t=24517.36≈14.11seconds
Final Answer
Thus, the time taken by the car to come to rest is approximately:
t≈14seconds
---
The initial speed of the car is given as 125 km/h. To convert this to meters per second (m/s), we use the conversion factor:
Since the car comes to rest, the final speed (V) is:
We can use the first equation of motion to find the acceleration (a):
Next, we will use the third equation of motion to relate distance (s), initial speed (U), time (t), and acceleration (a):
From equation (1), we can express acceleration (a) in terms of time (t):
Now, we can solve for t:
Thus, the time taken by the car to come to rest is approximately:
Similar Questions
A car accelerates from rest with 2m/s2 on a straight line path and then comes to rest after applying brakes. Total distance travelled by the car is 100 m in 20 seconds. Then, the maximum velocity attained by the car is
View SolutionA car 2 m long travelling at 30ms−1 overtakes another car 4 m long travelling at 20ms−1. The time taken by the first car to pass the second car is
View Solution
A block of mass m is suspended from one end of a light spring as shown. The origin O is considered at distance equal to natural length of the spring from the ceiling and vertical downwards direction as positive y-axis. When the system is in equilibrium a bullet of mass m3 moving in vertical up wards direction with velocity v0 strikes the block and embeds into it. As a result, the block (with bullet embedded into it) moves up and start oscillating. Based on the given information, answer the following question:
Q. The time taken by the block bullet system to move from y=mgk (initial equilibrium position) to y=0 (natural length of spring) is (A represents the amplitude of motion)View SolutionA bullet travelling with a velocity of 16 m/s penetrates a tree trunk and comes to rest in 0.4 m. Find the time taken during the retardation.
View SolutionThe variation of velocity of a particle with time moving along a straight line is illustrated in the following figure. The distance travelled by the particle in four seconds is.
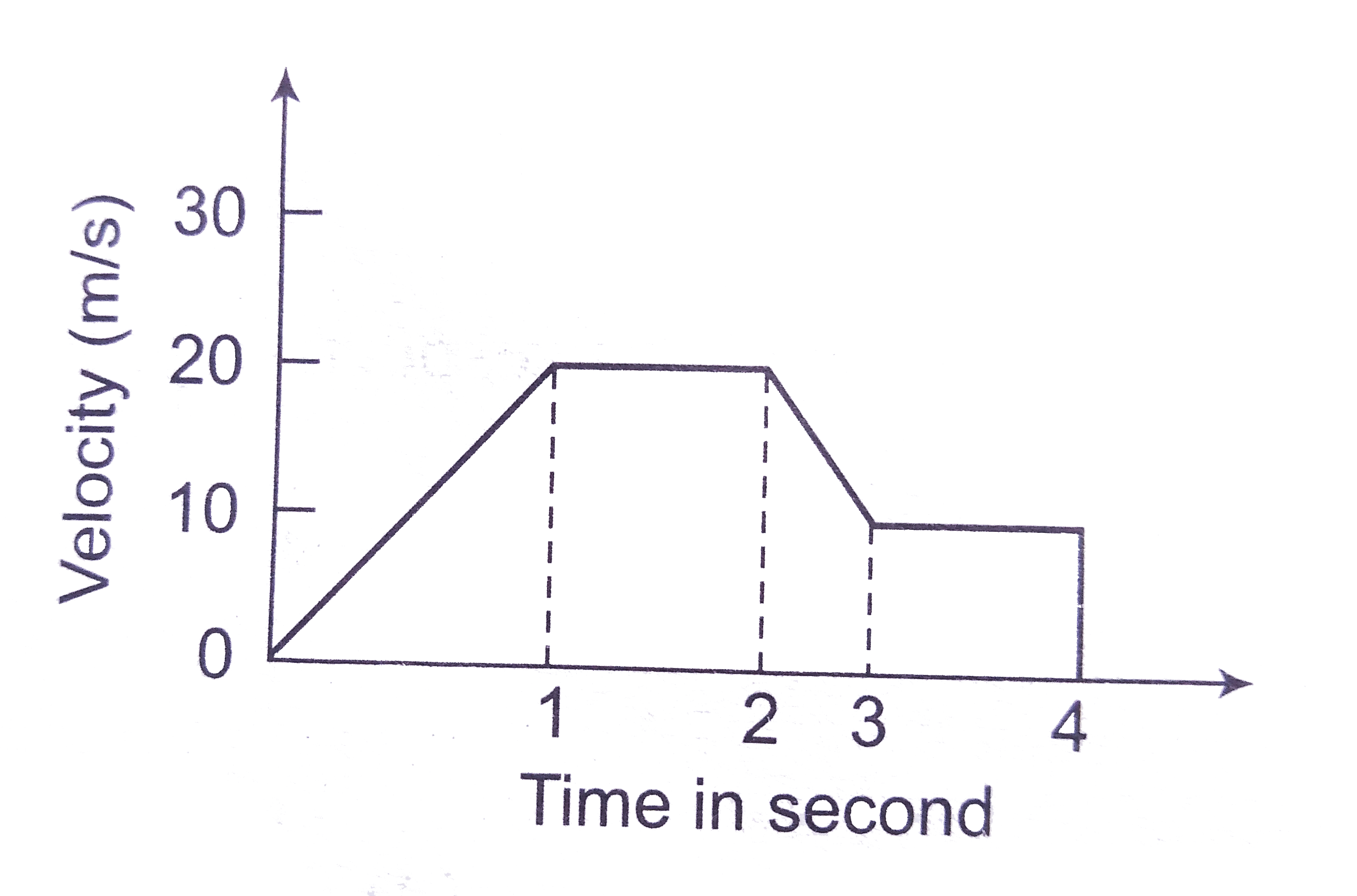 View Solution
View SolutionA particle starts with initial speed u and retardation a to come to rest in time T. The time taken to cover first half of the total path travelled is
View SolutionA particle moves with a simple harmonic motion in a straight line, in the first second starting from rest it travels a distance a and in the next second it travels a distance b in the same direction. The amplitude of the motion is
View SolutionThe distance by road between two towns A and B, is 216km , by rail it is 208 km. If car travels at a speed of x km/h, and train travel at a speed which is 16 km/h fastest than the car.Calculate (i) The time taken by the car, to reach town B from A, in terms of x.
View SolutionA particle start moving from rest state along a straight line under the action of a constant force and travel distance x in first 5 seconds. The distance travelled by it in next five seconds will be
View SolutionA car, starting from rest, has a constant acceleration for a time interval t1 during which it covers a distance In the next time interval the car has a constant retardation and comes to rest after covering a distance in time Which of the following relations is correct?
View Solution

