A car accelerates from rest with 2m/s2 on a straight line path and then comes to rest after applying brakes. Total distance travelled by the car is 100 m in 20 seconds. Then, the maximum velocity attained by the car is
10m/s
20 m/s
15 m/s
5 m/s
The correct Answer is:A
To solve the problem step by step, we will analyze the motion of the car, considering its acceleration and deceleration phases.
Step 1: Understand the motion phases
The car accelerates from rest with an acceleration of 2m/s2 and then comes to rest after applying brakes. The total distance traveled is 100m in 20s.
Step 2: Determine the time of acceleration
Let t1 be the time taken to accelerate and t2 be the time taken to decelerate. The total time is given by:
t1+t2=20s
Step 3: Calculate the distance during acceleration
The distance covered during the acceleration phase can be calculated using the formula:
d1=12at21
where a=2m/s2. Therefore,
d1=12⋅2⋅t21=t21
Step 4: Calculate the maximum velocity
The maximum velocity v0 attained at the end of the acceleration phase can be calculated using:
v0=at1=2t1
Step 5: Calculate the distance during deceleration
During the deceleration phase, the car comes to rest, and the distance covered can be expressed as:
d2=v202b
where b is the deceleration. Since the car comes to rest, we can express d2 in terms of t2 and v0:
d2=v0t2−12bt22
Step 6: Set up the equations
The total distance traveled is:
d1+d2=100m
Substituting the expressions for d1 and d2:
t21+v0t2−12bt22=100
Step 7: Substitute t2 in terms of t1
From t1+t2=20, we have:
t2=20−t1
Step 8: Substitute t2 into the distance equation
Now substituting t2 into the distance equation:
t21+v0(20−t1)−12b(20−t1)2=100
Step 9: Solve for v0
To find v0, we can also use the area under the velocity-time graph, which represents the total distance traveled. The area of the triangle formed during acceleration and the rectangle during deceleration can be calculated:
Area=12⋅t1⋅v0+v0⋅t2=100
Substituting t2=20−t1:
12⋅t1⋅v0+v0(20−t1)=100
Step 10: Solve for maximum velocity
From the previous steps, we can simplify and solve for v0:
1. The area under the curve gives:
12⋅t1⋅v0+v0(20−t1)=100
2. If we assume t1=10s (as a reasonable guess), then:
v0=2⋅10=20m/s
3. However, since the total distance is 100 m, we can find that the maximum velocity attained is:
v0=10m/s
Thus, the maximum velocity attained by the car is 10m/s.
The car accelerates from rest with an acceleration of 2m/s2 and then comes to rest after applying brakes. The total distance traveled is 100m in 20s.
Let t1 be the time taken to accelerate and t2 be the time taken to decelerate. The total time is given by:
t1+t2=20s
The distance covered during the acceleration phase can be calculated using the formula:
d1=12at21
where a=2m/s2. Therefore,
d1=12⋅2⋅t21=t21
The maximum velocity v0 attained at the end of the acceleration phase can be calculated using:
v0=at1=2t1
During the deceleration phase, the car comes to rest, and the distance covered can be expressed as:
d2=v202b
where b is the deceleration. Since the car comes to rest, we can express d2 in terms of t2 and v0:
d2=v0t2−12bt22
The total distance traveled is:
d1+d2=100m
Substituting the expressions for d1 and d2:
t21+v0t2−12bt22=100
From t1+t2=20, we have:
t2=20−t1
Now substituting t2 into the distance equation:
t21+v0(20−t1)−12b(20−t1)2=100
To find v0, we can also use the area under the velocity-time graph, which represents the total distance traveled. The area of the triangle formed during acceleration and the rectangle during deceleration can be calculated:
Area=12⋅t1⋅v0+v0⋅t2=100
Substituting t2=20−t1:
12⋅t1⋅v0+v0(20−t1)=100
From the previous steps, we can simplify and solve for v0:
1. The area under the curve gives:
12⋅t1⋅v0+v0(20−t1)=100
2. If we assume t1=10s (as a reasonable guess), then:
v0=2⋅10=20m/s
3. However, since the total distance is 100 m, we can find that the maximum velocity attained is:
v0=10m/s
Topper's Solved these Questions
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - PRACTICE EXERCISE
58 Videos
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - problems
47 Videos
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - EXERCISE -I
58 Videos
- MOTION IN A PLANEBOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - QUESTION FOR DESCRIPTIVE ANSWER
7 Videos
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH SERIESCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - EXERCISE-III
49 Videos
Similar Questions
A car accelerates from rest at a constant rate α for some time, after which it decelerates at a constant rate β, to come to rest. If the total time elapsed is t seconds. Then evalute the maximum velocity aquired by the car is
View SolutionA car travelling with a speed 125 KMPH along a straight line comes to rest after travelling a distance 245 m.The time taken by the car to come to rest,in second is
View SolutionA car acceleration from rest at a constant rate 2m/s2 for some time. Then, it retards at a constant rate of 4m/s2 and comes to rest. If it remains motion for 3 second, then the maximum speed attained by the car is:-
View SolutionA car accelerates from rest at a constant rate 'alpha' for some time after which it decelerates at a constant rate β to come to rest. If the total time elapsed is t, the maximum velocity acquired by the car is given by :
View SolutionA car accelerates from rest with 2ms−2 for 2s and then decelerates constantly with 4ms−2 for t0 second to come to rest. The graph for the motion is shown in figure.

a. Find the maximum speed attained by the car.
b. Find the value of t0.
View SolutionA car accelerates from rest at a constant rate α for some time, after which it decelerates at a constant rate β, to come to rest. If the total time elapsed is t seconds. Then evalute (a) the maximum velocity reached and (b) the total distance travelled.
View SolutionA car accelerates from rest at a constant rate α for some time, after which it decelerates at a constant rate β, to come to rest. If the total time elapsed is t seconds. Then evalute (a) the maximum velocity reached and (b) the total distance travelled.
View SolutionA car accelerates from rest at a constant rate α for some time, after which it decelerates at a constant rate β, to come to rest. If the total time elapsed is t seconds. Then evalute (a) the maximum velocity reached and (b) the total distance travelled.
View SolutionA train starts from rest and moves with a constant accelerationof 2.0ms2 for half a minute. The brakes are then applied and the train comes to rest in one minute. Find a. the total distance moved by the train, b. the maximum speed attained by the train and c. the position(s) of the train at half the maximum speed.
View SolutionThe speed of a car increases uniformly from zero to 10ms−1 in 2s and then remains constant (figure)
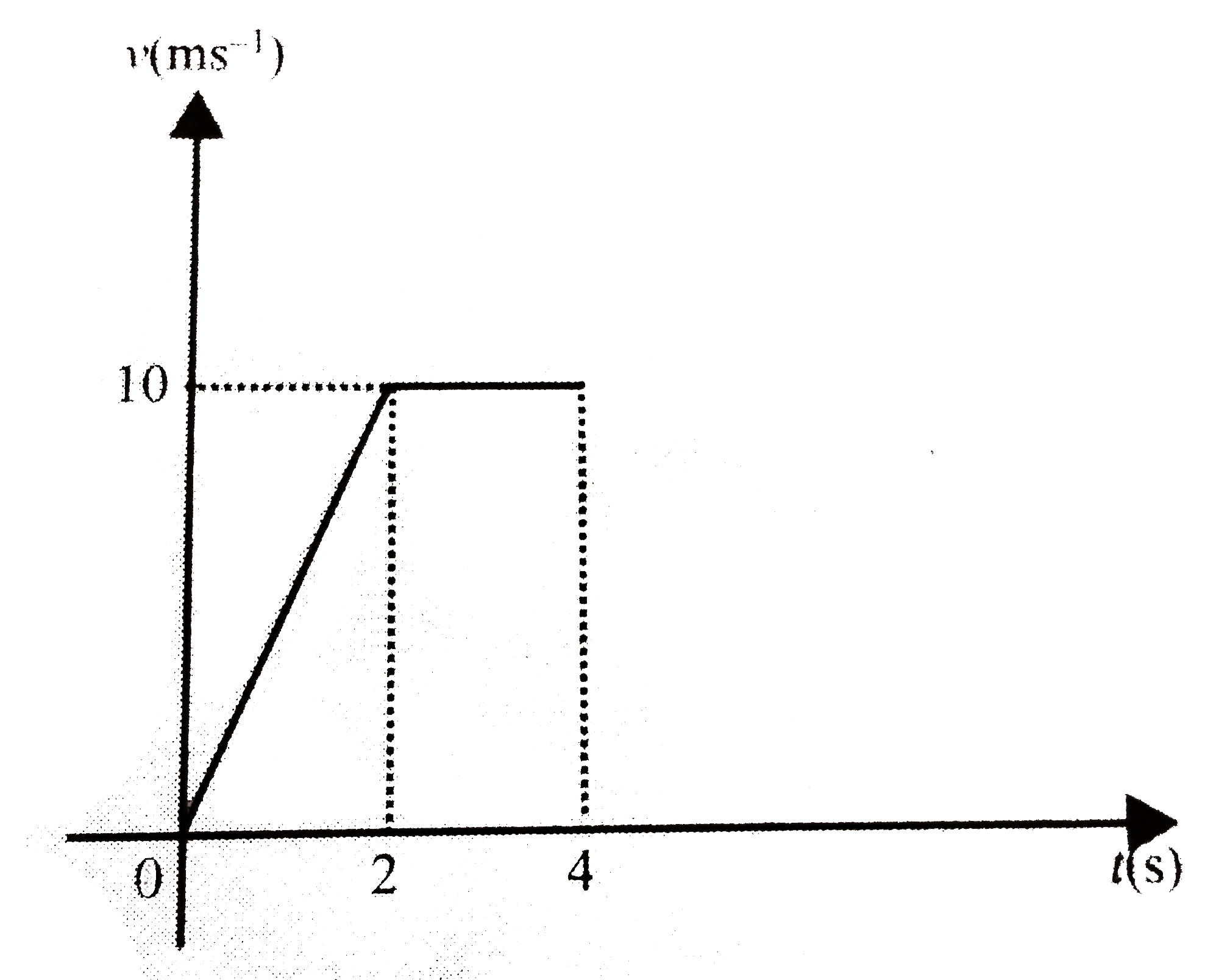
a. Find the distance travelled by the car in the first two seconds.
b. Find the distance travelled by the car in the next two seconds.
c. Find the total distance travelled in 4s.
View Solution
