The angle between the tangents to the circle with centre (4,5) drawn from P(-2,-3) is 120∘ then length of the tagent to the circle from P is
4
3
2
5
The correct Answer is:D
To find the length of the tangent to the circle from point P(-2, -3), we can follow these steps:
Step 1: Identify the center of the circle and the point P
The center of the circle is given as O(4, 5) and the point P is given as P(-2, -3).
Step 2: Calculate the distance OP
We will use the distance formula to find the distance between points O and P.
The distance formula is given by:
d=√(x2−x1)2+(y2−y1)2
Substituting the coordinates of O and P:
OP=√(4−(−2))2+(5−(−3))2
=√(4+2)2+(5+3)2
=√62+82
=√36+64
=√100
=10
Step 3: Use the angle between the tangents
Given that the angle between the tangents (∠BPA) is 120°, we can find the angle ∠AOP. The angle ∠AOP is half of ∠BPA:
∠AOP=12×120°=60°
Step 4: Apply the cosine rule in triangle AOP
In triangle AOP, we can use the cosine of angle AOP to find the length of the tangent AP. The cosine rule states:
cos(θ)=adjacenthypotenuse
Here, we have:
cos(60°)=APOP
We know that:
cos(60°)=12
Substituting the known values:
12=AP10
Step 5: Solve for AP
Multiplying both sides by 10:
AP=10×12=5
Conclusion
The length of the tangent from point P to the circle is 5.
The center of the circle is given as O(4, 5) and the point P is given as P(-2, -3).
We will use the distance formula to find the distance between points O and P.
d=√(x2−x1)2+(y2−y1)2
Substituting the coordinates of O and P:
OP=√(4−(−2))2+(5−(−3))2
=√(4+2)2+(5+3)2
=√62+82
=√36+64
=√100
=10
Given that the angle between the tangents (∠BPA) is 120°, we can find the angle ∠AOP. The angle ∠AOP is half of ∠BPA:
∠AOP=12×120°=60°
In triangle AOP, we can use the cosine of angle AOP to find the length of the tangent AP. The cosine rule states:
cos(θ)=adjacenthypotenuse
Here, we have:
cos(60°)=APOP
We know that:
cos(60°)=12
Substituting the known values:
12=AP10
Multiplying both sides by 10:
AP=10×12=5
The length of the tangent from point P to the circle is 5.
Similar Questions
The equation of a tangent to the circle with centre (2,−1) is 3x+y=0. Twice of the square of the length of the tangent to the circle from the point (23,17) is __________ .
View SolutionIf the angle between the two equal circles with centres (-2,0),(2,3) is 120∘ then the radius of the circle is
View SolutionIf the angle between two equal circles with centres (3, 10) (-5, 4) is 120∘ then the radius of the circles is
View SolutionShow that the angle between the tangents drawn from (-1,3) to the circle x2+y2=5 is 90∘.
View SolutionShow that if the length of the tangent from a point P to the circle x2+y2=a2 be four times the length of the tangent from it to the circle (x−a)2+y2=a2, then P lies on the circle 15x2+15y2−32ax+a2=0.
View SolutionAngle between tangents drawn from a points P to circle x2+y2−4x−8y+8=0 is 60∘ then length of chord of contact of P is
View SolutionFind the angle between the tangents
drawn from (3,2) to the circle
x2+y2−6x+4y−2=0
View SolutionA circle with centre 'P' is tangent to negative x and y axis and externally tangent to a circle with centre (-6,0) and radius = 2. what is the sum of all possible radii of the circle with centre P ?
View SolutionFind the length of a tangent drawn to a circle with radius 5 cm, from a point 13 cm from the centre of the circle.
View SolutionIn the given figure PA and PB are tangents to the circle drawn from an external point P. CD is a third tangent touching the circle at Q. If PB=10cm and CQ=2cm. What is the length of PC?
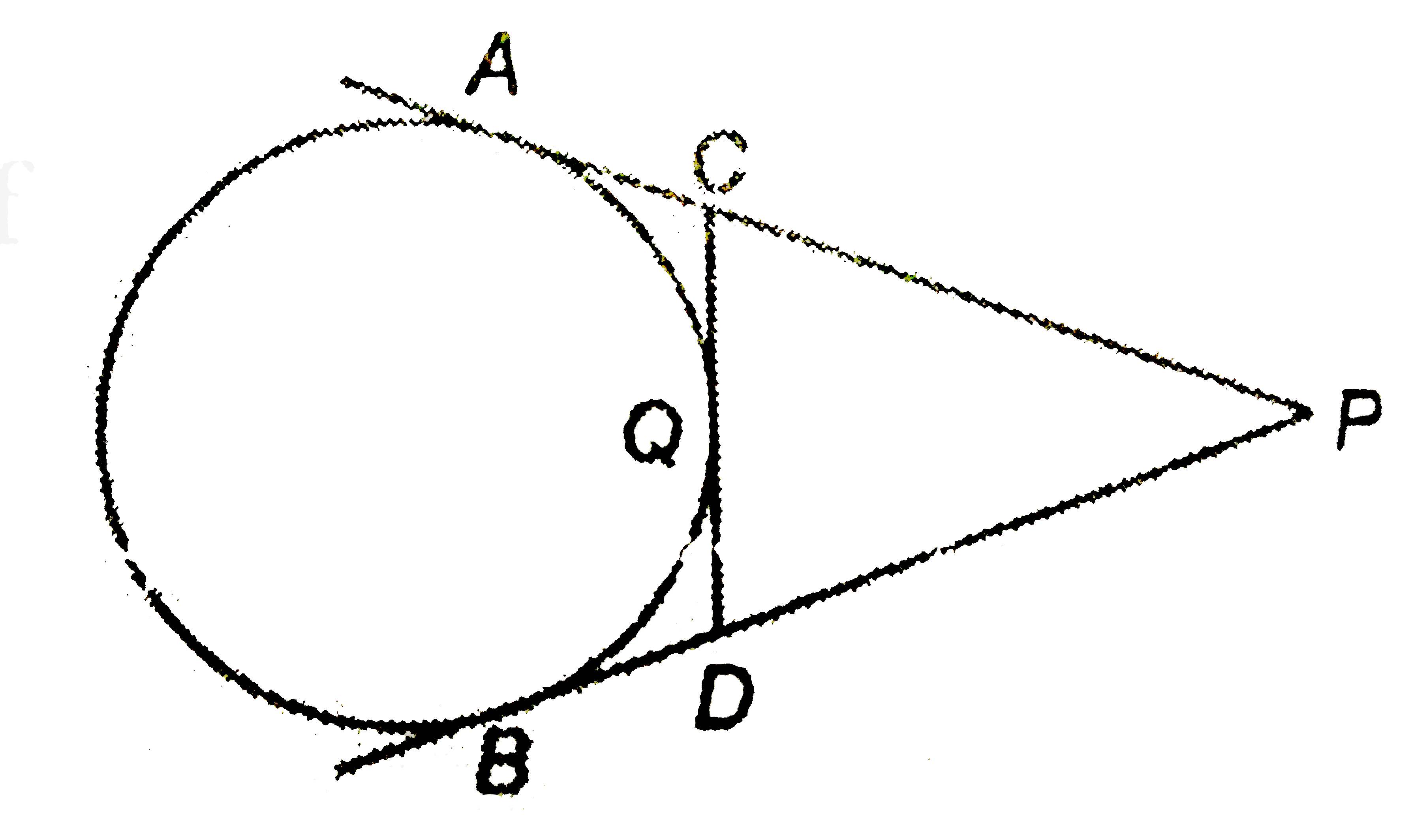
View Solution
