A circle with centre 'P' is tangent to negative x and y axis and externally tangent to a circle with centre (-6,0) and radius = 2. what is the sum of all possible radii of the circle with centre P ?
To solve the problem, we need to find the sum of all possible radii of a circle with center P that is tangent to the negative x-axis and negative y-axis, and also externally tangent to another circle with center (-6, 0) and radius 2.
1. Understanding the Circle with Center P:
- Since the circle is tangent to the negative x-axis and negative y-axis, the center P of the circle can be represented as (-R, -R), where R is the radius of the circle.
2. Position of the Other Circle:
- The other circle has its center at (-6, 0) and a radius of 2.
3. Distance Between Centers:
- The distance from the center of the circle with center P to the center of the other circle is given by the formula:
d=√((−6)−(−R))2+(0−(−R))2
- This simplifies to:
d=√(−6+R)2+R2
- Expanding this gives:
d=√(R2−12R+36)+R2=√2R2−12R+36
4. Condition for External Tangency:
- For the two circles to be externally tangent, the distance between their centers must equal the sum of their radii:
d=R+2
- Therefore, we set up the equation:
√2R2−12R+36=R+2
5. Squaring Both Sides:
- Squaring both sides to eliminate the square root gives:
2R2−12R+36=(R+2)2
- Expanding the right side:
2R2−12R+36=R2+4R+4
6. Rearranging the Equation:
- Bringing all terms to one side results in:
2R2−12R+36−R2−4R−4=0
- This simplifies to:
R2−16R+32=0
7. Using the Quadratic Formula:
- To find the roots of the quadratic equation R2−16R+32=0, we can use the quadratic formula:
R=−b±√b2−4ac2a
- Here, a=1, b=−16, and c=32:
R=16±√(−16)2−4⋅1⋅322⋅1
- Calculating the discriminant:
R=16±√256−1282=16±√1282=16±8√22=8±4√2
8. Finding the Sum of the Roots:
- The roots are 8+4√2 and 8−4√2. The sum of the roots can be calculated as:
(8+4√2)+(8−4√2)=16
Final Answer:
The sum of all possible radii of the circle with center P is 16.
- Since the circle is tangent to the negative x-axis and negative y-axis, the center P of the circle can be represented as (-R, -R), where R is the radius of the circle.
- The other circle has its center at (-6, 0) and a radius of 2.
- The distance from the center of the circle with center P to the center of the other circle is given by the formula:
d=√((−6)−(−R))2+(0−(−R))2
- This simplifies to:
d=√(−6+R)2+R2
- Expanding this gives:
d=√(R2−12R+36)+R2=√2R2−12R+36
- For the two circles to be externally tangent, the distance between their centers must equal the sum of their radii:
d=R+2
- Therefore, we set up the equation:
√2R2−12R+36=R+2
- Squaring both sides to eliminate the square root gives:
2R2−12R+36=(R+2)2
- Expanding the right side:
2R2−12R+36=R2+4R+4
- Bringing all terms to one side results in:
2R2−12R+36−R2−4R−4=0
- This simplifies to:
R2−16R+32=0
- To find the roots of the quadratic equation R2−16R+32=0, we can use the quadratic formula:
R=−b±√b2−4ac2a
- Here, a=1, b=−16, and c=32:
R=16±√(−16)2−4⋅1⋅322⋅1
- Calculating the discriminant:
R=16±√256−1282=16±√1282=16±8√22=8±4√2
- The roots are 8+4√2 and 8−4√2. The sum of the roots can be calculated as:
(8+4√2)+(8−4√2)=16
The sum of all possible radii of the circle with center P is 16.
Topper's Solved these Questions
Similar Questions
Knowledge Check
The equation of the circle with centre (6,0) and radius 6 is
Ax2+y2−12=0Bx2+y2+12=0Cx2+y2−12x=0Dx2+y2+12x=0In the xy-plane , a circle with center (6,0) is tangent to the line y=x. what is the radius of the circle ?
A2√6B2√3C3√2D3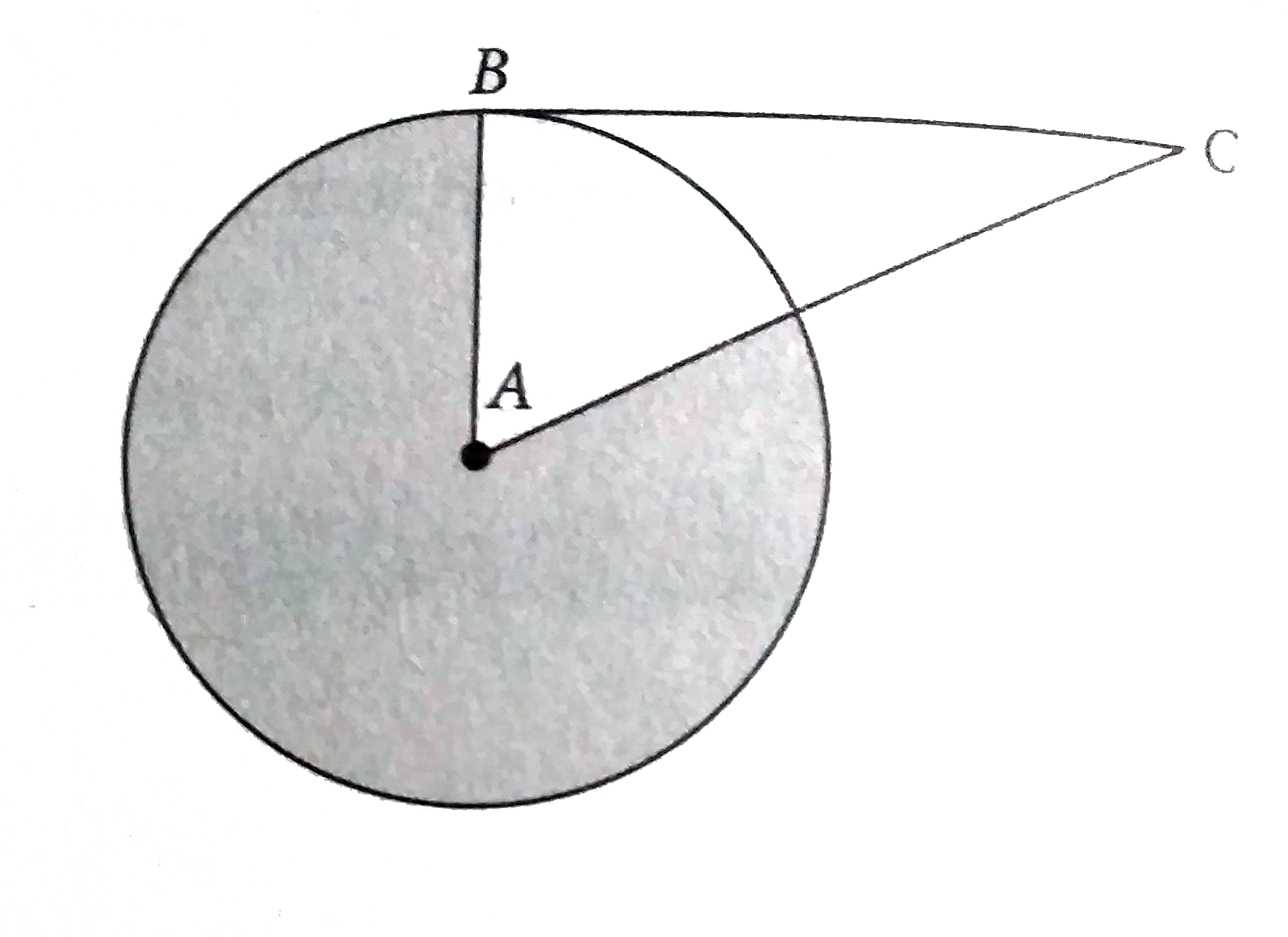
The circle above with centre A has an area of 21. BC is tangent to the circle with centre A at point B. IF AC=2AB, then what is the area of the shaded region?A3.5B15.75C17.5D21
A circle touches the X-axis and also touches externally the circle with centre (0,3) and radius 2. Show that the locus of the centre of circle is the parabola x2=10y−5.
View SolutionIf y=3x is a tangent to a circle with centre (1,1) then the other tangent drawn through (0,0) to the circle is
View SolutionThe length of tangent from an external point P on a circle with centre 0 is always less than OP.
View SolutionPA and PB are two tangents drawn from an external point P to a circle with centre C and radius=4cm If PA⊥PB then length of each tangent is
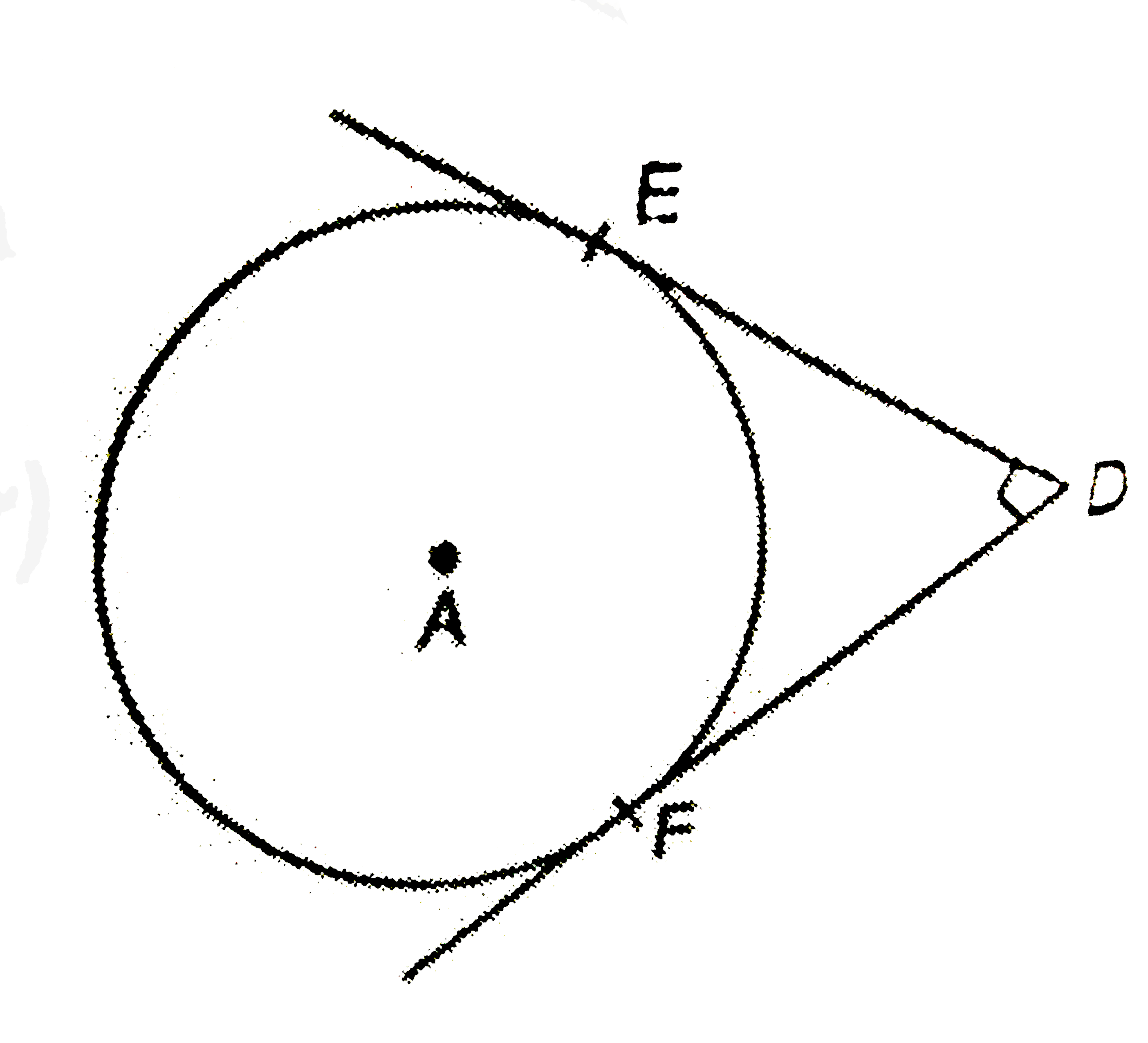
View SolutionIn figure, DE and DF are tangents from an external point D to a circle with centre A. If DE = 5 cm and DE⊥DF, then find the radius of the circle.
View Solution
