Question

If a square of side 10 is inscribed in a circle then radius of the circle is

Options

A. `10`
B. `5sqrt(2)`
C. `10sqrt(2)`
D. `5`

Answer

B

Explanation

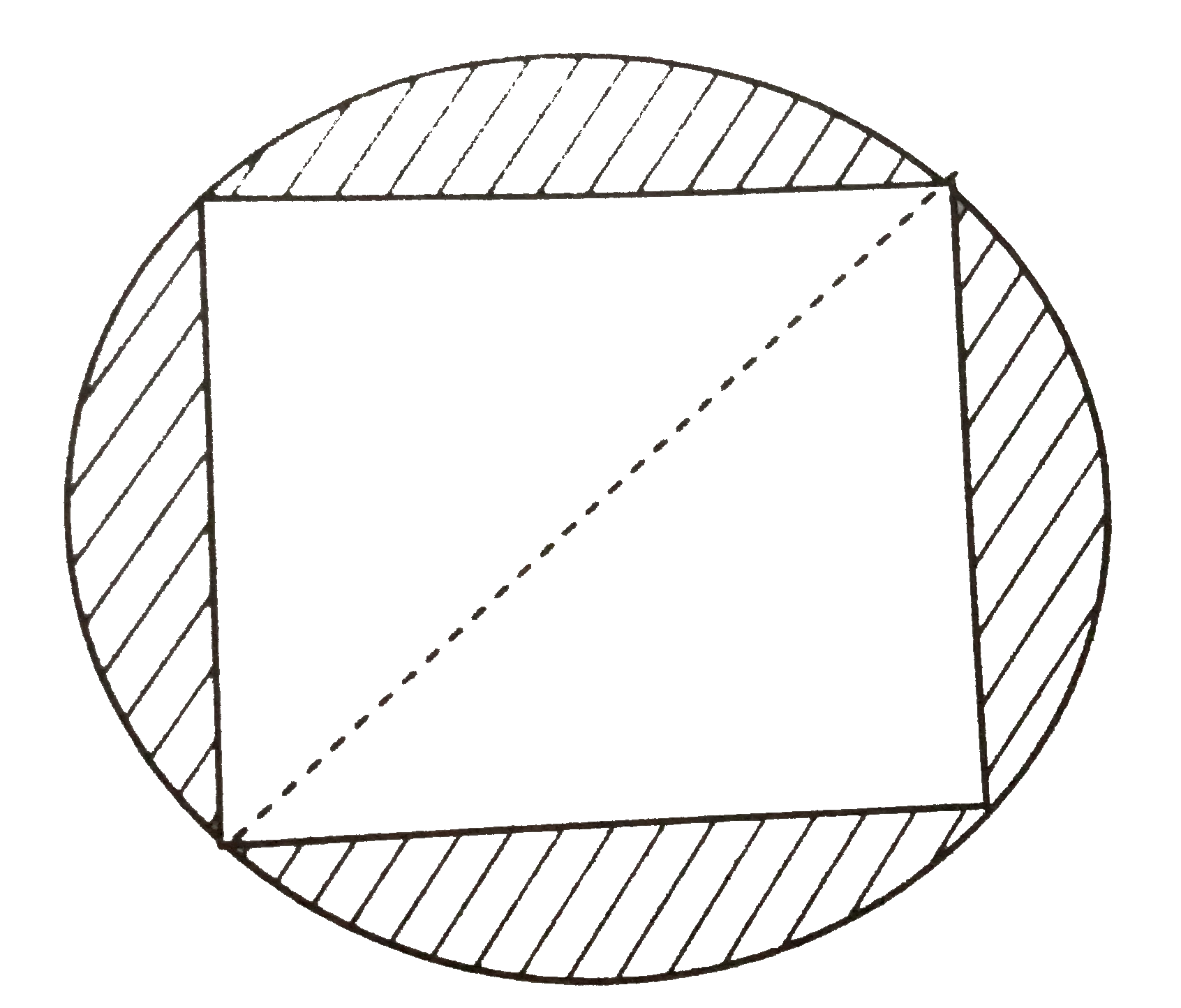
To find the radius of the circle in which a square of side 10 is inscribed, we can follow these steps:

Step 1: Understand the relationship between the square and the circle
When a square is inscribed in a circle, the diagonal of the square is equal to the diameter of the circle.

Step 2: Calculate the diagonal of the square
The formula for the diagonal \(d\) of a square with side length \(A\) is given by:
\( d = A \sqrt{2} \)
In this case, the side length \(A\) is 10. Therefore, the diagonal \(d\) is:
\( d = 10 \sqrt{2} \)

Step 3: Relate the diagonal to the diameter of the circle
Since the diagonal of the square is equal to the diameter \(D\) of the circle, we have:
\( D = d = 10 \sqrt{2} \)

Step 4: Find the radius of the circle
The radius \(R\) of the circle is half of the diameter:
\( R = \frac{D}{2} = \frac{10 \sqrt{2}}{2} = 5 \sqrt{2} \)

Conclusion
Thus, the radius of the circle is:
\( R = 5 \sqrt{2} \)