Acceleration of block parallel to plane (if M=2m,θ=45,μ=0.5)
5g9√2
5g18√2
5g3√2
5g8√2
The correct Answer is:A
To solve the problem of finding the acceleration of a block on an inclined plane, we can follow these steps:
Step 1: Understand the Problem
We have a block of mass M=2m on an inclined plane with an angle θ=45∘ and a coefficient of friction μ=0.5. We need to find the acceleration of the block parallel to the plane.
Step 2: Draw the Free Body Diagram
1. Draw the inclined plane and the block on it.
2. Identify the forces acting on the block:
- Weight acting downwards: W=2mg
- Normal force N acting perpendicular to the inclined plane
- Frictional force f acting parallel to the plane (upwards, opposing motion)
Step 3: Resolve Forces
1. Resolve the weight into components:
- Parallel to the plane: W∥=2mgsin(45∘)=2mg⋅1√2=2mg√2
- Perpendicular to the plane: W_{\perpendicular} = 2mg \cos(45^\circ) = 2mg \cdot \frac{1}{\sqrt{2}} = \frac{2mg}{\sqrt{2}}
Step 4: Calculate Normal Force
The normal force N is equal to the perpendicular component of the weight:
N = W_{\perpendicular} = \frac{2mg}{\sqrt{2}}
Step 5: Calculate Maximum Static Friction
The maximum static friction fs can be calculated using:
fs=μN=μ(2mg√2)=0.5⋅2mg√2=mg√2
Step 6: Calculate Net Force
The net force Fnet acting on the block parallel to the incline is given by:
Fnet=W∥−fs
Substituting the values:
Fnet=2mg√2−mg√2=mg√2
Step 7: Calculate Acceleration
Using Newton's second law, F=ma:
a=Fnetmass=mg√22m
This simplifies to:
a=g2√2
Final Answer
The acceleration of the block parallel to the plane is:
a=g2√2
---
We have a block of mass M=2m on an inclined plane with an angle θ=45∘ and a coefficient of friction μ=0.5. We need to find the acceleration of the block parallel to the plane.
1. Draw the inclined plane and the block on it.
2. Identify the forces acting on the block:
- Weight acting downwards: W=2mg
- Normal force N acting perpendicular to the inclined plane
- Frictional force f acting parallel to the plane (upwards, opposing motion)
1. Resolve the weight into components:
- Parallel to the plane: W∥=2mgsin(45∘)=2mg⋅1√2=2mg√2
- Perpendicular to the plane: W_{\perpendicular} = 2mg \cos(45^\circ) = 2mg \cdot \frac{1}{\sqrt{2}} = \frac{2mg}{\sqrt{2}}
The normal force N is equal to the perpendicular component of the weight:
N = W_{\perpendicular} = \frac{2mg}{\sqrt{2}}
The maximum static friction fs can be calculated using:
fs=μN=μ(2mg√2)=0.5⋅2mg√2=mg√2
The net force Fnet acting on the block parallel to the incline is given by:
Fnet=W∥−fs
Substituting the values:
Fnet=2mg√2−mg√2=mg√2
Using Newton's second law, F=ma:
a=Fnetmass=mg√22m
This simplifies to:
a=g2√2
The acceleration of the block parallel to the plane is:
a=g2√2
Similar Questions
Acceleration of block 'm' is (θ<45∘)
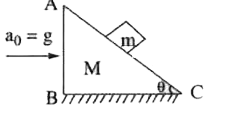
View SolutionA block of mass m is placed on a rough plane inclined at an angle θ with the horizontal. The coefficient of friction between the block and inclined plane is μ . Ifθ<tan−1(μ) , then net contact force exerted by the plane on the block is :
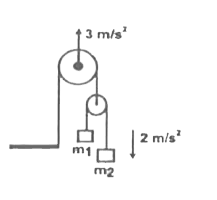
View SolutionIn the figure shown, the acceleration of block of mass m2 is 2m/s2. The acceleration of m1 :
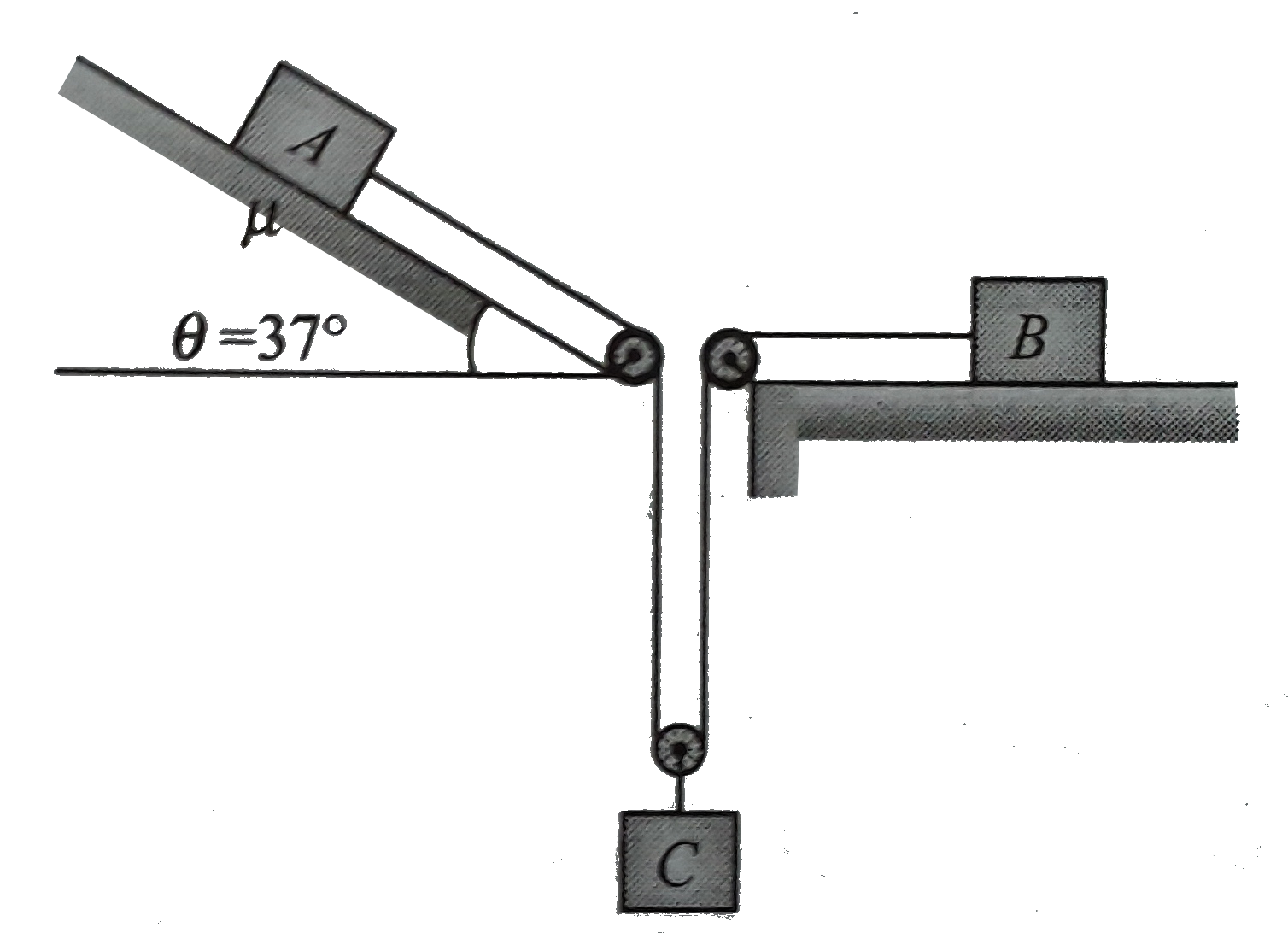
View SolutionIn the arrangement shown in figure pulleys are mass of block A,b and C is m1=5kgm2=4kg and m3=2.5kg respectively .Co-efficient acceleration of friction for both the plane is μ=0.50 Calculate acceleration of which block when system is released from rest
View SolutionA block B is pulled by a force of 18 N applied to a light pulley as shown in the figure. If the coefficient of friction is 0.4 and the acceleration of the block is 0.5 ms−2 , the mass of the block is (g=10m/s2)

View SolutionIf acceleration of block B is 4m/s2 upward & that of C is 6m/s2 downward. Find acceleration of A :−
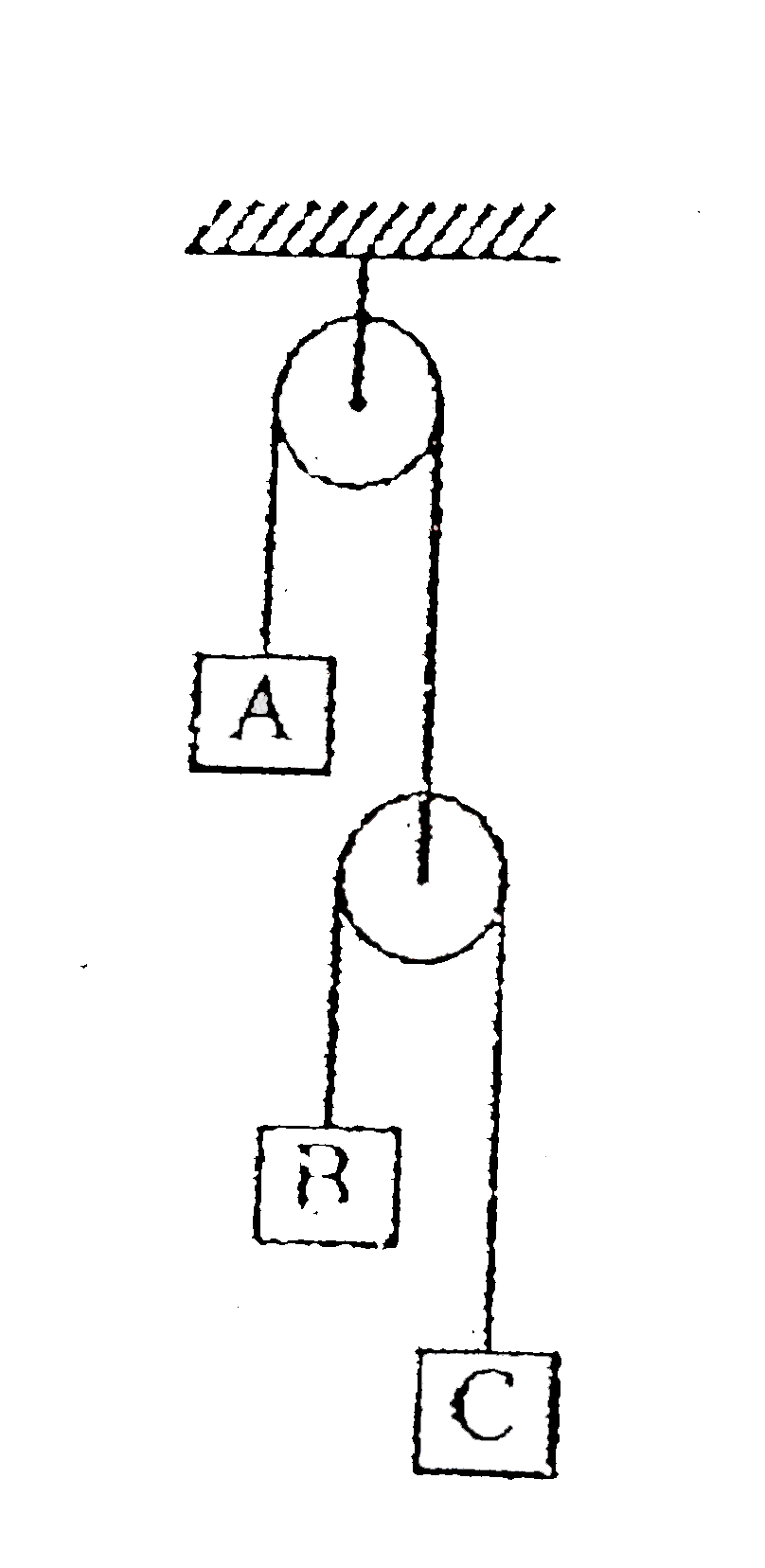
View SolutionIn fig. A is a 1kg block and B is a 0.5kg block. (a) Find the minimum weight of block C which must be placed on A to keep it from sliding , if μs between A and the table is 0.2. The block C is suddenly lifted off A. What is the acceleration of block A if μk between A and the table is 0.2 ?
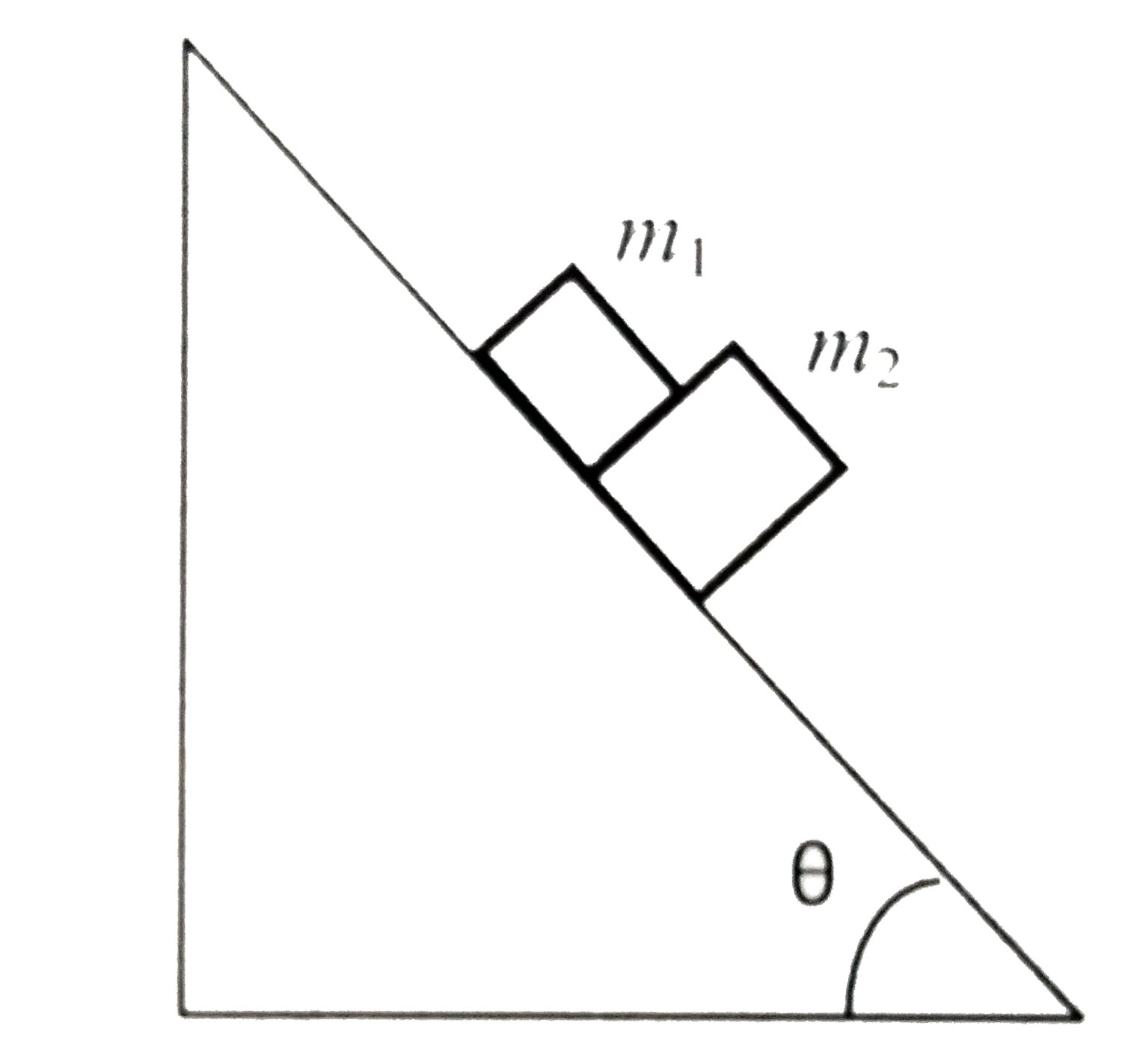
View SolutionA block of mass m1 = 1 kg another mass m2 = 2 kg, are placed together (see figure) on an inclined plane with angle of inclination θ. Various values of θ are given in List I. The coefficient of friction between the block m1 and the plane is always zero. The coefficient of static and dynamic friction between the block m2 and the plane are equal to μ=0.3 . In List II expressions for the friction on block m2 are given. Match the correct expression of the friction in List II with the angles given in List I, and choose the correct option. The acceleration due to gravity is denoted by g
[Useful information :tan(5.5∘)≈0.1,tan(11.5∘)≈0.2,tan(16.5∘)≈0.3].

View SolutionA block of mass m1 connected with another of mass m2 by a light spring of natural length l0 and stiiffness k is kept stationary on a rough horizontal surface . The coefficient of friction between m1 and surface is μ and the block m2 is smooth .The block m2 is moved with certain the block m1 in horizontal plane Find the (a) maximum to m1 and (b) acceleration of m2 in part(a)

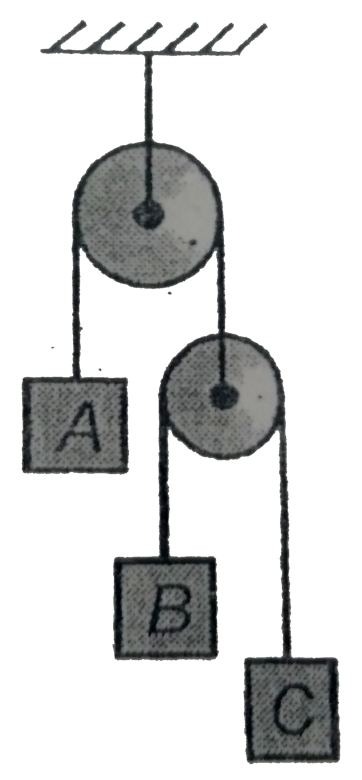
View SolutionAt a certain moment of time, acceleration of the block A is 2 m/s2 . Upward and acceleration of block B is 3 m/s2 upward. The acceleration of block C is ( masses of pulleys and string are negligible )
View Solution
