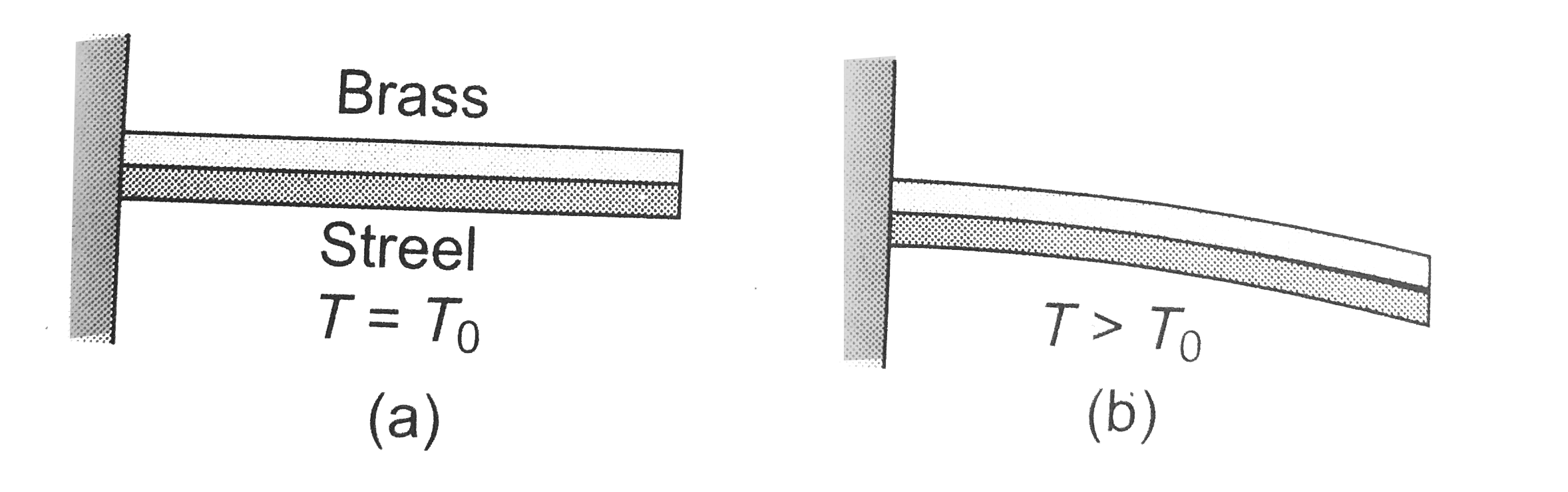
(A) : When a Bimetalic strip made up of Brass and Iron is heated then brass lies on the convex side.
( R) : Due to lower value of coefficient of linear expansion, brass aquires convex shape.
Both (A) and (R) are true and (R) is the correct explanation of (A)
Both (A) and (R) are true and (R) is not the correct explanation of (A)
(A) is true but (R) is false
Both (A) and (R) are false
The correct Answer is:C
To solve the question, we will analyze both the assertion and the reason provided.
1. Understanding the Assertion:
- The assertion states that when a bimetallic strip made of brass and iron is heated, the brass lies on the convex side.
- This means that upon heating, the strip bends such that the brass side is on the outer curve.
2. Understanding the Reason:
- The reason given is that brass acquires a convex shape due to having a lower coefficient of linear expansion.
- The coefficient of linear expansion indicates how much a material expands per degree of temperature increase.
3. Comparing Coefficients of Linear Expansion:
- It is known that brass has a higher coefficient of linear expansion compared to iron.
- This means that when both materials are heated, brass will expand more than iron.
4. Behavior of the Bimetallic Strip:
- When the bimetallic strip is heated, the brass (which expands more) will push outward compared to the iron (which expands less).
- This differential expansion causes the strip to bend, with brass on the outside (convex side) and iron on the inside (concave side).
5. Conclusion:
- The assertion is true: brass does lie on the convex side when heated.
- The reason is false: brass does not have a lower coefficient of linear expansion; it has a higher one compared to iron.
Final Answer:
- The assertion is true, and the reason is false.
- The assertion states that when a bimetallic strip made of brass and iron is heated, the brass lies on the convex side.
- This means that upon heating, the strip bends such that the brass side is on the outer curve.
- The reason given is that brass acquires a convex shape due to having a lower coefficient of linear expansion.
- The coefficient of linear expansion indicates how much a material expands per degree of temperature increase.
- It is known that brass has a higher coefficient of linear expansion compared to iron.
- This means that when both materials are heated, brass will expand more than iron.
- When the bimetallic strip is heated, the brass (which expands more) will push outward compared to the iron (which expands less).
- This differential expansion causes the strip to bend, with brass on the outside (convex side) and iron on the inside (concave side).
- The assertion is true: brass does lie on the convex side when heated.
- The reason is false: brass does not have a lower coefficient of linear expansion; it has a higher one compared to iron.
- The assertion is true, and the reason is false.
Similar Questions
Knowledge Check
At 50∘C , a brass rod has a length 50 cm and a diameter 2 mm . It is joined to a steel rod of the same length and diameter at the same temperature . The change in the length of the composite rod when it is heated to 250∘C is (Coefficient of linear expansion of brass = 2.0×10−5∘C−1 , coefficient of linear expansion of steel = 1.2×10−5∘C−1)
A0.28 cmB0.30 cmC0.32 cmD0.34 cm
What is a bimetallic strip? Why does a bimetallic strip of brass and iron bend on heating?
View SolutionThe apparent coefficient of expansion of a liquid when heated in a brass vessel is X and when heated in a tin vessel is Y. If α is the coefficient of linear expansion for brass, the coefficient of linear expansion of tin is
View SolutionA bimetallic strip is formed out of two identical strips one of copper and the other of brass. The coefficients of linear expansion of the strip goes up by ΔTand the strip bends to from an arc of radius of curvature R. Then R is.
View SolutionA bimetallic strip is formed out of two identical strips one of copper and the other of brass. The co-efficients of linear expansion of the two metals are αC and αB. On heating, the temperature of the strip goes up by △T the strip bends to form an are of radius of curvature R. Then R is
View SolutionConsider a brass rod and a steel rod (80 cm longer than brass rod) at 0∘C. It is observed that on increasing temperatures of the two rods by same amount difference in lengths of the two rods does not change. Given that the thermal coefficient of linear expansion for steel and brass are 11×10−6.∘C−1 and 19×10−6.∘C−1 respectively. The sum of lengths of the two rods at 0∘C is
View SolutionA brass rod of length 50cm and diameter 3.0cm is joined to a steel rod of the same length and diameter. What is the change in length of the combined rod at 250∘C, if the original length are at 40.0∘C?
(Coefficient of linear expansion of brass =2.0×10−5/∘C,steel=1.2×10−5/∘C
View SolutionA thin rod of negligible mass and area of cross section S is suspended vertically from one end. Length of the rod is L0 at T∘C . A mass m is attached to the lower end of the rod so that when temperature of the rod is reduced to 0∘C its length remains L0 Y is the Young’s modulus of the rod and α is coefficient of linear expansion of rod. Value of m is :
View Solution