A bimetallic strip is formed out of two identical strips one of copper and the other of brass. The coefficients of linear expansion of the strip goes up by ΔTand the strip bends to from an arc of radius of curvature R. Then R is.
Proportional to ΔT
inversely proportional to ΔT
proportional to |αB−αC|
inversely proportional to |αB−αC|
The correct Answer is:B, D
To solve the problem regarding the radius of curvature R of a bimetallic strip made of copper and brass when the temperature increases by ΔT, we will follow these steps:
Step 1: Understand the Linear Expansion
When the temperature of the bimetallic strip increases, both metals will expand. The linear expansion for each metal can be described by the formula:
L=L0(1+αΔT)
where L0 is the original length, α is the coefficient of linear expansion, and ΔT is the change in temperature.
Step 2: Set Up the Equations for Each Metal
For brass (with coefficient of linear expansion αB):
LB=L0(1+αBΔT)
For copper (with coefficient of linear expansion αC):
LC=L0(1+αCΔT)
Step 3: Relate Lengths to the Radius of Curvature
When the strip bends to form an arc, the lengths of the brass and copper strips can be expressed in terms of the radius of curvature R and the angle θ subtended at the center:
- Length of brass strip:
LB=Rθ+d
- Length of copper strip:
LC=Rθ
where d is the additional length due to the bending of the brass strip.
Step 4: Write the Length Equations
From the linear expansion equations, we have:
1. Rθ+d=L0(1+αBΔT) (for brass)
2. Rθ=L0(1+αCΔT) (for copper)
Step 5: Divide the Two Equations
Dividing the equation for brass by the equation for copper:
Rθ+dRθ=1+αBΔT1+αCΔT
Step 6: Simplify the Equation
This simplifies to:
1+dRθ=1+αBΔT1+αCΔT
Step 7: Use Binomial Approximation
Assuming αBΔT and αCΔT are small, we can use the binomial approximation:
1+αBΔT1+αCΔT≈1+(αB−αC)ΔT
Step 8: Rearranging the Equation
From the previous step, we can write:
dRθ=(αB−αC)ΔT
Thus,
d=Rθ(αB−αC)ΔT
Step 9: Solve for Radius of Curvature R
Rearranging gives:
R=dθ(αB−αC)ΔT
Conclusion
The radius of curvature R is inversely proportional to the difference in coefficients of linear expansion (αB−αC) and the temperature change ΔT.
Final Result
R∝1(αB−αC)ΔT
When the temperature of the bimetallic strip increases, both metals will expand. The linear expansion for each metal can be described by the formula:
L=L0(1+αΔT)
where L0 is the original length, α is the coefficient of linear expansion, and ΔT is the change in temperature.
For brass (with coefficient of linear expansion αB):
LB=L0(1+αBΔT)
For copper (with coefficient of linear expansion αC):
LC=L0(1+αCΔT)
When the strip bends to form an arc, the lengths of the brass and copper strips can be expressed in terms of the radius of curvature R and the angle θ subtended at the center:
- Length of brass strip:
LB=Rθ+d
- Length of copper strip:
LC=Rθ
where d is the additional length due to the bending of the brass strip.
From the linear expansion equations, we have:
1. Rθ+d=L0(1+αBΔT) (for brass)
2. Rθ=L0(1+αCΔT) (for copper)
Dividing the equation for brass by the equation for copper:
Rθ+dRθ=1+αBΔT1+αCΔT
This simplifies to:
1+dRθ=1+αBΔT1+αCΔT
Assuming αBΔT and αCΔT are small, we can use the binomial approximation:
1+αBΔT1+αCΔT≈1+(αB−αC)ΔT
From the previous step, we can write:
dRθ=(αB−αC)ΔT
Thus,
d=Rθ(αB−αC)ΔT
Rearranging gives:
R=dθ(αB−αC)ΔT
The radius of curvature R is inversely proportional to the difference in coefficients of linear expansion (αB−αC) and the temperature change ΔT.
R∝1(αB−αC)ΔT
Topper's Solved these Questions
- CALORIMETRY AND THERMAL EXPANSIONBOOK - RESONANCE ENGLISHCHAPTER - CALORIMETRY AND THERMAL EXPANSIONEXERCISE - Exercise-2
1 Videos
- CALORIMETRY AND THERMAL EXPANSIONBOOK - RESONANCE ENGLISHCHAPTER - CALORIMETRY AND THERMAL EXPANSIONEXERCISE - Exercie-3
1 Videos
- CALORIMETRY AND THERMAL EXPANSIONBOOK - RESONANCE ENGLISHCHAPTER - CALORIMETRY AND THERMAL EXPANSIONEXERCISE - Exercise-1
1 Videos
- CALORIMETRYBOOK - RESONANCE ENGLISHCHAPTER - CALORIMETRYEXERCISE - Exercise
19 Videos
- CAPACITORBOOK - RESONANCE ENGLISHCHAPTER - CAPACITOREXERCISE - Exercise
45 Videos
Similar Questions
A bimetallic strip is formed out of two identical strips one of copper and the other of brass. The temperature of the strip goes up by ΔTand the strip bends to from an arc of radius of curvature R. Then R is.
View SolutionA bimetallic strip is formed out of two identical strips one of copper and the other of brass. The co-efficients of linear expansion of the two metals are αC and αB. On heating, the temperature of the strip goes up by △T the strip bends to form an are of radius of curvature R. Then R is
View Solution
In figure which strip (brass or steel) has higher coefficient of linear expansion.
View SolutionIn figure which strip brass or steel have higher coefficient of linear expansion.
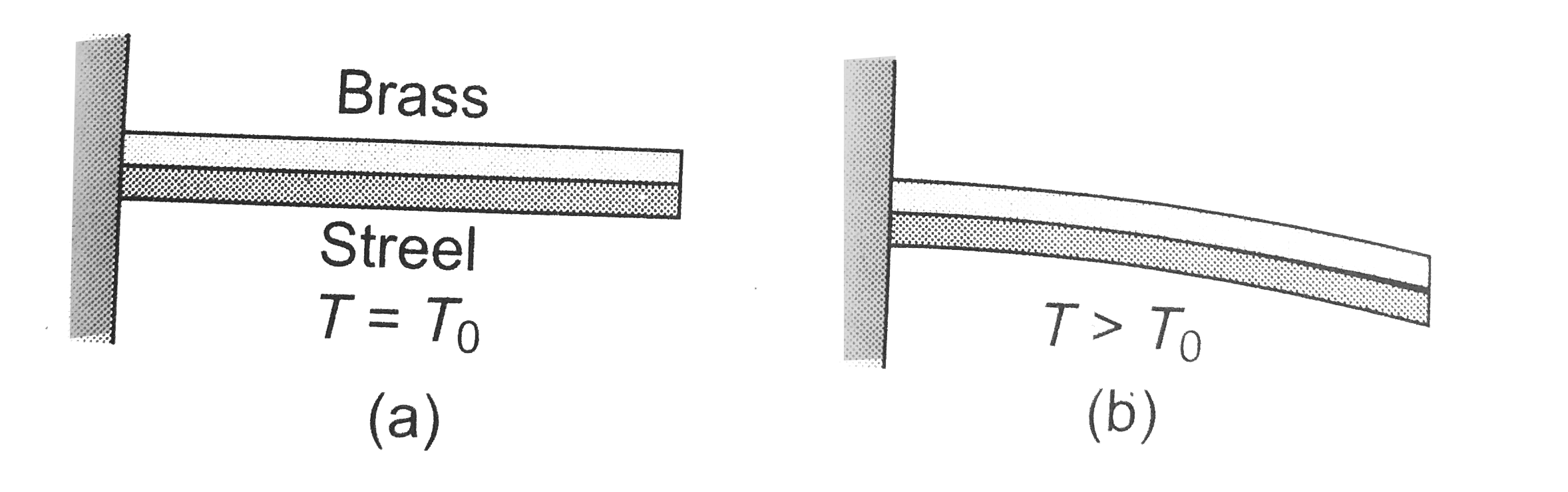
View SolutionTwo straight metallic strips each of thickness t and length l are rivetted together. Their coefficients of linear expansions areα1andα2. If they are heated through temperature ΔT, the bimetallic strip will bend to form an arc of radius
View SolutionA bimetallic strip consists of metals X and Y. It mounted rigidly at the base as shown. The metal X has a higher coefficient of expansion compared to that for metal Y. When the bimetallic strip is placed in a cold bath
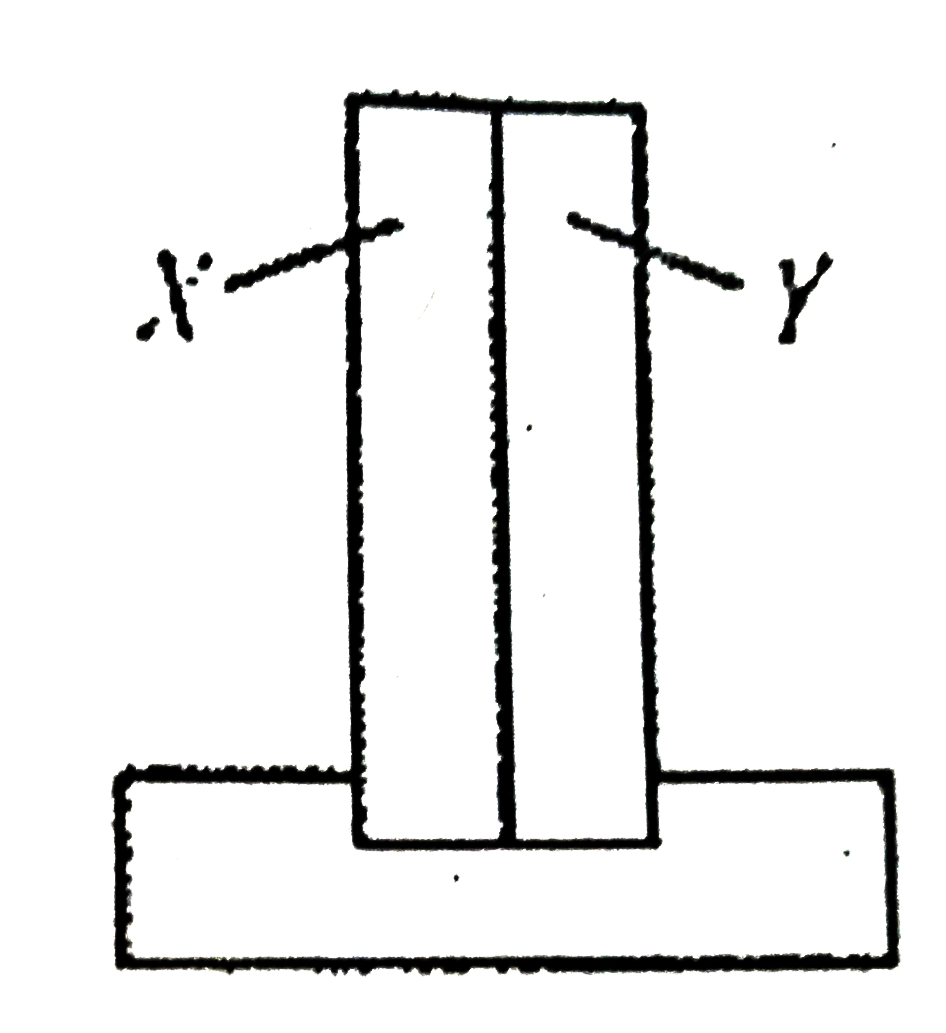
View SolutionA bimetallic strip consists of metals X and Y. It mounted rigidly at the base as shown. The metal X has a higher coefficient of expansion compared to that for metal Y. When the bimetallic strip is placed in a cold bath

View SolutionA wooden wheel of radius R is made of two semicircular part . The two parts are held together by a ring made of a metal strip of cross sectional area S and length L. L is slightly less than 2πR. To fit the ring on the wheel, it is heated so that its temperature rises by ΔTand it just steps over the wheel. As it cools down to surrounding temperature, it process the semicircle parts together. If the coefficient of linear expansion of the metal is α, and it Young's modulus is Y, the force that one part of the wheel applies on the other part is :
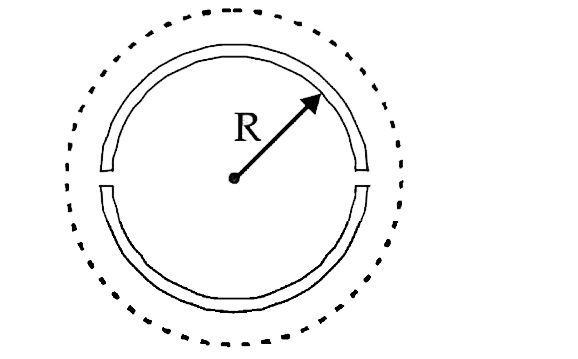
View Solution(A) : When a Bimetalic strip made up of Brass and Iron is heated then brass lies on the convex side.
( R) : Due to lower value of coefficient of linear expansion, brass aquires convex shape.
View SolutionTwo identical rectangular strips. One of copper and the other of steel, are riveted together to from a bimetallic strip. On heating. the strip will
(αcopper>αsteel)
View Solution
