If the two radii of a thin lens are not equal, will the focal length change with change in surface facing the light?
To determine whether the focal length of a thin lens changes when the surfaces facing the light are interchanged, we can analyze the situation using the lens maker's formula.
1. Understanding the Lens Configuration:
- Consider a thin lens with two surfaces having different radii of curvature: R1 and R2.
- The lens can be oriented in two ways: one where the surface with radius R1 faces the incoming light and another where the surface with radius R2 faces the light.
2. Applying the Lens Maker's Formula:
- The lens maker's formula for the focal length f of a thin lens is given by:
1f=(n−1)(1R1−1R2)
- Here, n is the refractive index of the lens material relative to the surrounding medium (usually air).
3. Calculating Focal Length for the First Orientation:
- When the surface with radius R1 faces the light, the formula becomes:
1f1=(n−1)(1R1−1R2)
4. Calculating Focal Length for the Second Orientation:
- When the surface with radius R2 faces the light, we need to consider the sign of the radii. The formula now becomes:
1f2=(n−1)(1R2−1R1)
- This can be rewritten as:
1f2=(n−1)(−(1R1−1R2))=−(n−1)(1R1−1R2)
5. Comparing the Two Focal Lengths:
- From the above calculations, we observe that:
f1=(n−1)1R1−1R2andf2=−(n−1)1R1−1R2
- This indicates that f2 is the negative of f1.
6. Conclusion:
- The focal length does change when the surfaces facing the light are interchanged. Specifically, f2=−f1, indicating that the focal lengths have opposite signs, which means the lens will behave differently depending on which surface is facing the light.
- Consider a thin lens with two surfaces having different radii of curvature: R1 and R2.
- The lens can be oriented in two ways: one where the surface with radius R1 faces the incoming light and another where the surface with radius R2 faces the light.
- The lens maker's formula for the focal length f of a thin lens is given by:
1f=(n−1)(1R1−1R2)
- Here, n is the refractive index of the lens material relative to the surrounding medium (usually air).
- When the surface with radius R1 faces the light, the formula becomes:
1f1=(n−1)(1R1−1R2)
- When the surface with radius R2 faces the light, we need to consider the sign of the radii. The formula now becomes:
1f2=(n−1)(1R2−1R1)
- This can be rewritten as:
1f2=(n−1)(−(1R1−1R2))=−(n−1)(1R1−1R2)
- From the above calculations, we observe that:
f1=(n−1)1R1−1R2andf2=−(n−1)1R1−1R2
- This indicates that f2 is the negative of f1.
- The focal length does change when the surfaces facing the light are interchanged. Specifically, f2=−f1, indicating that the focal lengths have opposite signs, which means the lens will behave differently depending on which surface is facing the light.
Topper's Solved these Questions
- RAY OPTICSBOOK - AAKASH SERIESCHAPTER - RAY OPTICSEXERCISE - PROBLEMS ( LEVEL-I)
57 Videos
- RAY OPTICSBOOK - AAKASH SERIESCHAPTER - RAY OPTICSEXERCISE - PROBLEMS ( LEVEL-II)
60 Videos
- RAY OPTICSBOOK - AAKASH SERIESCHAPTER - RAY OPTICSEXERCISE - EXERCISE (SHORT ANSWER QUESTIONS )
24 Videos
- PRACTICAL PHYSICSBOOK - AAKASH SERIESCHAPTER - PRACTICAL PHYSICSEXERCISE - LECTURE SHEET LEVEL-II (ADVANCED) Linked Comprehension Type Questions
4 Videos
- SEMICONDUCTOR DEVICESBOOK - AAKASH SERIESCHAPTER - SEMICONDUCTOR DEVICESEXERCISE - EXERCISE - III
3 Videos
Similar Questions
If a thin lens is dipped in water, does its focal length change ?
View SolutionIf the lens is placed in water instead of air, how does its focal length change?
View SolutionHow does focal length of a convex lens change with increase in wavelength of incident light?
View SolutionWhich lens, thick or thin has greater focal length?
View SolutionThe focal length of a plano-concave lens is -10 cm , then its focal length when its palne surface is polished is
View SolutionA point object is placed at a distance of 20cm from a thin plano-concex lens of focal length 15cm. The plane surface of the lens is now silvered. The image created by the is at :
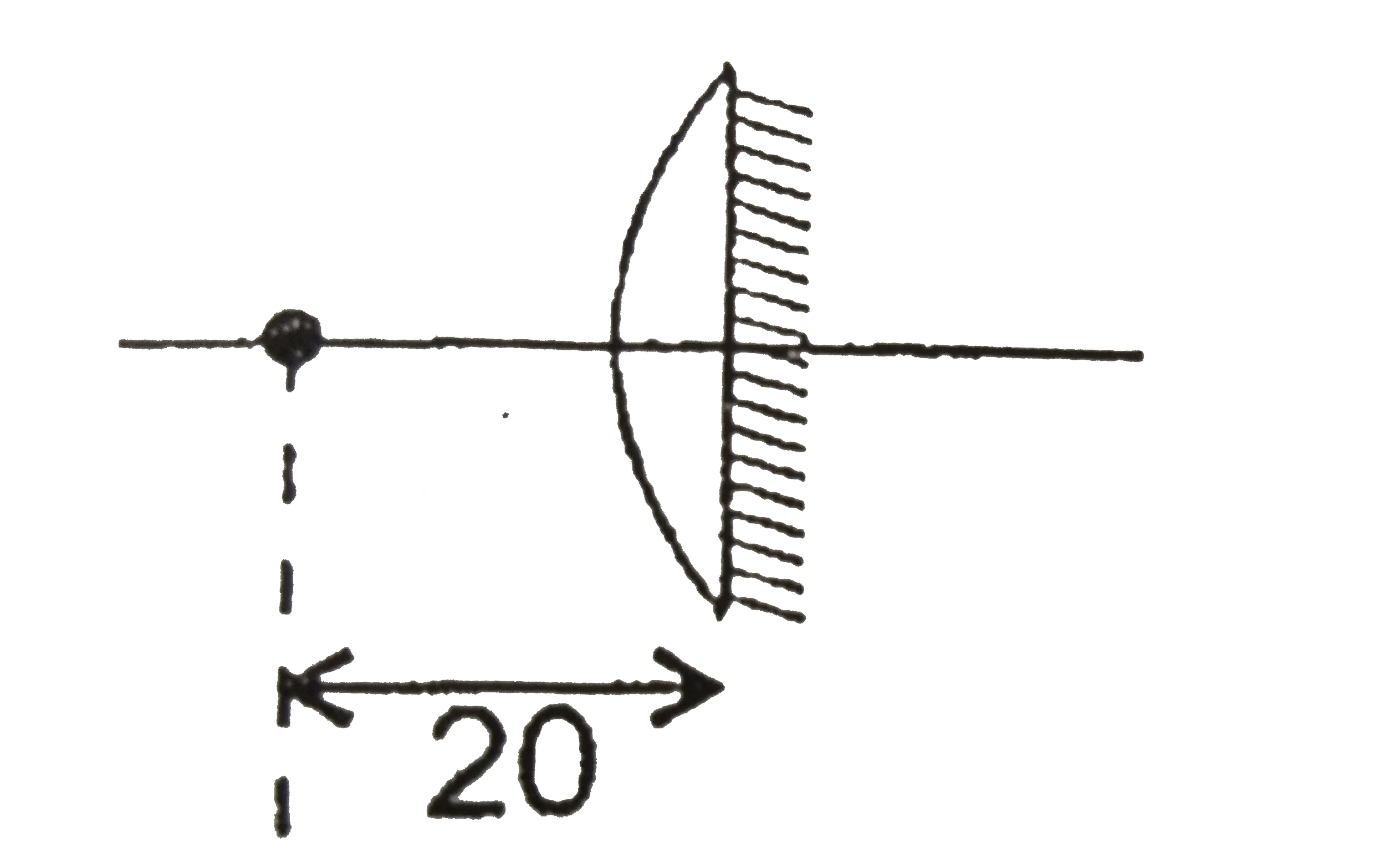
View SolutionShow that the focal length of a thin lens is not changed when the lens is rotated so that the left and the right surfaces are interchanged.
View SolutionA thin convex lens of focal length 20 cm is kept in contact with a thin concave lens of focal length 15 cm. Find the focal length and the nature of the combination.
View SolutionThe focal length of a thin lens in vacuum is f. If the material of the lens has μ=3/2, its focal length when immersed in water of refractive index 4/3 will be.
View SolutionA change in the focal length of the eye lens is brought by.
View Solution
