Calculate the refractive index of material with respect to air if its critical angle is 60∘.
To calculate the refractive index of a material with respect to air given its critical angle of 60∘, we can follow these steps:
1. Understand the Concept of Critical Angle:
The critical angle (C) is the angle of incidence above which total internal reflection occurs when light travels from a denser medium to a rarer medium.
2. Use the Formula for Critical Angle:
The formula relating the critical angle to the refractive indices of the two media is given by:
sinC=μrarerμdenser
where μrarer is the refractive index of the rarer medium (air in this case) and μdenser is the refractive index of the denser medium (the material we want to find).
3. Identify the Refractive Indices:
- The refractive index of air (μair) is approximately 1.
- Let the refractive index of the material be μmaterial=x.
4. Substitute the Known Values:
Given that the critical angle C=60∘:
sin(60∘)=μairμmaterial=1x
5. Calculate sin(60∘):
We know that:
sin(60∘)=√32
6. Set Up the Equation:
Substitute sin(60∘) into the equation:
√32=1x
7. Rearrange to Solve for x:
Rearranging gives:
x=1sin(60∘)=1√32=2√3
8. Final Result:
Thus, the refractive index of the material with respect to air is:
μmaterial=2√3
Final Answer:
The refractive index of the material with respect to air is 2√3.
---
The critical angle (C) is the angle of incidence above which total internal reflection occurs when light travels from a denser medium to a rarer medium.
The formula relating the critical angle to the refractive indices of the two media is given by:
sinC=μrarerμdenser
where μrarer is the refractive index of the rarer medium (air in this case) and μdenser is the refractive index of the denser medium (the material we want to find).
- The refractive index of air (μair) is approximately 1.
- Let the refractive index of the material be μmaterial=x.
Given that the critical angle C=60∘:
sin(60∘)=μairμmaterial=1x
We know that:
sin(60∘)=√32
Substitute sin(60∘) into the equation:
√32=1x
Rearranging gives:
x=1sin(60∘)=1√32=2√3
Thus, the refractive index of the material with respect to air is:
μmaterial=2√3
The refractive index of the material with respect to air is 2√3.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - RAY OPTICSEXERCISE - PROBLEMS ( LEVEL-II)60videos
RAY OPTICS
- BOOK - AAKASH SERIESCHAPTER - RAY OPTICSEXERCISE - EXERCISE (VERY SHORT ANSWER QUESTIONS )53videos
RAY OPTICS
- BOOK - AAKASH SERIESCHAPTER - PRACTICAL PHYSICSEXERCISE - LECTURE SHEET LEVEL-II (ADVANCED) Linked Comprehension Type Questions4videos
PRACTICAL PHYSICS
- BOOK - AAKASH SERIESCHAPTER - SEMICONDUCTOR DEVICESEXERCISE - EXERCISE - III3videos
SEMICONDUCTOR DEVICES
Similar Questions
Write a formula to express the relationship between refractive index of the denser medium with respect to rarer medium and its critical angle for that pair of media.
View SolutionCalculate the refractive index of a liquid with respect to air applying Snell.s law (use geometric construction).
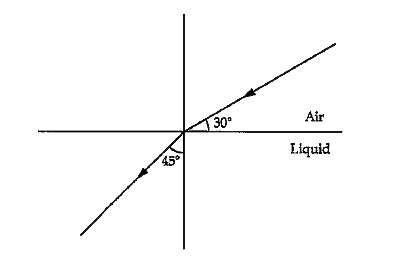 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Refractive index of a transparent material is :
Asame for all colours.Bmaximum for violet colour.Cminimum for violet colour.Dmaximum for red colour.
A plano convex lens has radius of curvature 10 cm. Its focal length is 80 cm under water. Calculate the refractive index of the material of the lens, given refractive index of water is 4/3.
View SolutionThe refractive index of glass with respect to air is 32 and the refraction index of water with respect to air is 43. The refractive index of glass with respect to water will be:
View SolutionThe refractive index of water with respect to air is aμw and of glass with respect to air is aμg. Express the refractive index of glass with respect to water.
View SolutionThe refractive index of water with respect to air is 4/3. What is the refractive index of aiir with respect to water ?
View SolutionWhat is the relation between the refractive index of water with respect to air (aμw) and the refractive index of air with respect to water (wμa).
View SolutionRefractive index of material is equal to tangent of polarising angle. It is called
View SolutionIf the refractive index of water with respect to air (aμw) is 53. Calculate the refractive index of air with respect to water (wμa).
View Solution

