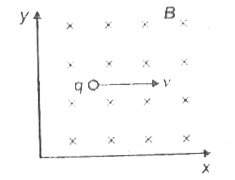
A positive charge 'q' of mass 'm' is moving along the +x axis. We wish to apply a uniform magnetic field B for time Δt so that the charge reverses its direction crossing the y axis at a distance d. Then :
b=mυ2qdandΔt=πd2υ
B=mυqdandΔt=πdυ
B=2mυqdandΔt=πdυ
B=2mυqdandΔt=πd2υ
The correct Answer is:A
To solve the problem, we need to find the expressions for the magnetic field B and the time interval Δt required for a positive charge q of mass m moving along the positive x-axis to reverse its direction after crossing the y-axis at a distance d.
1. Understanding the Motion:
- The charge q is initially moving in the positive x-direction.
- We want it to reverse direction after crossing the y-axis at a distance d.
2. Path of the Charge:
- The charge will follow a semicircular path due to the magnetic field applied perpendicular to its motion.
- Let the radius of the semicircular path be r. The distance d is equal to the diameter of the semicircle, so:
d=2r⇒r=d2
3. Equating Forces:
- The magnetic force acting on the charge is given by:
FB=qvB
- The centripetal force required to keep the charge moving in a circular path is given by:
FC=mv2r
- For the charge to move in a circular path, these forces must be equal:
qvB=mv2r
4. Solving for Magnetic Field B:
- Rearranging the above equation gives:
B=mvqr
- Substituting r=d2:
B=mvq⋅d2=2mvqd
5. Finding the Time Interval Δt:
- The distance traveled by the charge while it is in the magnetic field is the circumference of the semicircle:
Circumference=πr
- The distance traveled can also be expressed as:
Distance=vΔt
- Setting these equal gives:
vΔt=πr
- Substituting r=d2:
vΔt=π⋅d2
- Solving for Δt:
Δt=πd2v
Final Results:
- The magnetic field B is given by:
B=2mvqd
- The time interval Δt is given by:
Δt=πd2v
- The charge q is initially moving in the positive x-direction.
- We want it to reverse direction after crossing the y-axis at a distance d.
- The charge will follow a semicircular path due to the magnetic field applied perpendicular to its motion.
- Let the radius of the semicircular path be r. The distance d is equal to the diameter of the semicircle, so:
d=2r⇒r=d2
- The magnetic force acting on the charge is given by:
FB=qvB
- The centripetal force required to keep the charge moving in a circular path is given by:
FC=mv2r
- For the charge to move in a circular path, these forces must be equal:
qvB=mv2r
- Rearranging the above equation gives:
B=mvqr
- Substituting r=d2:
B=mvq⋅d2=2mvqd
- The distance traveled by the charge while it is in the magnetic field is the circumference of the semicircle:
Circumference=πr
- The distance traveled can also be expressed as:
Distance=vΔt
- Setting these equal gives:
vΔt=πr
- Substituting r=d2:
vΔt=π⋅d2
- Solving for Δt:
Δt=πd2v
- The magnetic field B is given by:
B=2mvqd
- The time interval Δt is given by:
Δt=πd2v
Topper's Solved these Questions
- APPENDICES ( REVISION EXERCISE )BOOK - AAKASH SERIESCHAPTER - APPENDICES ( REVISION EXERCISE )EXERCISE - REVISION EXERCISE (MAGNETISM AND MATTER )
52 Videos
- APPENDICES ( REVISION EXERCISE )BOOK - AAKASH SERIESCHAPTER - APPENDICES ( REVISION EXERCISE )EXERCISE - REVISION EXERCISE (CUTTENT ELECTRICITY )
108 Videos
- ALTERNATING CURRENTBOOK - AAKASH SERIESCHAPTER - ALTERNATING CURRENTEXERCISE - EXERCISE - III
24 Videos
- APPENDICES (REVISION EXERCISE)BOOK - AAKASH SERIESCHAPTER - APPENDICES (REVISION EXERCISE)EXERCISE - LAW OF MOTION
128 Videos
Similar Questions
A positively charged particle is moving along the positive X-axis.You want to apply a magnetic field for a short time so that the particle may reverse its direction and move parallel to the negative X-axis.This can be done be applying the magnetic field along.
View SolutionAn electron is moving along the positive x-axis. You want to apply a magnetic field for a short time so that the electron may reverse its direction and move parallel to the nagative x-axis. This can be done by applying the magnetic field along
View SolutionA charge q of mass m is moving with a velocity of V, at right angles to a uniform magnetic field B. Deduce the expression for the radius of the circular path it describes.
View SolutionA particle of charge q and mass m moving with a velocity v along the x-axis enters the region x>0 with uniform magnetic field B along the ˆk direction. The particle will penetrate in this region in the x-direction upto a distance d equal to
View SolutionA positively charged particle moving along x-axis with a certain velocity enters a uniform electric field directed along positive y-axis. Its
View SolutionA charge particle of charge q and mass m is moving with velocity v as shown in fig In a uniform magnetic field B along -ve z-direction.Select the correct alternative (s).

View SolutionA moving charged particle q travelling along the positive X-axis enters a uniform magnetic field B. When will the force acting on q be maximum?
View SolutionA charge q moves with a velocity 2m/s along x- axis in a unifrom magnetic field B=(ˆi+2ˆj+3ˆk) tesla.
View SolutionMagnetic field exist in the space and given as →B=−B0l2x2ˆk, where B0and l positive constants. A particle having positive charge 'q' and mass 'm' is pojected wit speed 'v0' along positive x axis from the origin. What is the maimum distance of the charged particle from the y-axis before it turns back due to the magnetic field. (Ignore any interaction other than magnetic field)
View SolutionA positive charge particle with charge q is moving with speed v in a region of uniform magnetic field B at the instant shown in figure. An external electric field so to be applied so that the charged particle follows a straight line path. The magnitude and direction of electric field required are respectively.
View Solution
