On a logarithmic scale, the relation between species richness and area is described by the following equation:
logS=logC+ZLogA
In the above equation 'Z' represents
Y-intercept
Species richness
Regression coefficient
Area explored
The correct Answer is:C
To solve the question regarding the equation logS=logC+ZlogA, we need to identify what the variable 'Z' represents in the context of the species-area relationship. Let's break it down step by step:
1. Understanding the Equation: The equation logS=logC+ZlogA describes the relationship between species richness (S) and area (A) on a logarithmic scale. Here, S is the species richness, C is a constant, A is the area, and Z is a coefficient.
2. Identifying the Variables:
- S (Species Richness): This represents the number of different species in a given area.
- C (Constant): This is the y-intercept of the relationship when plotted on a logarithmic scale.
- A (Area): This is the area being considered in relation to species richness.
- Z (Coefficient): This is the variable we need to define.
3. Understanding the Role of 'Z': In the context of the species-area relationship, 'Z' quantifies how species richness changes with area. It is often referred to as the slope of the line when the relationship is plotted on a logarithmic scale. This means that 'Z' indicates the rate at which species richness increases as the area increases.
4. Conclusion: Based on the definitions and roles of the variables in the equation, we can conclude that 'Z' represents the regression coefficient, which indicates the relationship between the area and species richness.
Final Answer:
In the equation logS=logC+ZlogA, 'Z' represents the regression coefficient.
---
- S (Species Richness): This represents the number of different species in a given area.
- C (Constant): This is the y-intercept of the relationship when plotted on a logarithmic scale.
- A (Area): This is the area being considered in relation to species richness.
- Z (Coefficient): This is the variable we need to define.
In the equation logS=logC+ZlogA, 'Z' represents the regression coefficient.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - BIODIVERSITY AND CONSERVATIONEXERCISE - EXERCISE-I The Importance of Species Diversity to the Ecosystem9videos
BIODIVERSITY AND CONSERVATION
- BOOK - AAKASH SERIESCHAPTER - BIODIVERSITY AND CONSERVATIONEXERCISE - EXERCISE-I Loss of Biodiversity28videos
BIODIVERSITY AND CONSERVATION
- BOOK - AAKASH SERIESCHAPTER - ANIMAL KINGDOM EXERCISE - EXERCISE -III (Previous AIPMT/NEET Questions)35videos
ANIMAL KINGDOM
- BOOK - AAKASH SERIESCHAPTER - BIOLOGICAL CLASSIFICATIONEXERCISE - EXERCISE-III (Previous AIPMT/NEET Question)37videos
BIOLOGICAL CLASSIFICATION
Similar Questions
Knowledge Check
- Question 1 - Select One
On a logarithmic scale, the species area relationship is a straight line described by the equation log S = log C + Z log A.
What does S, C, Z and A represent in the given equation? Select the correct answer from the codes given below.
Species richness=1Slope of the line=2Y-intercept=3Area=4
a) 1 2 3 4
C S Z A
b) 1 2 3 4
S Z C A
c) 1 2 3 4
Z S C A
d) 1 2 3 4
A C S ZAa) 1234CSZABb) 1234SZCACc) 1234ZSCADd) 1234ACSZ
The relation between species richness and area is described on a logarithmic scale by the equation [where S = species richness, A = area, Z = slope of the line (regression coefficient ), C= Y - intercept]
View SolutionThe relation between specises richness and area is described on a logarithmic scale by the equation [where S = species richness, A = area, Z = slope of the line (regression coefficient ), C= Y - intercept]
View SolutionAlexander von Humboldt gave the relationship between the species richness and its geographical area . Which of the following is the correct logarithmic equation for his findings ?
View SolutionThe relation between species richness and area for a wide variety of taxa turns out to be a rectangular hyperbola. Give a brief explanation.
View Solution
The graph above could represent which of the following equations?View Solution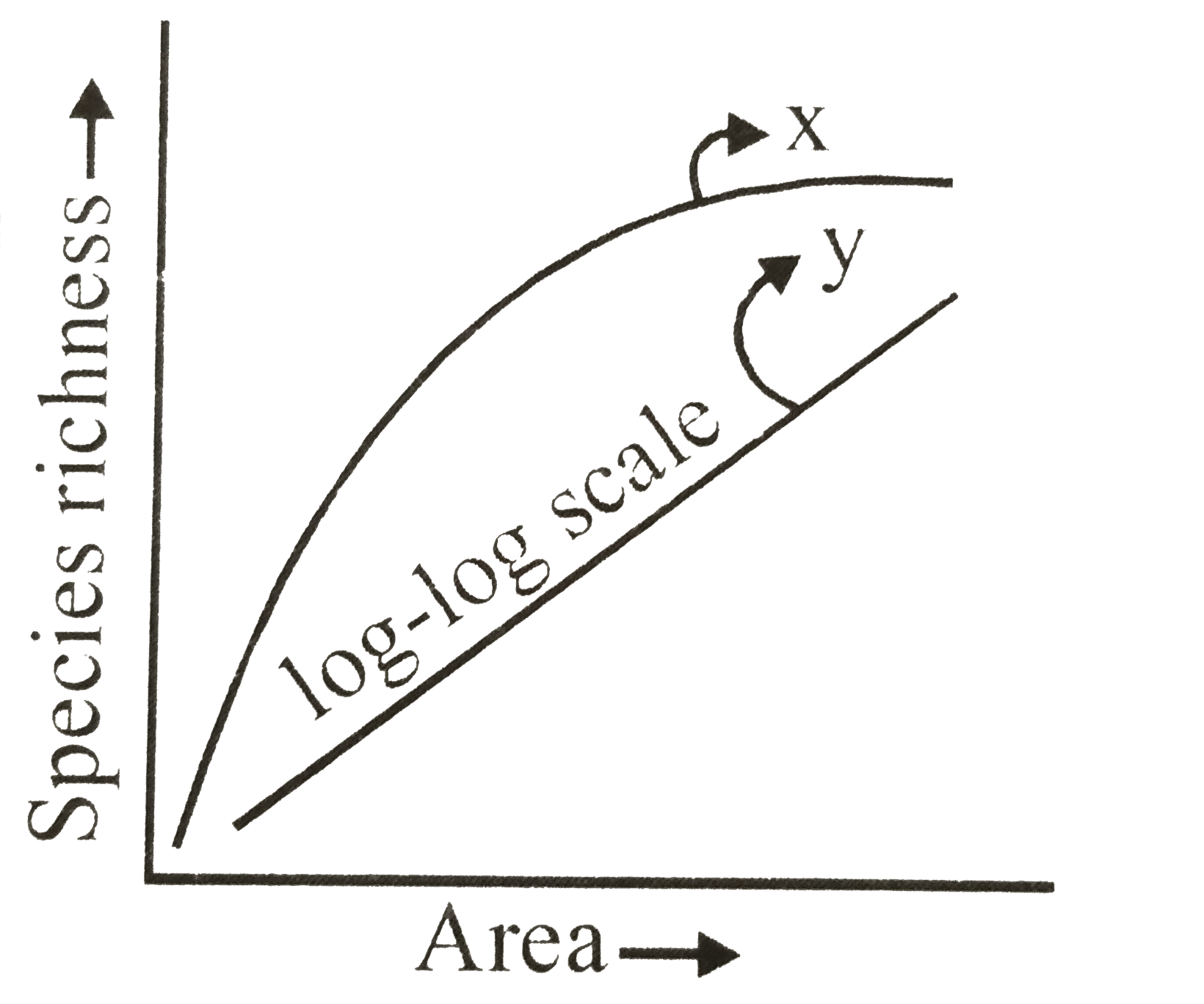
Study the above graph and answer the following questions -
(i) Identify x and y
(ii) The relation between species richness and area can be denoted as generally
(iii) The value of z lies in range of
(iv) On a log scale the relation between species richness and area isView SolutionThe population growth is generally described by the following equation : dNdt=rN(K−NK) What does 'r' represent in the given equation ?
View Solution


