For the reaction
(i) AkI−−→P
(ii) BKII−−→Q, following observation is made.
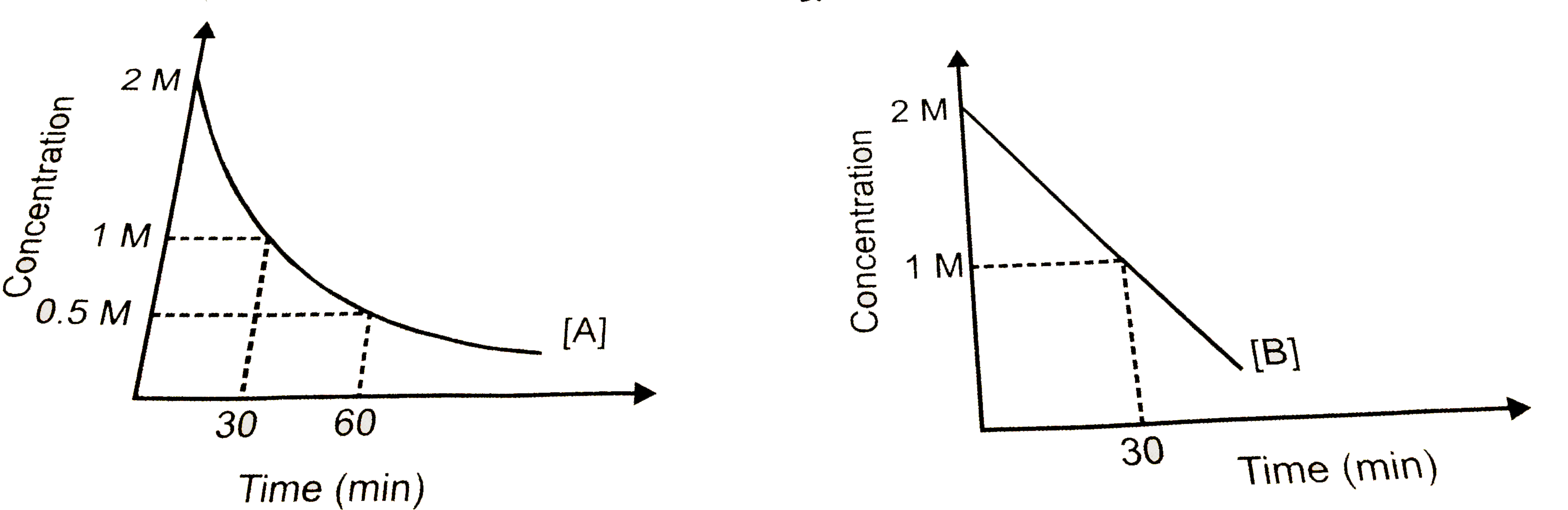
Calculate k1kII, where kI and kII and rate constant for the respective reaction.Solution in Telugu
2.303
1
0.36
0.693
The correct Answer is:D
Step by step video & image solution for For the reaction (i) Aoverset(k_(I))toP (ii) Boverset(K_(II))toQ, following observation is made. Calculate (k_(1))/(k_(II)), where k_(I) and k_(II) and rate constant for the respective reaction. by Chemistry experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CHEMICAL KINETIC & NUCLEAR CHEMISTRYBook:NARENDRA AWASTHIChapter:CHEMICAL KINETIC & NUCLEAR CHEMISTRYExercise:Level 1 (Q.3 To Q.32)
Explore 2 Videos
- CHEMICAL KINETIC & NUCLEAR CHEMISTRYBook:NARENDRA AWASTHIChapter:CHEMICAL KINETIC & NUCLEAR CHEMISTRYExercise:Level 1 (Q.33 To Q.62)
Explore 3 Videos
- SURFACE CHEMISTRYBook:NARENDRA AWASTHIChapter:SURFACE CHEMISTRYExercise:Level 3 - Assertion - Reason Type Questions
Explore 1 Video
Similar Questions
The reaction of A2 and B2 follows the equation
A2(g)+B2(g)→2AB(g)
The following data were observed
[A2]0[B2]0Initial rate of appearance of AB(g)(inMs−10.100.102.5×10−40.200.105×10−40.200.2010×10−4
The value of rate constatnt for the above reaction is :
View SolutionHalf-life (t1/2) and completion time (T) of the reaction (A→2B) are if initial concentration A is 1M and k = 0.001M/ min :
View Solutioneach question constain STATEMENT-1(Assertion ) and STATEMENT - 2 (reason). examine the statement carefully and work the correct answer accoridng to the instructions given below :
STATEMENT-1: Dispersed phase particles of colloidal solution cannot pass through ultra -filter paper.
STATEMENT-2: The size of colloidal particles are larger than the size of true solution particles.
View SolutionConisder the following reactions:
I. A+Bk1⇐⇒k−1C
II. C+Bk2−−→D
Then k1[A][B]−k−1[C]−k2[C][B] is equal to
View SolutionObserve the following reaction I and II k_(1),k_(1)^('),k_(2),k_(2)^(') are rate constant. Select the correct option(s). (I). underset([K_(1)^(')])overset((CH_(3)CH_(2))_(3)N)larrCH_(3)-I underset([k_(2)^(')])overset((CH_(3)CH_(2))_(3)N)larrCH_(3)-underset(I)underset(|)CH-CH_(3)
View SolutionFor the reaction (i) Aoverset(k_(I))toP (ii) Boverset(K_(II))toQ , following observation is made. Calculate (k_(1))/(k_(II)) , where k_(I) and k_(II) and rate constant for the respective reaction.
View SolutionIn the series reaction Aoverset(K_(2))(rarr)Boverset(K_(2))(rarr)Coverset(K_(2))(rarr)D , if K_(1)gtK_(2)gtK_(3) then the rate determing step of the reaction is
View SolutionFor the reactions (i) AKI−−→P and (ii) BKII−−→Q, following observation is made.
Calculate KIKII where KI and KII are rate constants for the respective reaction.
View SolutionConsider the following hypothetical reactions
Ak1⇐⇒k2B , Bk3−−→slowC
The overall rate constant kθ of the reaction is
View SolutionA reaction ArarrB , involes following mechanism : Step1 : Aoverset(k_(1))rarrB" "("fast") Step2 : Boverset(k_(2))rarrC " "("slow") Step3: Coverset(k_(3))rarrD " "("fast") The rate law of the reaction may be given as :
View Solutionअभिक्रिया भागफल (Q ) तथा साम्य सिथरांक (Kc) में निम्नलिखित सम्बन्ध है, तो अभिक्रिया की दिशा का निर्धारण कीजिये।
(i) Q<Kc, (ii) Q>Kc, (iii) Q=Kc
View SolutionGiven
i)A↔B+CKeq(1)<br>ii)B+C↔PKeq(2)
then Keq for reaction A↔pis
View Solution(i) Derive a general expression for the equilibrium constant Kp, and Kc for the reaction.
(ii) Write the Kp and KC for NH3 formation reaction.
View SolutionIf a unimolecular reaction, A(g) to Products, takes place according to the mechanism (I) A+Aoverset(k_(1))underset(k_(-1))(hArr)A^(a)+A (II) A^(a)underset(k_(2))(to)P where k_(1) , k_(-1) and k_(2) are the rate constants and P, A and A^(a) stand for product molecule, normal molecule of reactant and activated molecules of reactant and activated molecules of reactant respectively. For the reaction scheme, X+Yoverset(k_(f))underset(k_(b))(hArr)Z , Y+Zoverset(k)(to)T , the rate equation for the formation of T will be
View SolutionIf a unimolecular reaction, A(g)→ Products, takes place according to the mechanism
(I)A+Ak1⇐===⇒k−1Aa+A
(II)Aa−−→k2P
where k1, k−1 and k2 are the rate constants and P,A and Aa stand for product molecule, normal molecule of reactant and activated molecules of reactant and activated molecules of reactant respectively.
The order 'n' initial concentration a0 and time of half reaction t1/2, for a reaction are related as
View SolutionFor the reaction
(i) Ak1−−→P
(ii) BkII−−→Q, following observation is made.
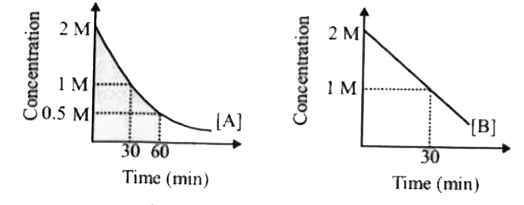
Calculate kIkII, where kI and kII are rate constant for the respective reaction.
View Solution