Question

Derive the balancing condition of a Wheatstone bridge.

Explanation

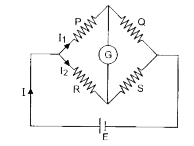
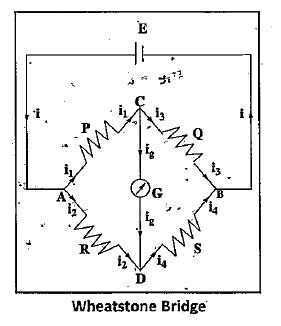
Wheatstone.s bridge - Balancing condition : Wheatstone.s bridge consists of four resistances P, Q, R, S connected as shown in the figure and are referred as arms of the bridge. A battery of emf .E. is connected between two junctions A. and B and a galvanometer of resistance .G. is connected between .C. and .D.
Applying Kirchoff.s first law,
at the junction C, `i_(1)= i_(g) + i_(3)` ...(1)
at the junction D, `i_(2)+ i_(g)+ i_(4)` ....(2)
Applying Kirchoff.s second law for the loop ACDA `-i_(1)P- i_(g) G+ i_(2)R= 0` ...(3)
For the loop CBDC, `-i_(3)Q + i_(4) S + i_(g)G= 0` .... (4)
If no current passes through the galvanometer then the bridge is said to be balanced. So, `i_(g)=0`, then (1), (2), (3) and (4) becomes `i_(1)= i_(3), i_(2)= i_(4), i_(1)P= i_(2)R and i_(3)Q= i_(4)S`
`therefore (i_(1)P)/(i_(3)Q) = (i_(2)R)/(i_(4)S)`
But `i_(1), i_(3) and i_(2) = i_(4)`
`therefore (P)/(Q)= (R )/(S)`

This is the balancing condition of a Wheatstones bridge.