A school wants to conduct tree plantation programme. For this a teacher allotted a circle of radius 6m ground to nineth standard students for planting sapplings. Four students plant trees at the points A,B,C and D as shown in figure. Here AB = 8m, CD = 10m and AB⊥CD. If another student places a flower pot at the point P, the intersection of AB and CD, then find the distance from the centre to P.

The correct Answer is:5.6m
Topper's Solved these Questions
- GEOMETRY BOOK - NCERT TAMILCHAPTER - GEOMETRY EXERCISE - EXERCISE 4.7
20 Videos
- GEOMETRY BOOK - NCERT TAMILCHAPTER - GEOMETRY EXERCISE - EXERCISE 4.3
12 Videos
- GEOMETRICAL CONSTRUCTIONSBOOK - NCERT TAMILCHAPTER - GEOMETRICAL CONSTRUCTIONSEXERCISE - EXERCISE 13.2
10 Videos
- LINEAR EQUATIONS IN TWO VARIABLES BOOK - NCERT TAMILCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - EXERCISE 8.5
12 Videos
Similar Questions
In a tree plantation programme, 40 students planted various number of plants . 8 students planted 5 saplings each. What is the percenteage of these students ? What is the percentage of the remaining students ?
View SolutionAB and CD are common tangents to two circles which intersect each other at C as shown in the figure IF AB = 6cm , find CD.

View Solution
Knowledge Check
There is certain numbers of toys in the box. They are divided into such a way that the person who gets 1/4 of the whole gets thrice of what the others get on an average. Find the number of people amongst whom the toys are distributed?
AA) 8BB) 10CC) 12DD) 9If two vertical pale AB and CD of height 15 m and 10 m and A and C are on ground. P is the point of intersection of BC and AD. What is height of P from the ground in m.
A203B103C5D−6In the figure , l||m ||n and AB||CD , then ∠BCD is -
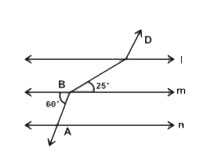 A120∘B145∘C85∘D60∘
A120∘B145∘C85∘D60∘
In Figure, l is a line intersecting the two concentric circles, whose common centre is O, at the points A,B,C and D . Show that AB=CD.
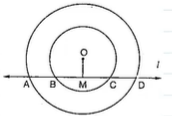
View SolutionIn Figure, l is a line intersecting the two concentric circles, whose common centre is O, at the points A, B, C and D . Show that AB=CD.
View SolutionAB and CD are two chords of a circle where AB = 10 cm, CD = 24 cm and AB||CD. Distance between AB and CD is 17 cm. Find the radius of the circle. (The chords are located on opposite sides of the centre)
View SolutionAB and CD are two chords of a circle such that AB = 10 cm, CD - 24 cm and AB||CD. If the distance between AB and CD is 17 cm. Then, the radius of the circle is equal to
View SolutionAB and CD are two chords of a circle such that AB = 10 cm, CD - 24 cm and AB||CD. If the distance between AB and CD is 17 cm. Then, the radius of the circle is equal to
View Solution
