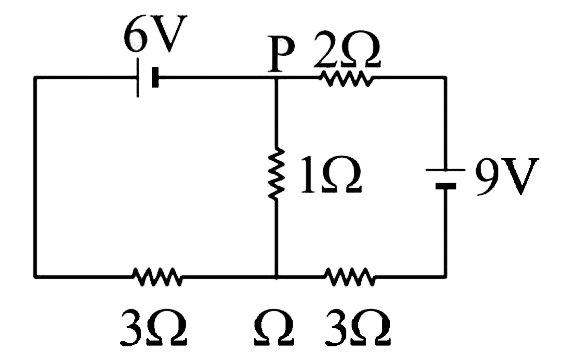
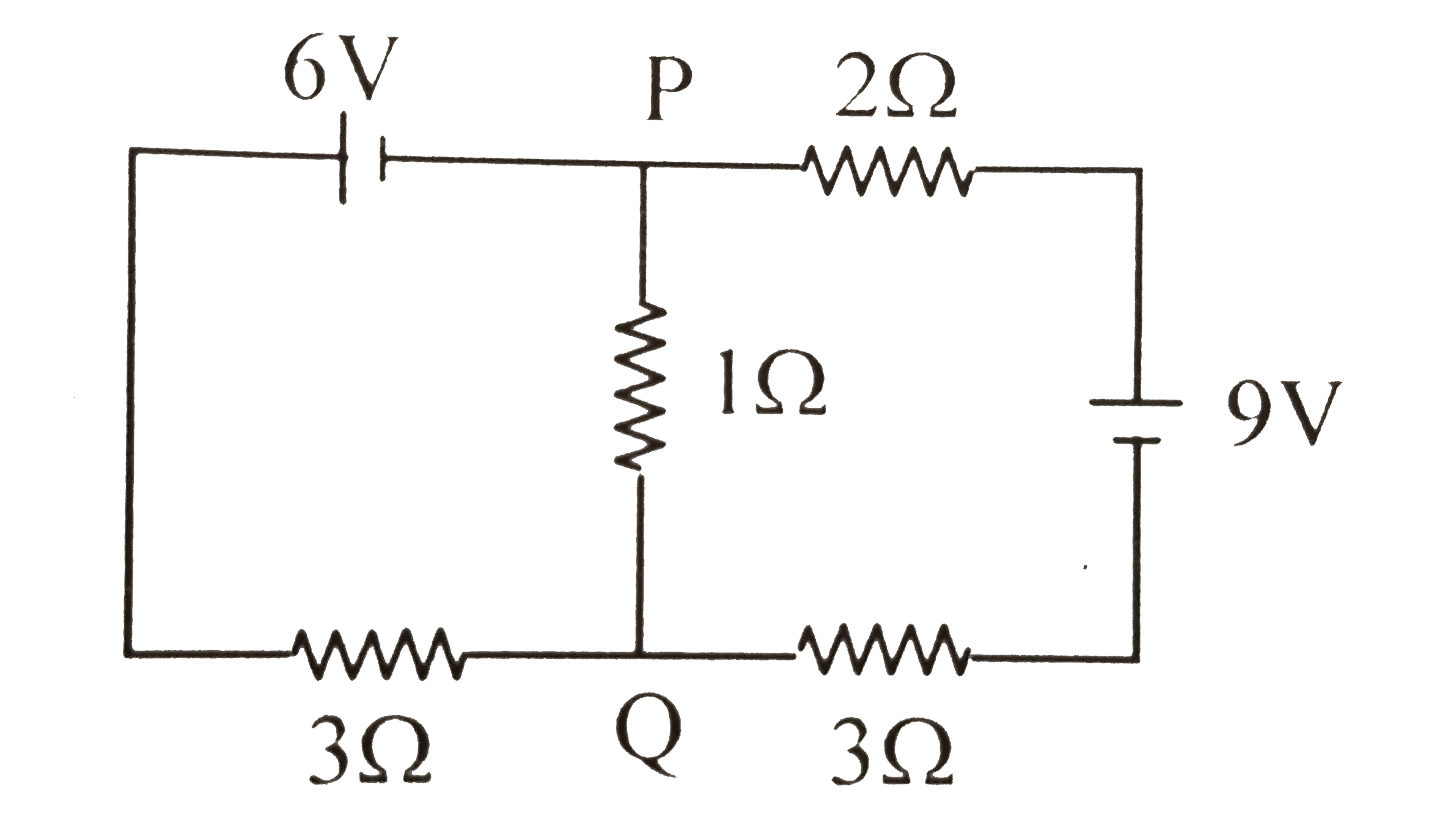
For the circuit given below, find the current (in A) in the 1Ω resistor ? Assume the batteries are ideal.
Text Solution
The correct Answer is:2.48
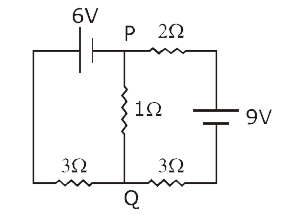
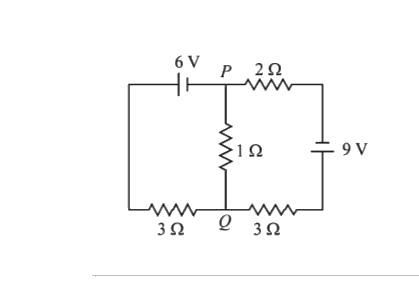
The circuit given in the problem can be redrawn as shown below.
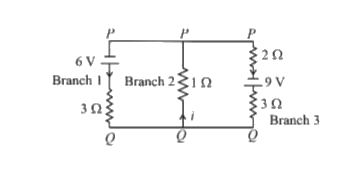
The three branches are connected between the same points P and Q and hence they are in parallel. For such a circuit the potential difference between the points P and Q is:
VQP=E1/E2+E2/R2+E3/R31/R1+1/R2+1/R3
Where E1,E2 and E3 are the emf of the batteries present in the first, second and the third branch. Similarly, R1,R2 and R3 are the values of resistance in each of the respective branches.
VQP=6/3+0/1+9/51/3+1/1+1/5=5723 V
The value of current through the 1Ω resistance is:
i=VQP1=5723A=2.48A
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
Consider the circuit shown below. The current in the 4Ω resistor is
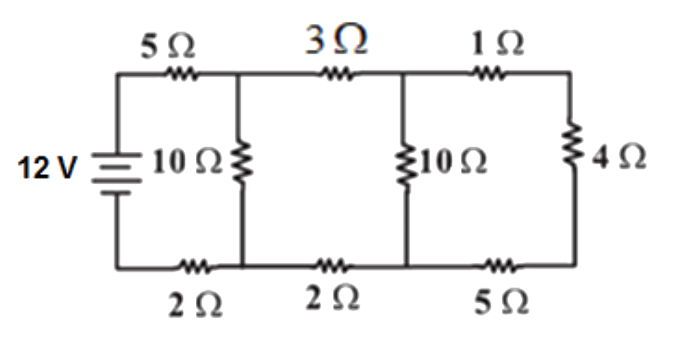 A0.25AB0.50AC0.75AD1.00A
A0.25AB0.50AC0.75AD1.00A
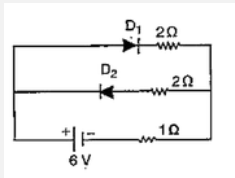
For the circuit shown in Fig., find the current flowing through the 1Ω resistor. Assume that the two diode are ideal diode.
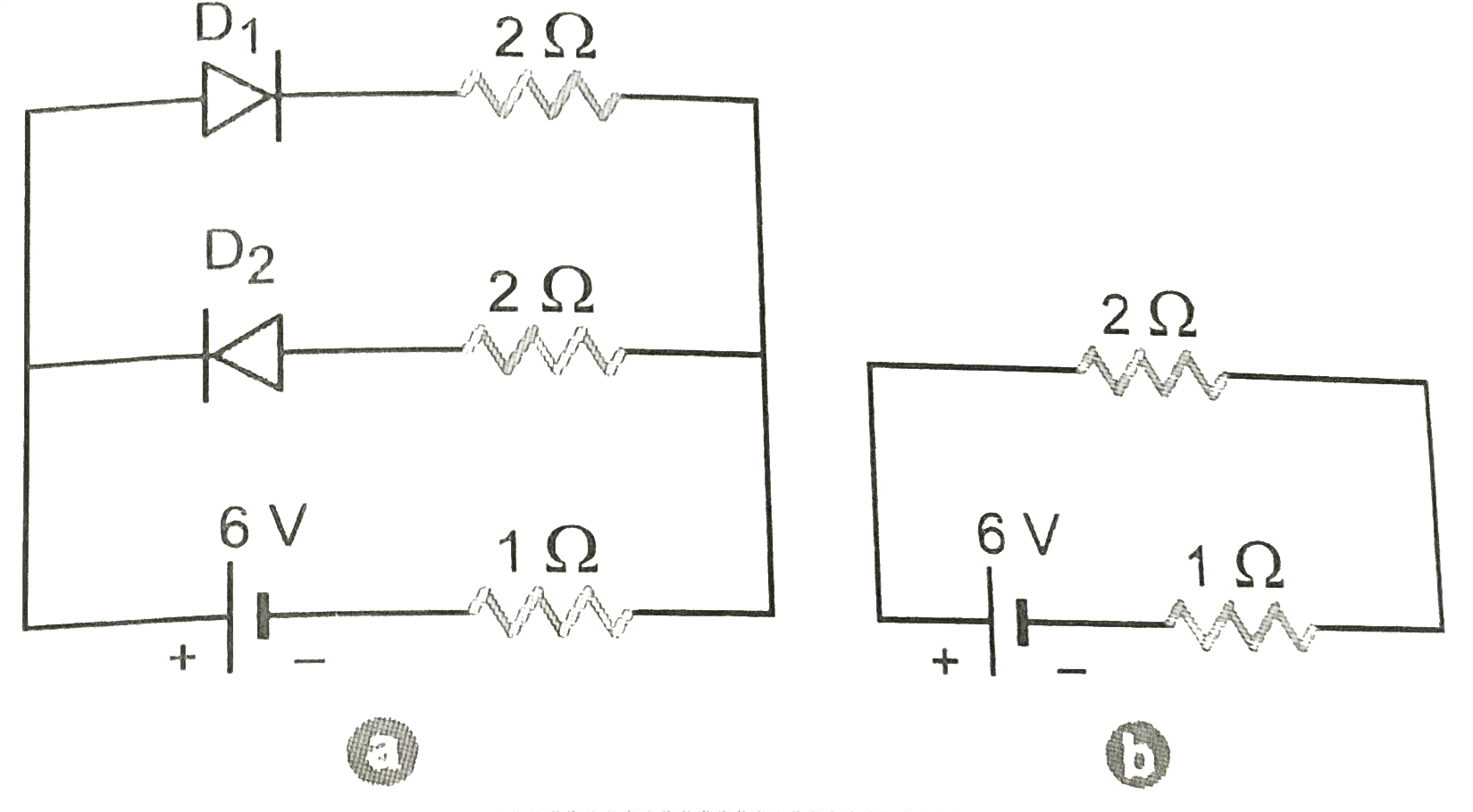
View SolutionFor the circuit shown here, find the current flowing through the 1Ω resistor. Assume that the two diodes D1 and D2 are ideal diodes.
View SolutionConsider the circuit shown below. The current in the 4Ω resistor is

View SolutionIn the circuit diagram shown in find the current through the 1Ω resistor.
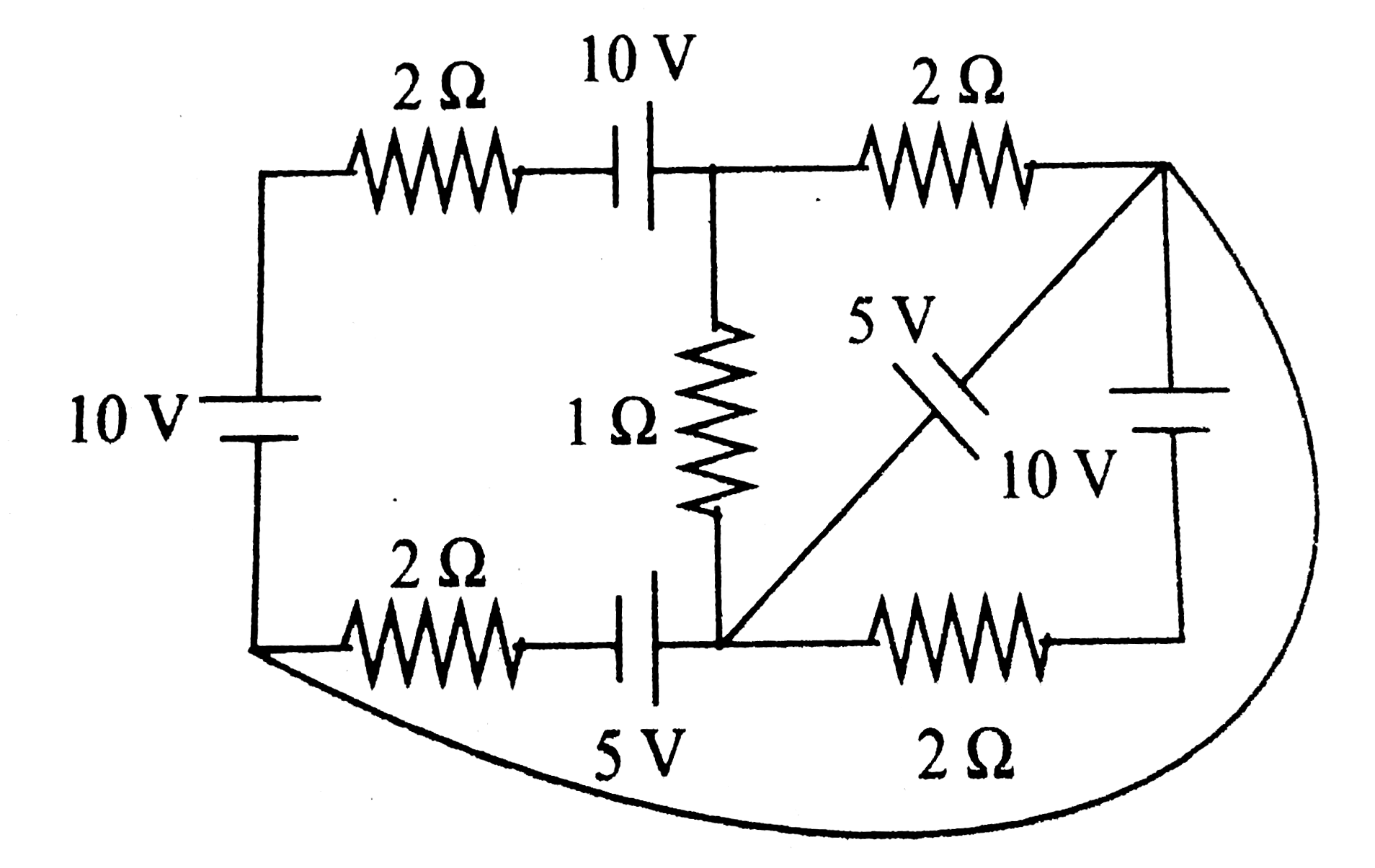
View SolutionIn the circuit diagram shown in find the current through the 1Ω resistor.

View SolutionIn the circuit shown, the current in the 1Ω resistor is :
View SolutionIn the circuit shown, the current in the 1Ω resistor is :

View Solution