Suppose the energy of a hydrogen–like atom is given as En=−54.4n2eV where n∈N. Calculate the following:
If the atom is in ground state, compute its first excitation potential and also its ionization potential.
For a hydrogen-like atom, ground state energy is E1=−13.6n2Z2eV
where Z is the atomic number. Hence, comparing this energy with given energy, we get, −13.6Z2=−54.4⇒Z=±2. Since, atomic number cannot be negative number, Z = 2.
Topper's Solved these Questions
- BOOK - NCERT TAMILCHAPTER - ATOMIC AND NUCLEAR PHYSICSEXERCISE - EVALUATION (Multiple Choice Questions)15videos
ATOMIC AND NUCLEAR PHYSICS
- BOOK - NCERT TAMILCHAPTER - ATOMIC AND NUCLEAR PHYSICSEXERCISE - EVALUATION (Exercises)13videos
ATOMIC AND NUCLEAR PHYSICS
- BOOK - NCERT TAMILCHAPTER - ALTERNATING CURRENTEXERCISE - ADDITIONAL EXERCISES19videos
ALTERNATING CURRENT
- BOOK - NCERT TAMILCHAPTER - ATOMSEXERCISE - ADDITIONAL EXERCISE10videos
ATOMS
Similar Questions
The energy level excitation potential of a hydrogen -like atom is shown in Figure
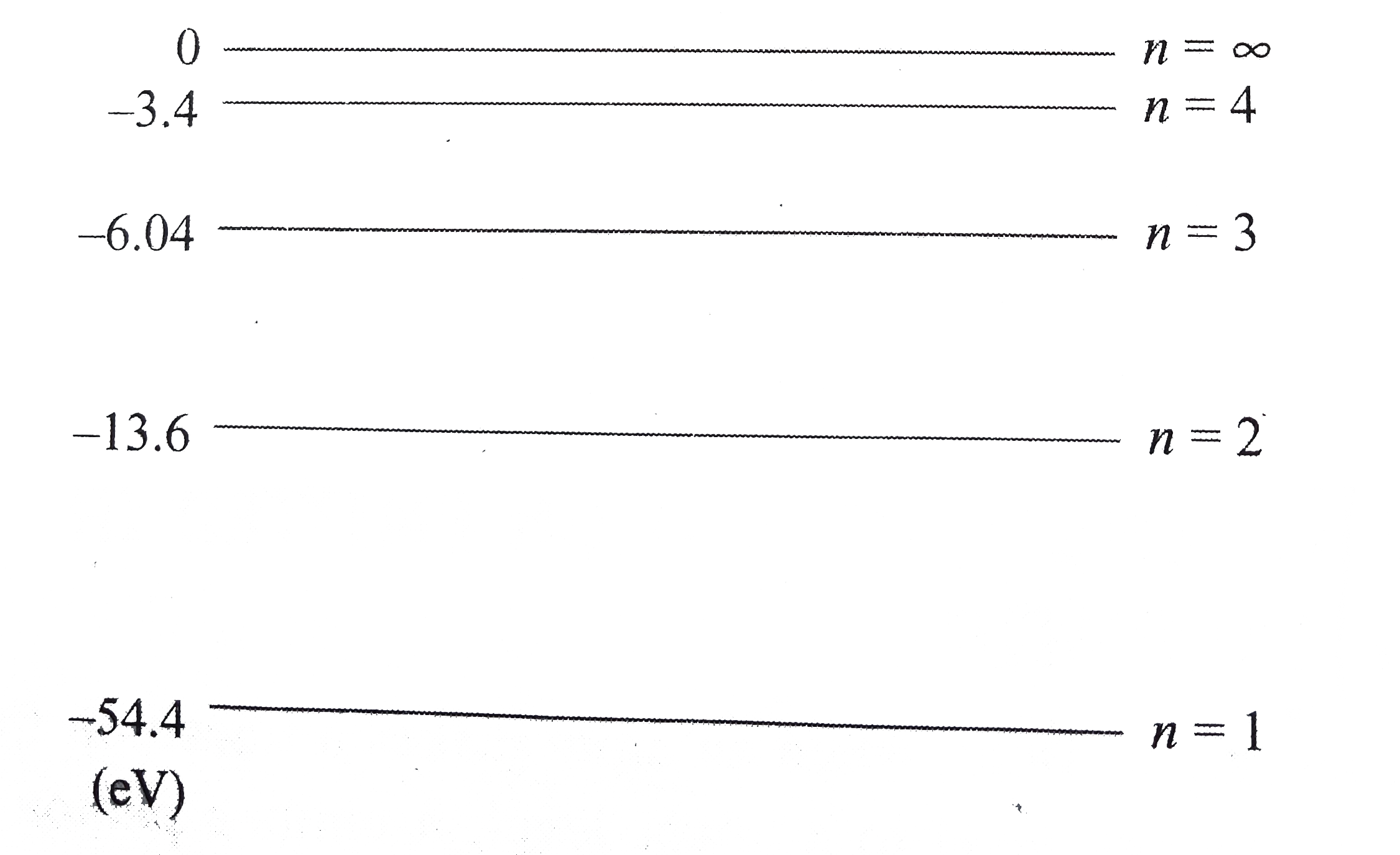
a Find the value of Z
b If initially the atom is in the ground state ,then
(i) determine its excitation potential, and
m (ii) determine its ionization potential.
c Can it absorbe a photom of 42eV?
d Can it absorbe a photom of 56eV?
e Calculate the radius of its first Bohr orbit.
f Calculate the kinectic and potential energy of an electron in the first orbit.View SolutionThe energy level excitation potential of a hydrogen -like atom is shown in Figure

a Find the value of Z
b If initially the atom is in the ground state ,then
(i) determine its excitation potential, and
m (ii) determine its ionization potential.
c Can it absorbe a photom of 42eV?
d Can it absorbe a photom of 56eV?
e Calculate the radius of its first Bohr orbit.
f Calculate the kinectic and potential energy of an electron in the first orbit.View Solution
Knowledge Check
- Question 1 - Select One
If the potential energy of a H-atom in the ground state be zero then its potential energy in the first excited state will be
A10.2evB20.4eVC23.8eVD27.2eV - Question 2 - Select One
Let the potential energy of the hydrogen atom in the ground state be zero . Then its energy in the excited state will be
A10.2eVB13.6eVC23.8eVD27.2eV
Calculate for a hydrogen atom the ionization potential, the first excitation potential and the wavelength of the resonance line (n'=2→n=1).
View SolutionThe energy levels of a hypothetical one electron atom are given by En=−18.0n2eV
where n = 1,2,3,…. (a) Compute the four lowest energy levels and construct the energy levels diagram. (b) What is the first excitation potential (c ) What wavelength (Å) can be emitted when these atoms in the ground state are bombarged by electrons that have been accelerated through a potential difference of 16.2 V? (e) what is the photoelectric threshold wavelength of this atom?View SolutionThe energy levels of a hypothetical one electron atom are given by En=−18.0n2eV
where n = 1,2,3,…. (a) Compute the four lowest energy levels and construct the energy levels diagram. (b) What is the first excitation potential (c ) What wavelength (Å) can be emitted when these atoms in the ground state are bombarged by electrons that have been accelerated through a potential difference of 16.2 V? (e) what is the photoelectric threshold wavelength of this atom?View SolutionThe energy of stable states of the hydrogen atom is given by En=−2.18×10−8/n2[J] where n denotes the principal quantum number.
Calculate the energy differences between n=2 (first excited state) and n=1 (ground state) and between n=7 and n=1.View SolutionThe energy of stable states of the hydrogen atom is given by En=−2.18×10−8/n2[J] where n denotes the principal quantum number.
Calculate the energy differences between n=2 (first excited state) and n=1 (ground state) and between n=7 and n=1.View SolutionLet the potential energy of the hydrogen atom in the ground state be zero . Then its energy in the excited state will be
View Solution

