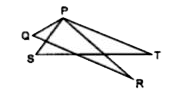
In the given if ΔPQR~ΔPSTandPT:ST=3:4 then QR : PR =
A
3:4
B
4:3
C
3:7
D
4:7
Text Solution
The correct Answer is:B
|
Topper's Solved these Questions
- SIMILARITY BOOK - ICSECHAPTER - SIMILARITY EXERCISE - MULTIPLE CHOICE QUESTIONS (ASSERTION AND REASON BASED QUESTIONS)
5 Videos
- SIMILARITY BOOK - ICSECHAPTER - SIMILARITY EXERCISE - MULTIPLE CHOICE QUESTIONS (COMPETENCY BASED QUESTIONS)
10 Videos
- SHARES AND DIVIDENDSBOOK - ICSECHAPTER - SHARES AND DIVIDENDSEXERCISE - Exercise 3(C )
20 Videos
- SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)BOOK - ICSECHAPTER - SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)EXERCISE - EXERCISE 15(E)
61 Videos
Similar Questions
In the figure, given alongside, ∠QPS=∠RPT
and ∠PRQ=∠PTS.

If PT : ST = 3:4, find the ratio between QR : PR.
View SolutionS and T are points on the sides PQ and PR, respectively of ΔPQR, such that PT = 2 cm, TR = 4 cm and ST is parallel to QR. Find the ratio of the areas of ΔPST and ΔPQR.
View Solution
Knowledge Check
It is given that ΔABC~ΔPRQandBCQR=23 then ar(ΔPQR)ar(ΔABC)=?
A23B32C49D94In ΔDEFandΔPQR, if PQ =DE, EF=PR and FD =QR, then
AΔDEF≅ΔRPQBΔDEF≅ΔQPRCΔDEF≅ΔQRPDΔDEF≅ΔPQRIn ΔDEFandΔPQR, if PQ =DE, EF=PR and FD =QR, then
AΔDEF≡ΔRPQBΔDEF≡ΔQPRCΔDEF≡ΔQRPDΔDEF≡ΔPQR
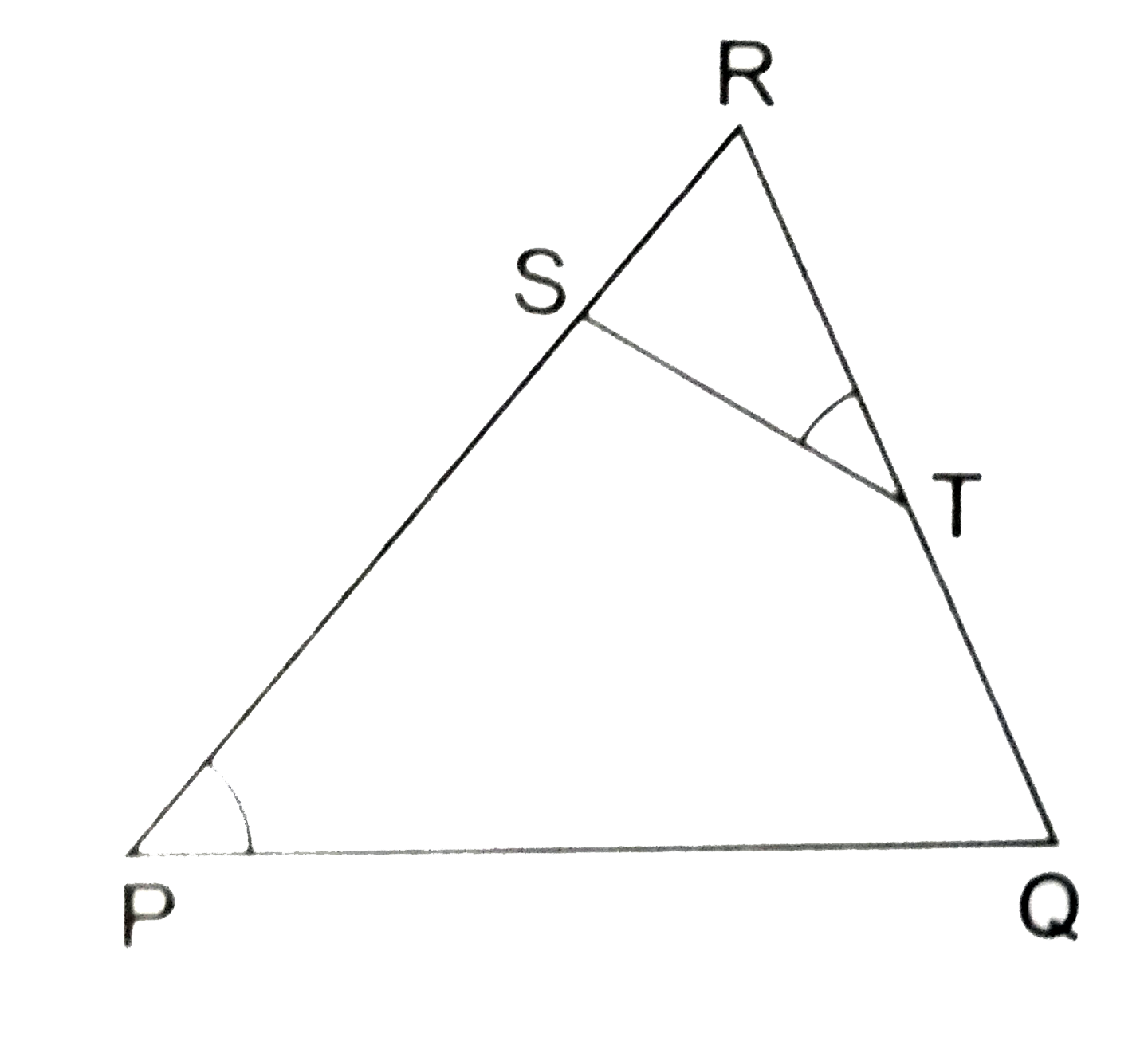
In the given figure, S and T are points on sides PR and QR of ΔPQR such that ∠P=∠RTS. Show that ΔRPQ~ΔRTS.
View SolutionName the types of following triangles :
Δ PQR such that PQ = QR = PR = 5 cm.
View SolutionIn ΔDEFandΔPQR, if PQ = DE , EF = PR and FD = QR , then
View SolutionIn a triangle PQR, PQ=20 cm and PR =6 cm, the side QR is :
View SolutionIt is given that ΔABC−ΔPQR, with BCQR=14. Then arΔPQRarΔABC is equal to:
View Solution
