Calculate the area of right triangle PQR where ,OP=6cm,OR=8cm,and QR=26cm
Step by step video, text & image solution for Calculate the area of right triangle PQR where ,OP=6cm,OR=8cm,and QR=26cm by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
The area of the circumcircle of a right angle triangle whose sides are 6 cm, 8 cm and 10 cm will be:
A9πcm2πB25πcm2C24.5πcm2D16πcm2
Construct a rectangle PQRS with PQ=5.2 cm and QR=2.6 cm.
View SolutionConstruct a rectangle PQRS with PQ=5.2 cm and QR=2.6 cm.
View SolutionThe sides of certain triangle are given below. Determine which of them are right triangle: a = 6 cm, b = 8cm and c = 10cm a = 5cm, b = 8cm and c = 11cm.
View SolutionIn fig , a circle isinscribed in a triangle PQR with PQ = 10 cm , QR = 8 cm and PR = 12 cm .Find the length of the QM , RN and PL.
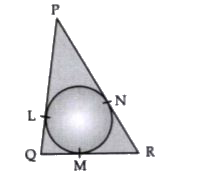 View Solution
View SolutionThe sides of certain triangles are given below. Determine which of them are right triangles: a=6cm , b=8cm and c=10cm (ii) a=5cm , b=8cm and c=11cm .
View SolutionA triangle and a parallelogram have the same base and the same area. If the sides of the triangle are 26 cm, 28 cm and 30 cm, and the parallelogram stands on the base 28 cm, find the height of the parallelogram.
View SolutionA triangle and a parallelogram have the same base and the same area. If the sides of the triangle are 26cm, 28cm and 30cm, and the parallelogram stands on the base 28cm, find the height of the parallelogram.
View Solution
