What is the magnitude of the acceleration of the system shown in Fig. 4.3.31 ? Also calculate the tension in the string. Given α=60∘,β=30∘m1=4kg,m2=2kg.
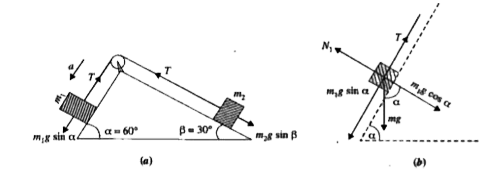
The weight of the mass m1 is resolved into two components, m1gcos∝ balances the normal reaction N and m1gsinα parallel to the plane produces acceleration. Applying Newton.s second law to the mass m1 and m2 separately
m1gsinα−T=m1a ..........(i) N1=m1gcosα
T−m2gsinβ=m2a ........(ii) N2=m2gcosβ
Adding the two equations
m1gsinα−T+T−m2gsinβ=m1a+m2a
a=(m1sinα−m2sinβm1+m2g=(4sin60−2sin306g
=(4×√32−2×12)9.86=4.02m/s2
Dividing equation (i) by equation (ii)
m1gsinα−TT−m2gsinβ=m1m2
m1m2gsinα−m2T=m1T−m1m2gsinβ
F(m1+m2)=m1m2g(sinα+sinβ)
T=m1m2g(sinα+sinβ)m1+m2=4×2×9.8(sin60+sin30)6
=17.8N.
Topper's Solved these Questions
- BOOK - ICSECHAPTER - DYNAMICSEXERCISE - CONCEPTUAL SHORT ANSWER QUESTIONS WITH ANSWERS20videos
DYNAMICS
- BOOK - ICSECHAPTER - DYNAMICSEXERCISE - SHORT ANSWER QUESTIONS93videos
DYNAMICS
- BOOK - ICSECHAPTER - DYNAMICSEXERCISE - SHORT ANSWER QUESTIONS WITH ANSWERS12videos
DYNAMICS
- BOOK - ICSECHAPTER - DIMENSIONSEXERCISE - SELECTED PROBLEMS (FROM CONVERSIONS OF ONE SYSTEMS OF UNITS INTO ANOTHER)9videos
DIMENSIONS
- BOOK - ICSECHAPTER - FRICTIONEXERCISE - Selected problems30videos
FRICTION
Similar Questions
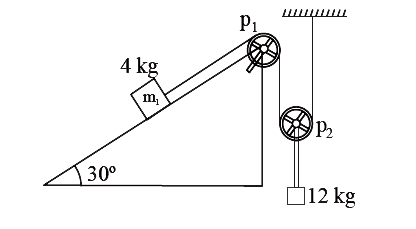
Calculate the acceleration of the masses 12 kg shown in the set up of fig. Also calculate the tension in the string connecting the 12 kg mass. The string are weightless and inextensible, the pulleys are weightless and frictionless.
 View Solution
View SolutionCalculate the acceleration of the masses 12 kg shown in the set up of fig. Also calculate the tension in the string connecting the 12 kg mass. The string are weightless and inextensible, the pulleys are weightless and frictionless.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Calculate the acceleration of the masses 12 kg shown in the set up of fig. Also calculate the tension in the string connecting the 12 kg mass. The string are weightless and inextensible, the pulleys are weightless and frictionless
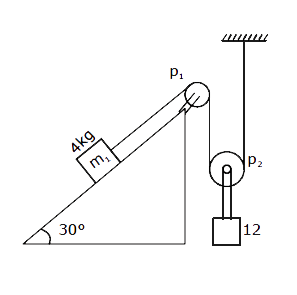 Ag10,60g7NB2g7,60g17NC10g,556gNDg14,556gN
Ag10,60g7NB2g7,60g17NC10g,556gNDg14,556gN - Question 2 - Select One
If m1=10kg,m2=4kg,m3=2kg, the acceleration of system is
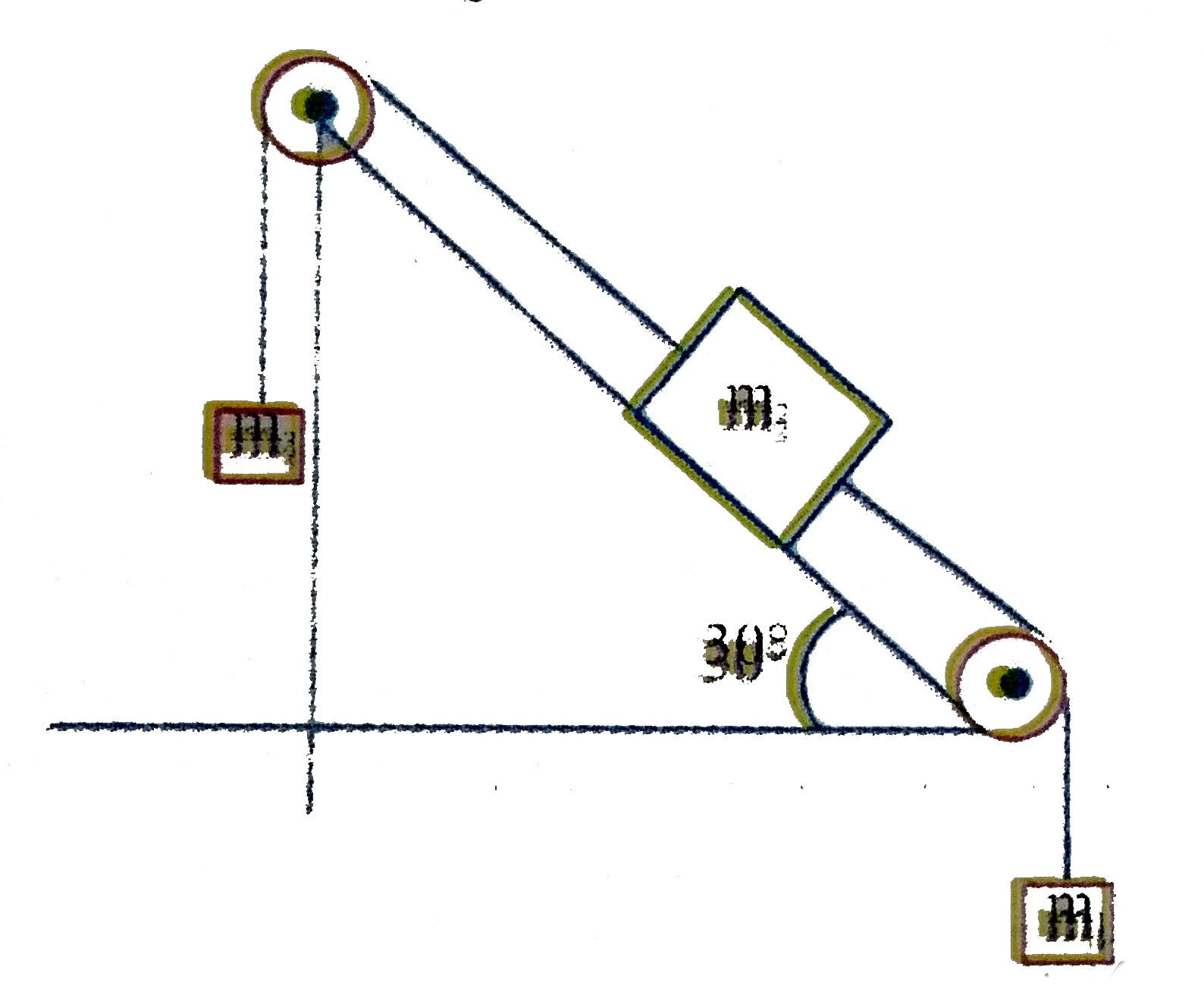 .A5g/2B5g/3C5g/8D5g/14
.A5g/2B5g/3C5g/8D5g/14
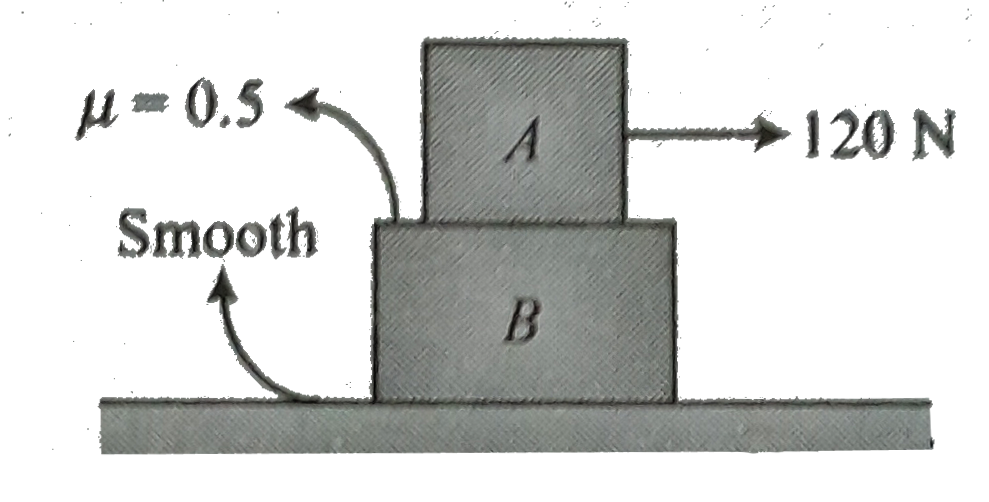
Find the acceleration of the two blocks . The system is initialy at rest and the friction are as shown in fig .Given mA=mB=10kg
 View Solution
View SolutionFind the acceleration of the two blocks . The system is initialy at rest and the friction are as shown in fig .Given mA=mB=10kg
 View Solution
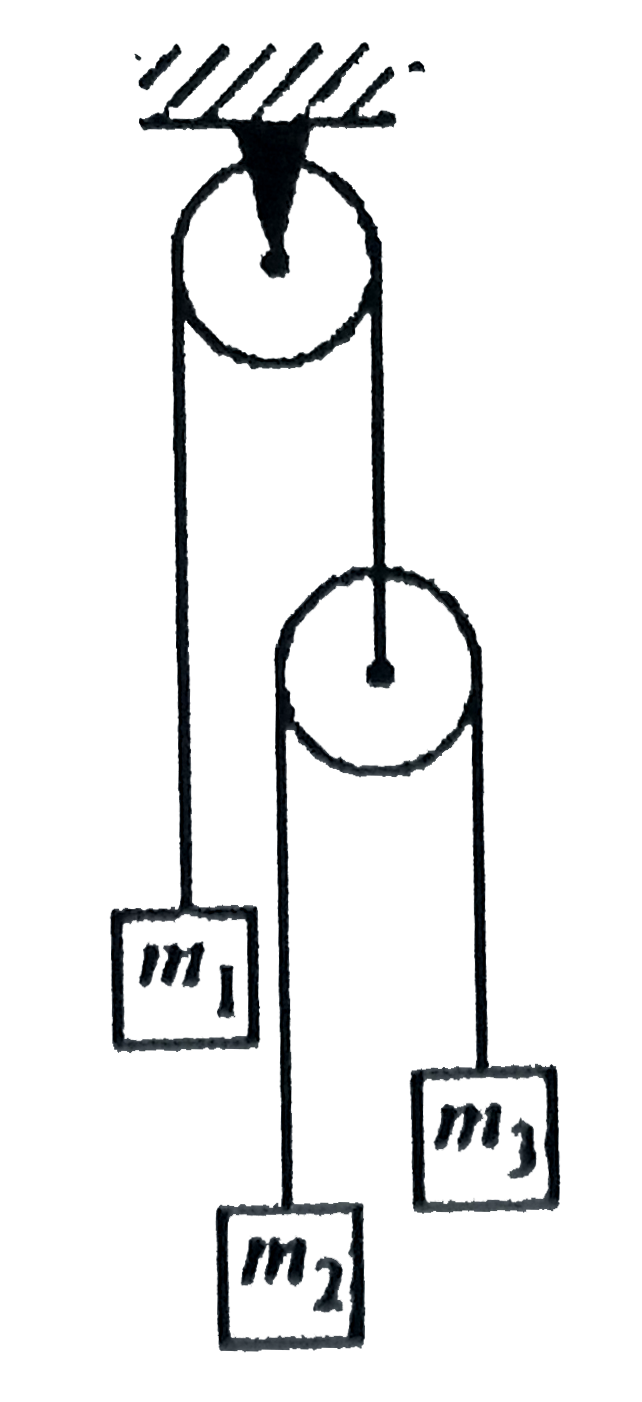
View SolutionConsider the system ofpulleysasshown in figure-2.197. Find the acceleration of the three masses m1,m2 and m3.(m1=Ikg,m2=2kg and m3=3kg)
 View Solution
View SolutionIn the situation shown, the tension in the string is 22N. What is m (in kg) ?
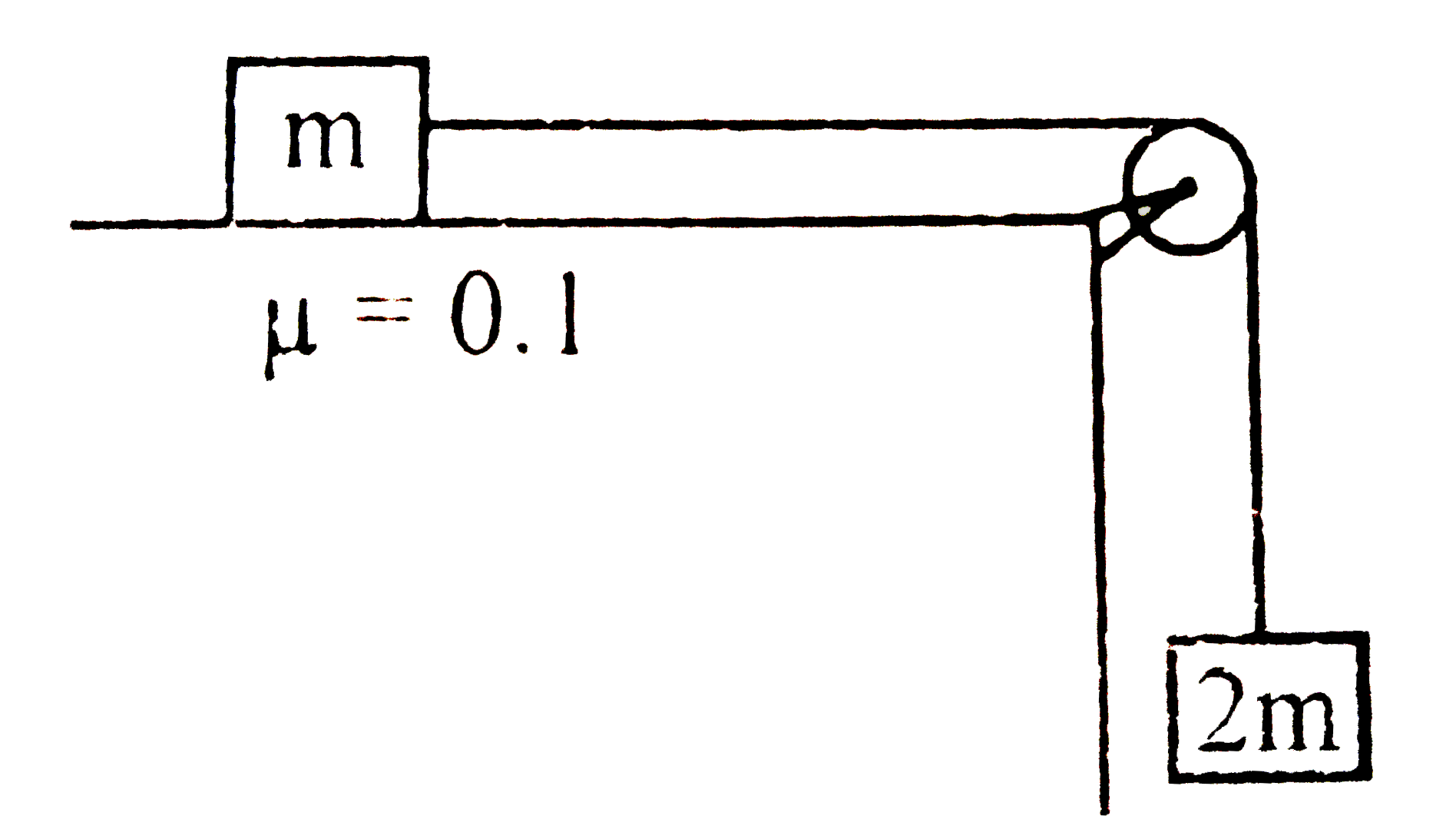 View Solution
View SolutionA block of mass 30 kg is suspended by three string as shown in fig, Find the tension in each string.
 View Solution
View SolutionA block of mass 30 kg is suspended by three string as shown in fig, Find the tension in each string.
 View Solution
View Solution

