ABC is a triangle with sides BC produced to BD. CE is drawn parallel to BA. Find x, y, and z.

The correct Answer is:x=y=77∘,z=55∘
Topper's Solved these Questions
- LINES AND ANGLESBOOK - ICSECHAPTER - LINES AND ANGLESEXERCISE - Revision exercise
20 Videos
- LINES AND ANGLESBOOK - ICSECHAPTER - LINES AND ANGLESEXERCISE - Challenge
2 Videos
- LINES AND ANGLESBOOK - ICSECHAPTER - LINES AND ANGLESEXERCISE - Try this
24 Videos
- LINEAR EQUATIONSBOOK - ICSECHAPTER - LINEAR EQUATIONSEXERCISE - EXERCISE 14B
27 Videos
- MENSURATIONBOOK - ICSECHAPTER - MENSURATIONEXERCISE - EXERCISE 23 G
14 Videos
Similar Questions
In Figure, ABC is an isosceles triangle whose side AC is produced to E . Through C, CD is drawn parallel to BA . The value of x is
(a) 52∘
(b) 76∘
(c) 156∘
(d) 104∘
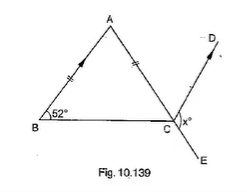
View SolutionIn Figure, the side BC of ABC is produced to form ray BD as shown. Ray CE is drawn parallel to BA . Show directly, without using the angle sum property of a triangle that ∠ACD=∠A+∠B and deduced that ∠A+∠B+∠C=1800.
View Solution
Knowledge Check
ΔABC is an isosceles triangle with AB=AC, side BA is produced to D such that AB=AD. Prove that ∠BCD is a right angle.
A60∘B90∘C120∘Dcan't be determinedΔABC is an isosceles triangle with AB=AC, side BA is produced to D such that AB=AD. Prove that ∠BCD is a right angle.
A60∘B90∘C120∘Dcan't be determinedSmplify:- 38% of ? = 3596 - 632
Aa. 7800Bb. 7900Cc. 8900Dd. 8700
In Figure, the side BC of ABC is produced to form ray BD as shown. Ray CE is drawn parallel to BA . Show directly, without using the angle sum property of a triangle that ACD= ∠A+∠b and deduced that ∠A+∠B+∠C=1800.
View SolutionIf side BC of triangle ABC is produced to P and CE is drawn perpendicular on AC such that angle A: angle B: angle C=3:2:1, find angle ECD
View SolutionIn the adjoining figure, ABC is a triangle in which side BC has been produced to D. If ∠BAC=x∘,∠ABC=(4a)∘,and∠ACD=(5a)∘, find the values of a a and x.

View SolutionLex X by any point on the side BC of a triangle ABC. If XM, XN are drawn parallel to BA and CA meeting CA, BA in M, N respectively; MN meets BC produced in T, prove that TX2=TB x TC
View SolutionIn a right angled triangle ABC, ∠B is right angle, side AB is half of the hypotenuse. AE is parallel to median BD and CE is parallel to BA. What is the ratio of length of BC to that of EC ?
View Solution
