x∈(0,π) के लिये, समीकरण sinx+2sin2x−sin3x=3 के
अनन्त (infinitely many) हल हैं।
तीन (three) हल हैं।
एक (one) हल है।
कोई हल नहीं है (no solution)
Step by step video & image solution for x in (0, pi) के लिये, समीकरण sin x + 2 sin 2x - sin3 x = 3 के by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - JEE ADVANCED पेपर - 2 (2014)EXERCISE - PART III : MATHEMATICS (खण्ड - 2 : अनुच्छेद प्रकार (केवल एक विकल्प सही))6videos
JEE ADVANCED पेपर - 2 (2014)
- BOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - JEE ADVANCED पेपर - 2 (2014)EXERCISE - PART III : MATHEMATICS (खण्ड - 3 : सुमेलन सूची प्रकार (केवल एक विकल्प सही) )4videos
JEE ADVANCED पेपर - 2 (2014)
- BOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - JEE ADVANCED पेपर - 2 (2013)EXERCISE - PART III : MATHEMATICS (खण्ड - 3) (सुमेलन सूची प्रकार)4videos
JEE ADVANCED पेपर - 2 (2013)
- BOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - JEE ADVANCED पेपर - 2 (2015)EXERCISE - भाग III : गणित (खंड - 3)4videos
JEE ADVANCED पेपर - 2 (2015)
Similar Questions
माना कि a, r, s, t शून्येतर वास्तविक संख्यायें (non zero real numbers) हैं, P(at2,2at),Q,R(ar2,2ar) तथा S(as2,2as) परवलय y2=4ax पर स्थित विभिन्न बिन्दु हैं। माना कि PQ नाभीय जीवा (focal chord) है एवम् रेखायें QR तथा PK समानान्तर हैं, जहाँ K बिन्दु (2a,0) है।
r का मान है03:16View Solutionइस खंड में 4 बहुविकल्पीय प्रश्न है। प्रत्येक प्रश्न में दो सुमेलन सूचियां है। सूचियों के लिए कूट के विकल्प और है जिनमें से केवल एक सही है।
माना की zk=cos(2kπ10)+isin(2kπ10),k=1,2,…,9
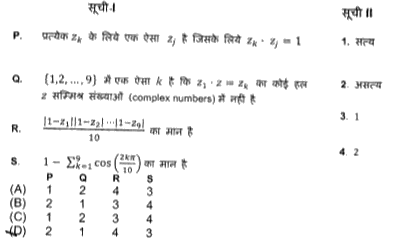 01:58View Solution
01:58View Solutionसूची I को सूची II से सुमेलित कीजिए तथा सूचियों के नीचे दिए गए कोड का प्रयोग करके सही उत्तर चुनिये :
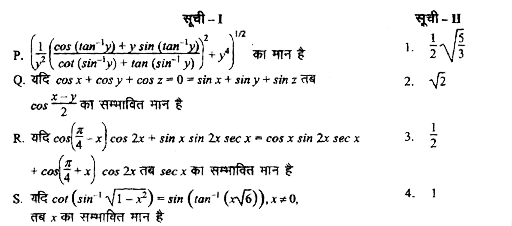 View Solution
View Solutionमाना कि बॉक्स I में n1 लाल गेंद और n2 काली गेंद हैं । माना कि बॉक्स II में n3 लाल गेंद और n4 काली गेंद हैं ।
बॉक्स I और बॉक्स II में से, यादृच्चया (at random) एक बॉक्स को चुना गया और इस चुने हुए बॉक्स से, यादृच्चया एक गेंद निकाली गयी । यह गेंद लाल रंग की पाई गयी । यदि इस लाल गेंद के बॉक्स II से निकाले जाने की प्रायिकता 13 है, तब निम्नलिखित में से n1,n2,n3 और n4 के सही संभव मान है (हैं)05:32View Solutionif 1+sin x+sin^(2)x+sin^(3)x+.........*oo=4+2sqrt(3),0
05:28View SolutionThe number of roots of the equation x^(3)+x^(2)+2x+sin x=0 in (-2 pi,2 pi)
04:11View SolutionThe number of distinct real roots of the equation sin3x+sin2xsinx−sinx−sin2x−2cosx=0 belonging to the interval (−π2,π2)
03:02View SolutionFor x∈(0,π), the equation
sinx+2sin2x−sin3x=3 has06:29View Solutionx के सापेक्ष समाकलन कीजिए :
sinx+cosx3sinx+2cosx03:50View Solutionवक्र y=sin3x के बिंदु π3,34 पर अभिलम्ब का समीकरण ज्ञात कीजिए।
04:24View Solutionयदि 0≤x<π2 है , तो x के उन मानों कि संख्या जिनके लिए sinx−sin2x+sin3x=0 है ,
03:46View Solutionx∈(0,π) के लिए समीकरण sinx+2sin2x−sin3x=3 के
05:06View SolutionThe number of distinct real roots of the equation sin3x+sin2x+sinx−2cosxsin2x−sin2x−2cosx=0 belonging to the interval (−π2,π2) is
View Solutionसमीकरण sin4x+cos4x+sin2x+α=0 ,α के निम्न मान के लिए हल योग्य है।
06:05View Solutionमाना फलन f(x)={xsin(πx),x>0के लिए0 ,x=0के लिए है, तब (0,1) में उन बिन्दुओं की संख्या जहाँ अवकलज f(x) विलुप्त होता है, होगी।
04:17View Solutionमाना f(x)=⎧⎪ ⎪ ⎪⎨⎪ ⎪ ⎪⎩[2(sinx−sin3x)+∣∣sinx−sin3x∣∣2(sinx−sin3x)−∣∣sinx−sin3x∣∣],x≠π2,x∈(0,π) के लिए3x=π2 जहाँ [] महत्तम पूर्णांक फलन हो दर्शाता है, तब
04:40View Solution

