The bulk modulus of a gas is defined as B = -VdP/dV. For an adiabatic process the variation of B is proportional to Pn. For an idea gas, n is
0
1
52
2
The correct Answer is:B
For adiabatic process PVγ = C
γPVγ−1+dPdVVγ=0⇒Pγ=−VdPdV
Hence n = 1
Topper's Solved these Questions
- BOOK - ERRORLESSCHAPTER - THERMODYNAMICSEXERCISE - NCERT BASED QUESTIONS (ISOBARIC AND ISOCHORIC PROCESS)10videos
THERMODYNAMICS
- BOOK - ERRORLESSCHAPTER - THERMODYNAMICSEXERCISE - NCERT BASED QUESTIONS (HEAT ENGINE, REFRIGERATOR AND SECOND LAW OF THERMODYNAMICS)35videos
THERMODYNAMICS
- BOOK - ERRORLESSCHAPTER - THERMODYNAMICSEXERCISE - NCERT BASED QUESTIONS (ISOTHERMAL PROCESS)16videos
THERMODYNAMICS
- BOOK - ERRORLESSCHAPTER - SURFACE TENSIONEXERCISE - ASSERTION & REASON11videos
SURFACE TENSION
- BOOK - ERRORLESSCHAPTER - THERMOMETRY, THERMAL EXPANSION AND CALORIMETRYEXERCISE - ASSERTION & REASON10videos
THERMOMETRY, THERMAL EXPANSION AND CALORIMETRY
Similar Questions
One mole of a gas is in state A[P,V,TA]. A small adiabatic process causes the state of the gas to change to B[P+dP,V+dV,TB]. The changes dV & dP are infinitesimally small and dV is negative. An alternative process takes the gas from state A to B via A→C→B. A→C is isochoric and C→B is isobaric path. State at C is [P+dP,V,TC].
(a) Rank the temperatures TA,TB and TC from highest to lowest.
(b) Find g of the gas in terms of TA, TB and TC.
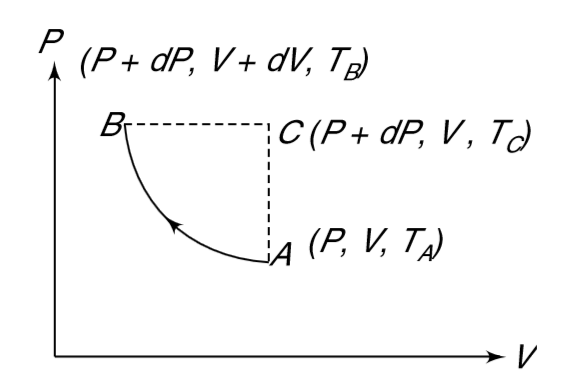 View Solution
View SolutionDuring an adiabatic process, the density of a gas is foung to be proportional to cube of temperature. The degree of freedom of gas molecule is
View Solution
Knowledge Check
- Question 1 - Select One
For adiabatic process of an ideal gas the value of dPP is equals to
A−γdVVB−γVdVCdVVD−γ2dVV - Question 2 - Select One
The isothermal Bulk modulus of an ideal gas at pressure P is
APBλpCp/2DP/λ - Question 3 - Select One
In an adiabatic process, R=23Cv. The pressure of the gas will be proportional to:
AT5/3BT5/2CT5/4DT5/6
The adiabatic Bulk modulus of a perfect gas at pressure is given by
View SolutionAssertion A diabatic bulk modulus of an ideal gas in more than its isothermal bulk modulus.
Reason Both the modulii aer proportional to the pressure of gas at that moment.View SolutionChoose the most appropriate option.
During an adiabatic process, the pressure of a gas is found to be proportional to the cube of its absolute temperature. The ratio of CpCv for the gas isView SolutionThe adiabatic Bulk modulus of a diatomic gas at atmosheric pressure is
View SolutionValue of adiabatic bulk modulus of elasticity of helium at NTP is
View Solution

