A boat crosses a river from port A to port B, which are just on the opposite side. The speed of the water is VW and that of boat is VB relative to still water. Assume VB=2VW. What is the time taken by the boat, if it has to cross the river directly on the AB line
2DVB√3
√3D2VB
DVB√2
D√2VB
The correct Answer is:A
To solve the problem of how long it takes for a boat to cross a river directly from port A to port B, we will follow these steps:
Step 1: Understand the problem
We have a river with a width D (the distance between ports A and B), a boat with speed VB relative to still water, and a current with speed VW. We know that VB=2VW.
Step 2: Set up the scenario
The boat must cross the river directly from A to B. To do this, it must angle its path upstream to counteract the current of the river. We denote the angle at which the boat must head upstream as θ.
Step 3: Analyze the velocities
- The component of the boat's velocity that acts against the current is VBsinθ.
- The component of the boat's velocity that acts across the river (toward B) is VBcosθ.
To ensure the boat moves directly across the river, the upstream component of the boat's speed must equal the downstream speed of the current:
VBsinθ=VW
Step 4: Substitute the relationship between VB and VW
Since VB=2VW, we can substitute this into the equation:
2VWsinθ=VW
Dividing both sides by VW (assuming VW≠0):
2sinθ=1
Thus,
sinθ=12
Step 5: Determine the angle θ
From trigonometry, we know that:
sinθ=12⟹θ=30∘
Step 6: Calculate the effective speed across the river
Now, we need to find the effective speed of the boat across the river:
VBcosθ=2VWcos30∘
Using cos30∘=√32:
VBcosθ=2VW⋅√32=VW√3
Step 7: Calculate the time taken to cross the river
The time T taken to cross the river can be calculated using the formula:
T=DistanceSpeed=DVBcosθ
Substituting the effective speed:
T=DVW√3
Step 8: Substitute VW back in terms of VB
Since VW=VB2:
T=DVB2√3=2DVB√3
Final Answer
Thus, the time taken by the boat to cross the river directly from A to B is:
T=2DVB√3
We have a river with a width D (the distance between ports A and B), a boat with speed VB relative to still water, and a current with speed VW. We know that VB=2VW.
The boat must cross the river directly from A to B. To do this, it must angle its path upstream to counteract the current of the river. We denote the angle at which the boat must head upstream as θ.
- The component of the boat's velocity that acts against the current is VBsinθ.
- The component of the boat's velocity that acts across the river (toward B) is VBcosθ.
VBsinθ=VW
Since VB=2VW, we can substitute this into the equation:
2VWsinθ=VW
Dividing both sides by VW (assuming VW≠0):
2sinθ=1
Thus,
sinθ=12
From trigonometry, we know that:
sinθ=12⟹θ=30∘
Now, we need to find the effective speed of the boat across the river:
VBcosθ=2VWcos30∘
Using cos30∘=√32:
VBcosθ=2VW⋅√32=VW√3
The time T taken to cross the river can be calculated using the formula:
T=DistanceSpeed=DVBcosθ
Substituting the effective speed:
T=DVW√3
Since VW=VB2:
T=DVB2√3=2DVB√3
Thus, the time taken by the boat to cross the river directly from A to B is:
T=2DVB√3
Topper's Solved these Questions
- BOOK - ERRORLESSCHAPTER - MOTION IN ONE DIMENSIONEXERCISE - NCERT BASED QUESTIONS (MOTION UNDER GRAVITY )56videos
MOTION IN ONE DIMENSION
- BOOK - ERRORLESSCHAPTER - MOTION IN ONE DIMENSIONEXERCISE - PAST YEARS QUESTIONS26videos
MOTION IN ONE DIMENSION
- BOOK - ERRORLESSCHAPTER - MOTION IN ONE DIMENSIONEXERCISE - NCERT BASED QUESTIONS (NON-UNIFORM MOTION )104videos
MOTION IN ONE DIMENSION
- BOOK - ERRORLESSCHAPTER - KINETIC THEORY OF GASES EXERCISE - ASSERTION AND REASON16videos
KINETIC THEORY OF GASES
- BOOK - ERRORLESSCHAPTER - MOTION IN TWO DIMENSIONEXERCISE - ASSERTION & REASON19videos
MOTION IN TWO DIMENSION
Similar Questions
For a boat to reach port B from port A, it has to travel 80 km upstream and then 240 km downstream. If the speed of the boat in still water is 45 km/h and the speed of the stream is 5 km/h ,then what is the time taken by the boat to reach port B from port A ?
View SolutionThe velocity of boat relative to water is 4msec . The velocity of water relative to ground is 8msec. In what direction boatman aims the boat to cross the river in minimum drift distance method.
View Solution
Knowledge Check
- Question 1 - Select One
A boat crosses a river of width 1 km by shortest path in 15 min. if the speed of boat in still water is 5 kmh−1, then what is the speed of the river?
A5 kmh−1B12 kmh−1C3 kmh−1D4 kmh−1 - Question 2 - Select One
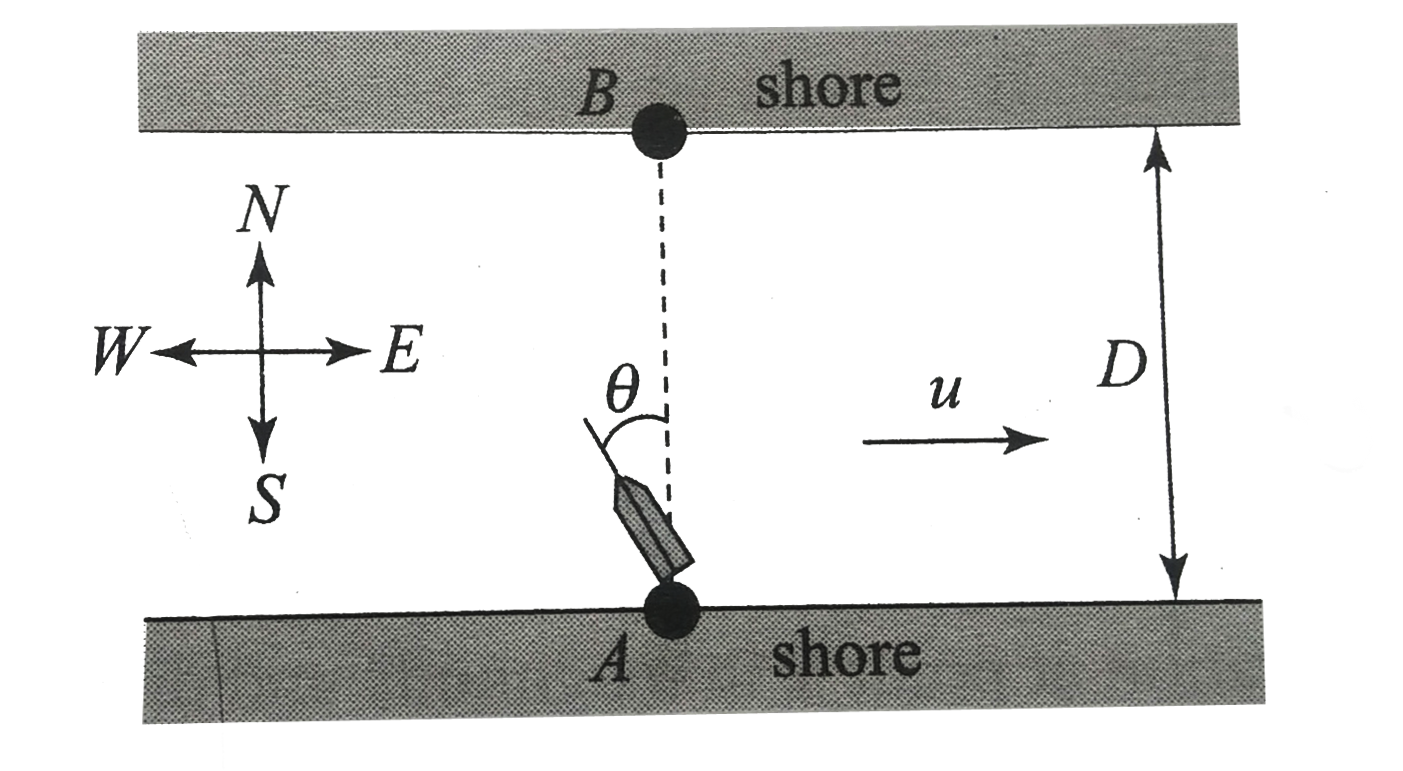
Two ports, A and B, on a north-south line ar separated by a river of width D. The river flows east with speed u. A boat crosses the river starting from port A. The speed of the boat relative to the river is v. Assume v=2u
Q. What is the direction of the velocity of boat relative to river θ, sothat it crosses directly on a line from A to B?A30∘ west of northB30∘ east of northC60∘ west of northD60∘ east of north - Question 3 - Select One

Two ports, A and B, on a north-south line ar separated by a river of width D. The river flows east with speed u. A boat crosses the river starting from port A. The speed of the boat relative to the river is v. Assume v=2u
Q. Suppose the boat wants to cross the river from A to the other side in the shortest possible time. Then what should be the direction of the velocity of boat relative to river?A30∘ west of northB30∘ east of northC60∘ west of northDalong north
(a) In which direction should the motorboat given in Illustration 5.46 head in order to reach a point on the opposite bank directly east from the starting point ? The boat's speed relative to the water remains 4ms−1.
(b) What is the velocity of the boat relative to the earth ?
( c) How much time is required to cross the river ?View SolutionA swimmer starts to swim from point a to cross a river. He wants to reach point B on the opposite side of the river. The line AB makes an angle 60∘ with the river flow as hown. The velocity of the swimmer in still water is same as that of the water

(i) In what direction should he try to direct his velocity ? Calculate angle between his velocity anf river velocity.
(ii) Find the ratio of the time taken to cross the river in this situation to the minimum time in which he can cross this river.View SolutionA river of width ω is flowing with a uniform velocity v. A boat starts moving from point P also With velocity v relative to the river. The direction of resultant velocity is always perpendicular to the line joining boat and the fixed point R. Point Q is on the opposite side of the river. P, Q and R are in a straight line. If PQ=QR=ω, find (a) the trajectory of the boat, (b) the drifting of the boat and (c) the time taken by the boat to cross the river.
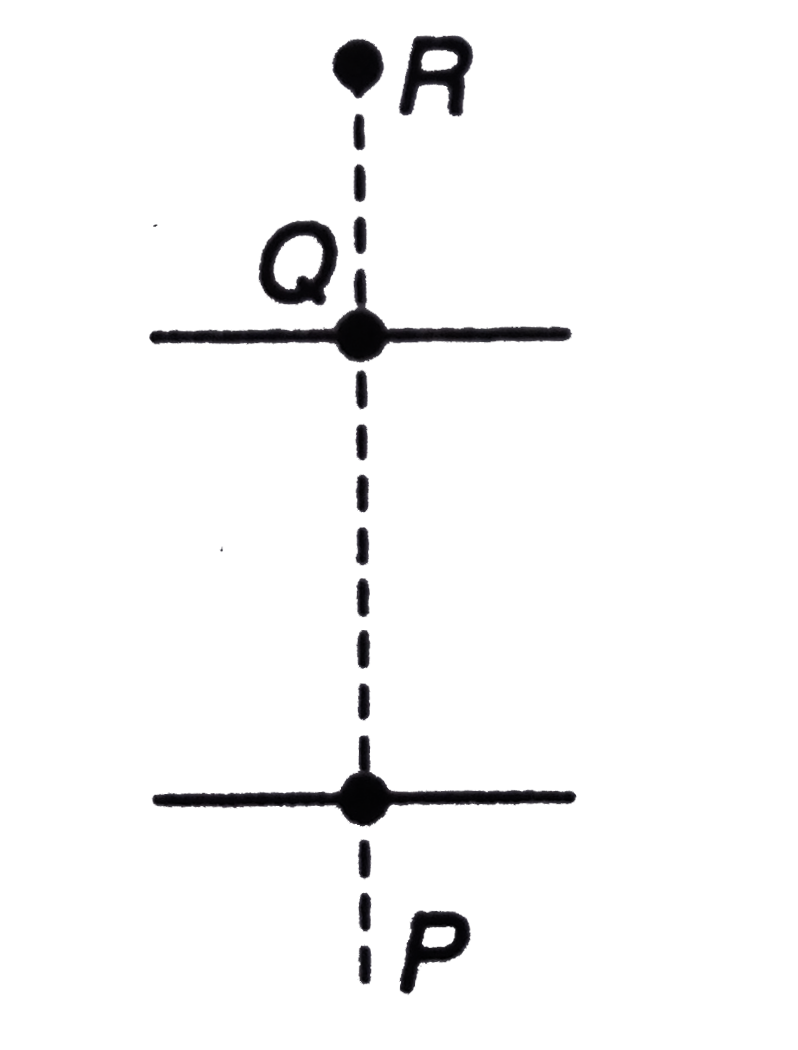 View Solution
View SolutionA man in a row boat must get from point A to point B on the opposite bank of the river (see figure).The distance BC=a.The width of the river AC=b.At what minimum speed u relative to the still water should the boat travel to reach the point B?The velocity of flow of the river is v0
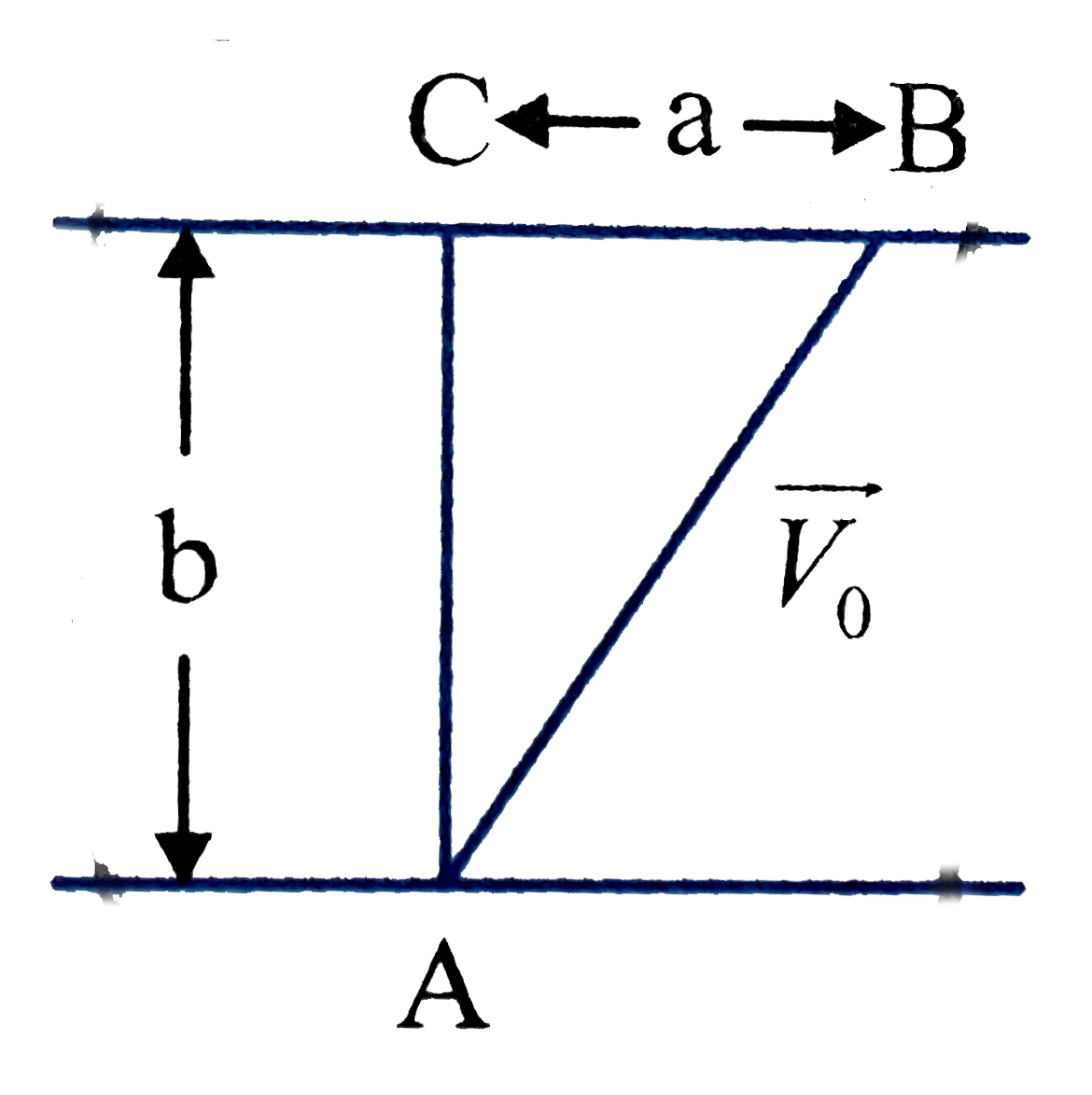 View Solution
View Solution
Two ports, A and B, on a north-south line ar separated by a river of width D. The river flows east with speed u. A boat crosses the river starting from port A. The speed of the boat relative to the river is v. Assume v=2u
Q. The boat crosses the river from A to the other side in shortest possible time, then how far is the boat from the port B after crossing the riverView Solution

