A particle is moving along the curve →r(t)=cost¯i+sint¯j+t¯k , starting at t = 0. Then its velocity and speed at time t=0 are given by
¯j,√2
¯j,√2
−¯j+¯k,√2
¯j+¯k,√2
To find the velocity and speed of the particle moving along the curve given by the position vector →r(t)=cos(t)^i+sin(t)^j+t^k at time t=0, we can follow these steps:
Step 1: Differentiate the Position Vector
To find the velocity →v(t), we need to differentiate the position vector →r(t) with respect to time t.
→v(t)=d→r(t)dt=ddt(cos(t)^i+sin(t)^j+t^k)
Step 2: Apply the Derivative
Now we apply the derivative to each component of the position vector:
→v(t)=−sin(t)^i+cos(t)^j+1^k
Step 3: Evaluate the Velocity at t=0
Next, we substitute t=0 into the velocity vector:
→v(0)=−sin(0)^i+cos(0)^j+1^k
Since sin(0)=0 and cos(0)=1:
→v(0)=0^i+1^j+1^k=^j+^k
Step 4: Calculate the Speed
The speed is the magnitude of the velocity vector:
Speed=|→v(0)|=√(0)2+(1)2+(1)2=√0+1+1=√2
Final Result
Thus, the velocity at t=0 is ^j+^k and the speed is √2.
Summary
- Velocity at t=0: ^j+^k
- Speed at t=0: √2
To find the velocity →v(t), we need to differentiate the position vector →r(t) with respect to time t.
Now we apply the derivative to each component of the position vector:
Next, we substitute t=0 into the velocity vector:
The speed is the magnitude of the velocity vector:
Thus, the velocity at t=0 is ^j+^k and the speed is √2.
- Velocity at t=0: ^j+^k
- Speed at t=0: √2
Topper's Solved these Questions
Similar Questions
A particle travels so that its acceleration is given by →a=5costˆi−3sintˆj. If the particle is located at (−3,2) at time t=0 and is moving with a velocity given by (−3ˆi+2ˆj). Find
(i) The velocity [→v=∫→a.dt] at time t and
(ii) The position vector [→r=∫→v.dt] of the particle at time t(t>0).
View SolutionThe acceleration-time graph of a particle moving along x-axis is shown in the figure. If the particle starts with velocity 3 m/s at t = 0, find the velocity of particle at t = 4 s.

View Solution
Knowledge Check
The acceleration versus time graph for a particle moving along a straight line is shown in the figure. If the particle starts from rest at t = 0, then its speed at t = 30 sec will be :
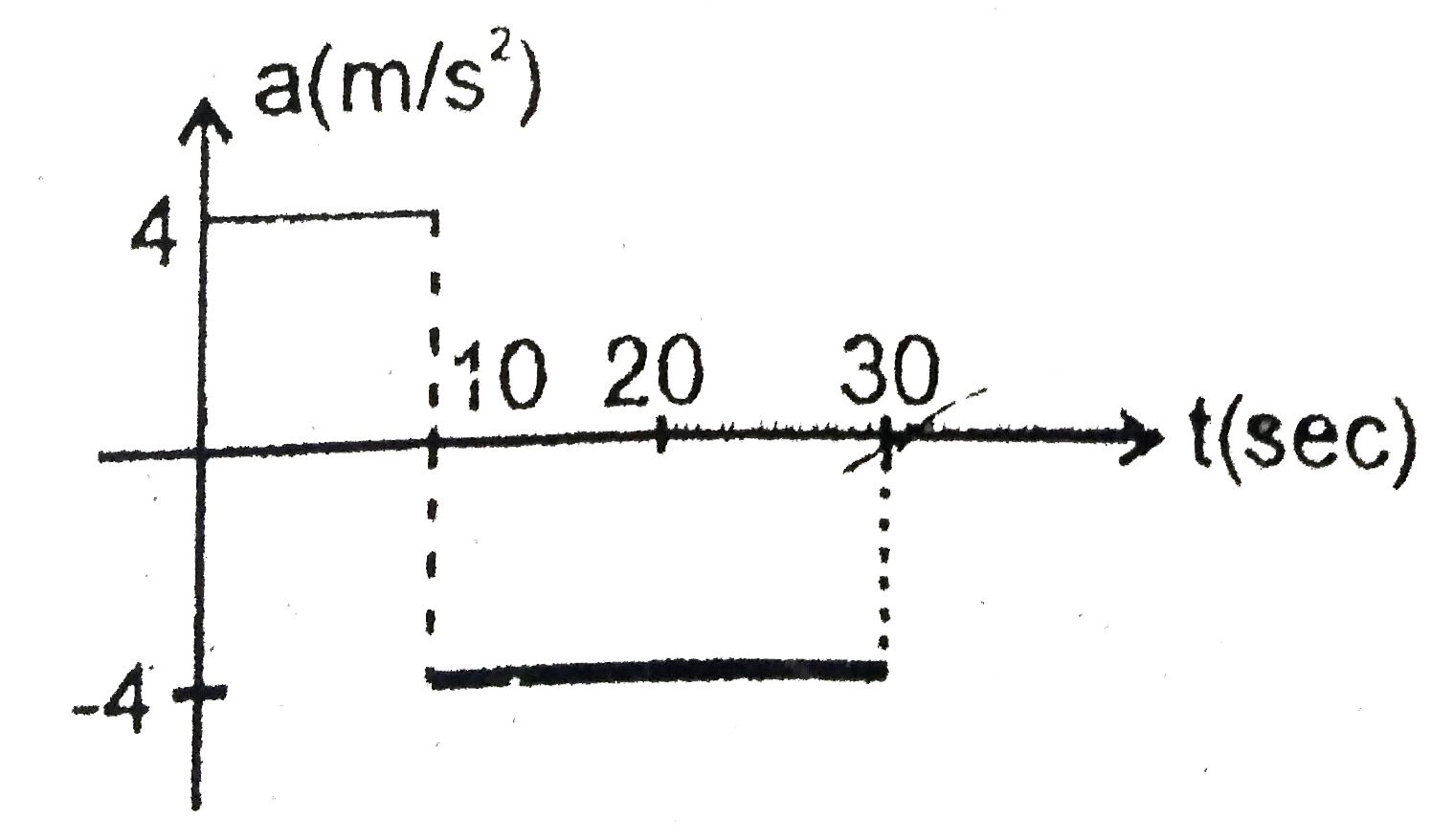 A20m/secB40m/secC0m/secD−40m/sec
A20m/secB40m/secC0m/secD−40m/secIf a particle is moving the velocity v(t)=cos πt, along a straight line such that at t=0, s=4, its position function is given by
A1πcosπt+2B−1πsinπt+4C1πsinπt+4Dnone of theseA particle is constrained to move along the curve y=√x starting at the origin at time t=0. The point on the curve where the abscissa and the ordinate are changing at the same rate is
A(12,1√2)B(18,12√2)C(14,12)D(1,1)
A particle is moving along x-axis with acceleration a=a0(1−t/T) where a0 and T are constants. The particle at t=0 has zero velocity. Calculate the average velocity between t=0 and the instant when a=0
View SolutionThe acceleration of a particle is given by a = 3t and at t = 0, v = 0, x = 0. The velocity and displacement at t = 2 sec will be-
View SolutionA particle is moving with a position vector, →r=[a0sin(2πt)ˆi+a0cos(2πt)ˆj]. Then -
View SolutionThe position vector of a particle is →r=acosωti+asinωtj, the velocity of the particle is
View SolutionThe coordinates of a moving particle at any time t are given by x = ct and y = bt. The speed of the particle at time t is given by
View Solution
