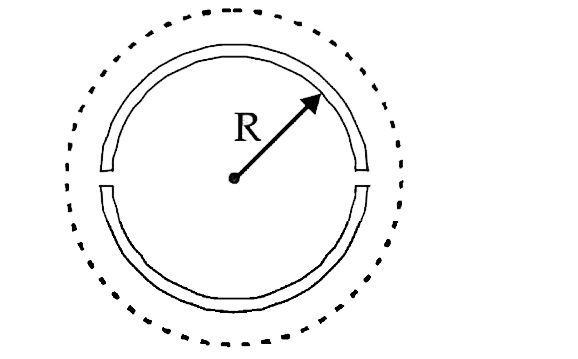
A wooden wheel of radius R is made of two semicircular part . The two parts are held together by a ring made of a metal strip of cross sectional area S and length L. L is slightly less than 2πR. To fit the ring on the wheel, it is heated so that its temperature rises by ΔTand it just steps over the wheel. As it cools down to surrounding temperature, it process the semicircle parts together. If the coefficient of linear expansion of the metal is α, and it Young's modulus is Y, the force that one part of the wheel applies on the other part is :
2πSYαΔT
SYαΔT
πSYαΔT
2SYαΔT
The correct Answer is:D
ΔL=LαΔTorΔLL=αΔT
Stress =TS=YΔLL=YαΔT
T=SYαΔT
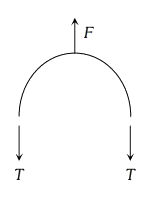
F=2SYαΔT
Similar Questions
A metallic ring of radius r and cross-sectional area A is fitted into a wooden circular disc of radius R(R>r). If the Young's modulus of the material of the ring is Y, the force with which the metal ring expands is
View SolutionStatements given below are incorrect. Write the correct statements. An iron rim of a slightly smaller diameter than a wooden wheel is cooled so that it easily slips over wooden wheel.
View SolutionIron rim is made slightly smaller than the wooden wheel. The rim is usually heated before fixing into the wooden wheel, because on heating the iron rim
View SolutionMetal rim is slightly ....... than wooden wheel.
View SolutionMetal rim is slightly ....... than wooden wheel.
View SolutionA wooden wheel of radius R is made of two semicircular part . The two parts are held together by a ring made of a metal strip of cross sectional area S and length L. L is slightly less than 2piR . To fit the ring on the wheel, it is heated so that its temperature rises by DeltaT and it just steps over the wheel. As it cools down to surrounding temperature, it process the semicircle parts together. If the coefficient of linear expansion of the metal is alpha , and it Young's modulus is Y, the force that one part of the wheel applies on the other part is :
View SolutionWhy is iron rim made slightly smaller than the wooden wheel?
View SolutionA blacksmith fixes iron rings on the rim of the wooden wheel of a bullock cart .The diameter of the rim the iron rings are 6.264 m and 6.253 m respectively at 27^(@)C . To what temperature should the ring be heated so as to fit the rim of the wheel ? (Given alpha_(L)=1.20xx10^(-5)""^(@)C^(-1))
View Solution
