Euclid's axiom 1 s
Things which are equal to the same thing are equal to one another.
If equals are added to equals, the whols are equal
Given two distinct points, there is a unique line that passes through them.
None of these
The correct Answer is:A
Topper's Solved these Questions
- INTRODUCTION TO EUCLID'S GEOMETRYBook:MTG IIT JEE FOUNDATIONChapter:INTRODUCTION TO EUCLID'S GEOMETRYExercise:EXERCISE (MULTIPLE CHOICE QUESTIONS LEVEL -2)
Explore 5 Videos
- INTRODUCTION TO EUCLID'S GEOMETRYBook:MTG IIT JEE FOUNDATIONChapter:INTRODUCTION TO EUCLID'S GEOMETRYExercise:EXERCISE (MULTIPLE CHOICE QUESTIONS LEVEL -2 MATCH THE FOLLOWING)
Explore 3 Videos
- INTRODUCTION TO EUCLID'S GEOMETRYBook:MTG IIT JEE FOUNDATIONChapter:INTRODUCTION TO EUCLID'S GEOMETRYExercise:NCERT SECTION( EXERCISE 5.2)
Explore 2 Videos
- HERON'S FORMULABook:MTG IIT JEE FOUNDATIONChapter:HERON'S FORMULAExercise:OLYMPIAD/HOTS CORNER
Explore 7 Videos
- LINEAR EQUATIONS IN TWO VARIABLES Book:MTG IIT JEE FOUNDATIONChapter:LINEAR EQUATIONS IN TWO VARIABLES Exercise:OLYMPIAD/HOTS CORNER
Explore 5 Videos
Similar Questions
Knowledge Check
It is known that if x+y=10, then x+y+z=10+z. The Euclid's axiom that illustrates this statement is
Afirst axiomBsecond axiomCthird axiomDfourth axiom
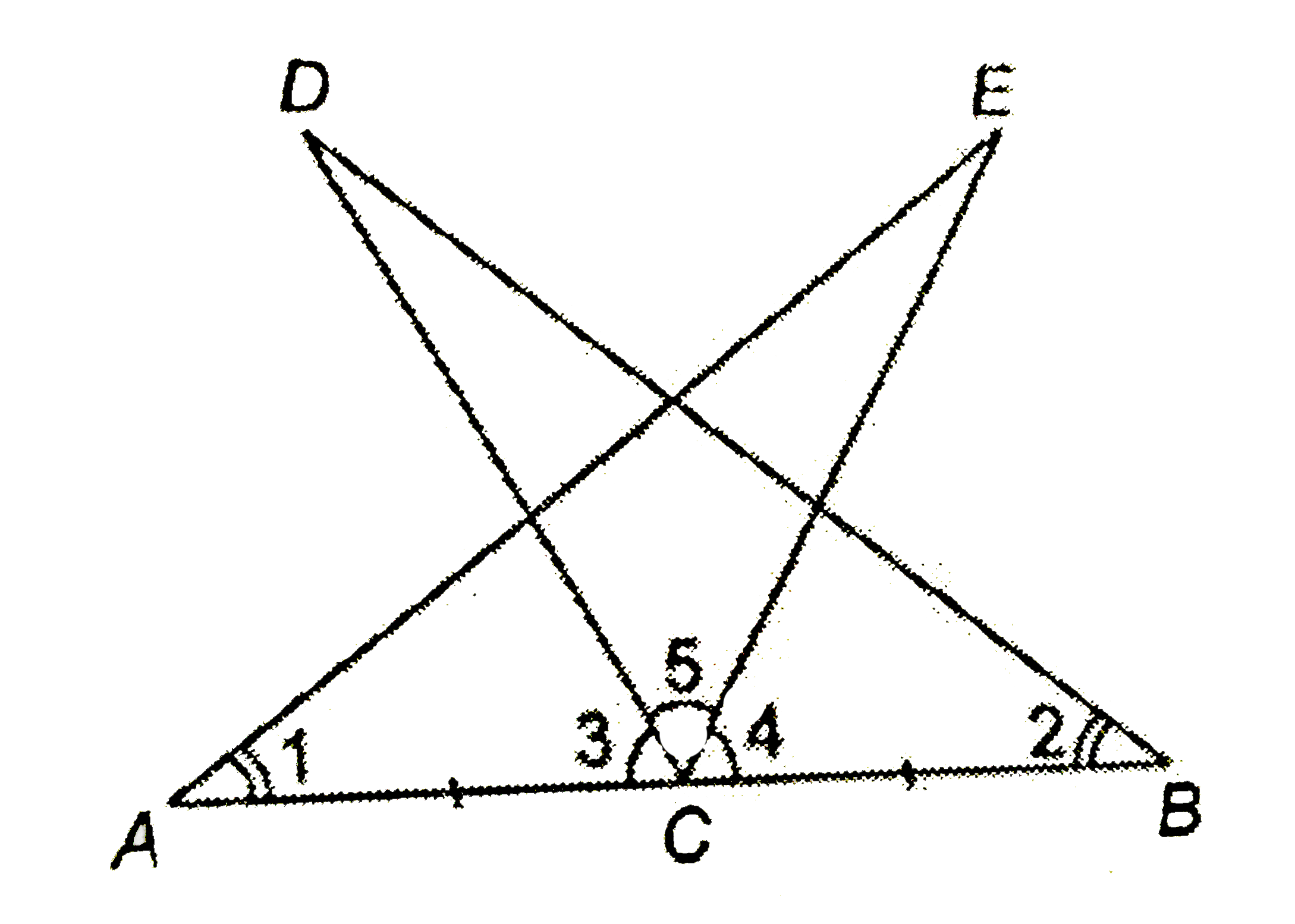
Use the information marked to prove :
(i) ΔEAC≅ΔDBC (ii) EC=DC
Also state the Euclid's axiom used in proving the congruency of two triangles.
View SolutionIntroduction and Euclid's Axioms
View SolutionIt is known that if x+y=10, then x+y+z=10+z. The Euclid's axiom that illustrates this statement is
View SolutionIn the given figure C is the midpoint of AB,D is the midpoint of XY and AC=XD Using an Euclid 's axiom prove that AB =XY
View SolutionAxioms And Postulates|Euclid's Axioms|Euclid's Postulates|Theorem
View SolutionAxioms And Postulates|Axioms, Postulates And Theorems|Euclid's Axioms|NCERT Examples
View SolutionEuclid's Axioms|Euclid's Postulates|Theorem|NCERT Exercise
View Solution
