For every line l and for every point P (not on l), there exist a unique line through P
Which is parallel to l.
Which is not perpendicular to l.
Which is coincident with.
None of these
The correct Answer is:A
Topper's Solved these Questions
- INTRODUCTION TO EUCLID'S GEOMETRYBOOK - MTG IIT JEE FOUNDATIONCHAPTER - INTRODUCTION TO EUCLID'S GEOMETRYEXERCISE - EXERCISE (MULTIPLE CHOICE QUESTIONS LEVEL -2 MATCH THE FOLLOWING)
3 Videos
- INTRODUCTION TO EUCLID'S GEOMETRYBOOK - MTG IIT JEE FOUNDATIONCHAPTER - INTRODUCTION TO EUCLID'S GEOMETRYEXERCISE - EXERCISE (MULTIPLE CHOICE QUESTIONS LEVEL -2 ASSERTION AND REASON TYPE)
2 Videos
- INTRODUCTION TO EUCLID'S GEOMETRYBOOK - MTG IIT JEE FOUNDATIONCHAPTER - INTRODUCTION TO EUCLID'S GEOMETRYEXERCISE - EXERCISE (MULTIPLE CHOICE QUESTIONS LEVEL -1)
15 Videos
- HERON'S FORMULABOOK - MTG IIT JEE FOUNDATIONCHAPTER - HERON'S FORMULAEXERCISE - OLYMPIAD/HOTS CORNER
7 Videos
- LINEAR EQUATIONS IN TWO VARIABLES BOOK - MTG IIT JEE FOUNDATIONCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - OLYMPIAD/HOTS CORNER
5 Videos
Similar Questions
"For every line L and for every point P not lying on a given line L, there exists a unique line m passing through P and parallel to L" is known as playfair 's axiom.
View Solution"For every line L and for every point P not lying on a given line L, there exists a unique line m passing through P and parallel to L" is known as playfair 's axiom.
View Solution
Knowledge Check
Following are the steps while constructing a perpendicular to a line 'l' through a point 'P' not on it. Out of these one of the steps is incorrect. Select the incorrect step.
Step-1: Given a line l and a point P not on it.
Step-2 : With P as centre, draw an arc which intersects line l at two points L and M.
Step-3 : With any convenient radius and with L and Mas centres, construct two arcs that intersect at a point, say Q, on the other side.
Step-4 : Join PQ.AStep-1BStep-2CStep-3DStep-4Every point on a number line represents.
Aa rational numberBa natural numberCan irrational numberDa unique real number
Let L1:x+y−3=0 and L2:7x−y+5=0 be two given lines. Let image of every point on L1, with respect to a line L lies on L2 then possible equation of L can be :
View SolutionIf B is the image of the point A with respect to the line l and P is any point lying on l, then the lengths of line segments PA and PB are _______.
View SolutionDraw a line l. Take any point A outside the line. Draw a line through A, parallel to line l.
View SolutionTo construct a line parallel to a given line and passing through a given point outside the line.
Question : Draw a line l, take a point P outside it. Draw a line m || line l passing through point P.
View SolutionDraw any line 'l'. Now take any point P not lying on it. Construct a line perpendicular to l and passing through point P using ruler and compasses.
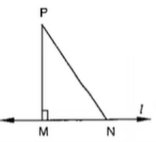
View SolutionOf all the line segments that can be drawn to a given line, from a point, not lying on it, the perpendicular line segment is the shortest. GIVEN : A straight line l and a point P not lying on l. PM⊥l and N is any point on 1 other than M. TO PROVE : PM<PN
View Solution
