In the given figure, if O is the centre of two concentric circles with OA =6 cm, OB =3 cm and AP= 10 cm, then BP =______ 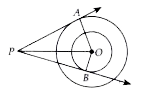
√91cm
√127cm
√119cm
√109cm
The correct Answer is:B
Topper's Solved these Questions
- CIRCLESBOOK - MTG IIT JEE FOUNDATIONCHAPTER - CIRCLESEXERCISE - Exercise (Comprehension (Passage-III))
4 Videos
- ARITHMETIC PROGRESSIONS BOOK - MTG IIT JEE FOUNDATIONCHAPTER - ARITHMETIC PROGRESSIONS EXERCISE - OLYMPIAD/HOTS CORNER
15 Videos
- CO-ORDINATE GEOMETRYBOOK - MTG IIT JEE FOUNDATIONCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - Olympiad/HOTS Corner
40 Videos
Similar Questions
In the given figure O is the centre of the circle. If AB = 16 cm, CP = 6 cm, PD = 8 cm and AP > PB then what will be the value of AP.

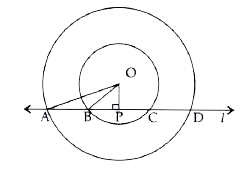
View SolutionIn the given figure o is the centre of the two concentric circles. A line T cuts the circles at A, B, C and D as shown in the figure. OP is perpendicular to AD.
Given OA = 34 cm, OP = 16 cm and AB = 18 cm Find :
radius of the smaller circle
View Solution
Knowledge Check
In the given figure, O is the centre of the circle. AB is tangent. AB=12cmandOB=13cm. Find OA:
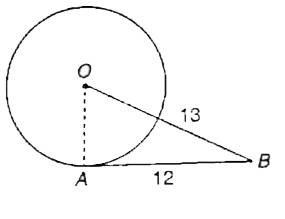 A6.5 cmB6 cmC5 cmDnone of these
A6.5 cmB6 cmC5 cmDnone of theseIn figure , if AP = 10 cm, then BP
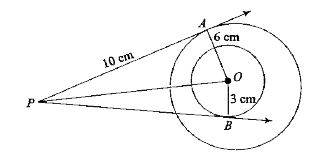 A√91 cmB√127cmC√119 cmD√109 cm
A√91 cmB√127cmC√119 cmD√109 cmIn the adjoining figure, O is the centre of circle and diameter AC=26cm. If chord AB=10cm, then the distance between chord AB and centre O of the circle is :
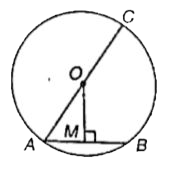 A24 cmB16 cmC12 cmDnone of the above
A24 cmB16 cmC12 cmDnone of the above
In the given figure o is the centre of the two concentric circles. A line T cuts the circles at A, B, C and D as shown in the figure. OP is perpendicular to AD.
Given OA = 34 cm, OP = 16 cm and AB = 18 cm Find :
length of chord BC

View SolutionIn the given figure o is the centre of the two concentric circles. A line T cuts the circles at A, B, C and D as shown in the figure. OP is perpendicular to AD.
Given OA = 34 cm, OP = 16 cm and AB = 18 cm Find :
length of chord AD

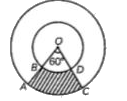
View SolutionIn the figure, the radii of two concentric circles with centre O are 7 cm and 14 cm and ∠AOC=60∘. Find the area of the shaded region.
View SolutionIn the figure, ACDB is a chord cutting two concentric circles with centre O. Find AB if CD = 8 cm, OD = 5 cm, and OB = 9 cm.
View SolutionIn the given figure, O is the centre of the circle. Radius of the circle is 17 cm. lf OC=8cm, then the length of the chord AB is :
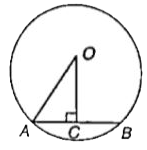
View Solution
