In the given fig, quadrilateral ABCD is circumscribed touching the circle at P, Q, R and S. If AP= 6 cm, BP= 5 cm, CQ= 3 cm and DR =4 cm, then perimeter of quadrilateral ABCD is 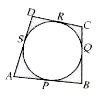
A
18cm
B
27cm
C
36cm
D
22cm
Text Solution
The correct Answer is:A
|
Topper's Solved these Questions
- CIRCLESBook:MTG IIT JEE FOUNDATIONChapter:CIRCLESExercise:Exercise (LEVEL -2)
Explore 20 Videos
- CIRCLESBook:MTG IIT JEE FOUNDATIONChapter:CIRCLESExercise:Exercise (Match the following)
Explore 6 Videos
- CIRCLESBook:MTG IIT JEE FOUNDATIONChapter:CIRCLESExercise:NCERT Section (Exercise 10.2)
Explore 16 Videos
- ARITHMETIC PROGRESSIONS Book:MTG IIT JEE FOUNDATIONChapter:ARITHMETIC PROGRESSIONS Exercise:OLYMPIAD/HOTS CORNER
Explore 15 Videos
- CO-ORDINATE GEOMETRYBook:MTG IIT JEE FOUNDATIONChapter:CO-ORDINATE GEOMETRYExercise:Olympiad/HOTS Corner
Explore 40 Videos
Similar Questions
Knowledge Check
In the given figure, quadrilateral ABCD is circumscribed, touching the circle at P, Q, R and S. If AP = 6 cm, BP = 5 cm, CQ = 3 cm and DR = 4 cm, then perimeter of quadrilateral ABCD is
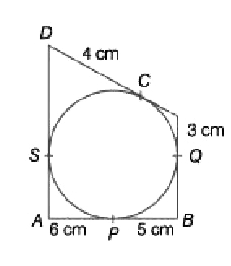 A18 cmB27 cmC36 cmD32 cm
A18 cmB27 cmC36 cmD32 cmQuadrilateral ABCD is circumscribed to a circle. If AB=6cm, BC=7cm and CD=4cm then the length of AD is
A3cmB4cmC6cmD7cmIf the sides of a quadrilateral ABCD touch a circle and AB = 6 cm, CD = 5 cm, BC = 7 cm, then the length of AD in cm is :
A4 cmB5 cmC3 cmD6 cm
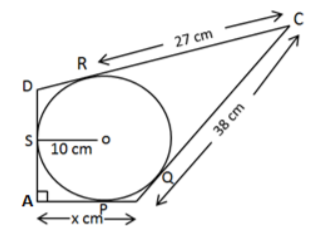
In the given figure quadrilateral ABCD is circumscribed and AD⊥DC. Find x if radius of circle is 10 cm.
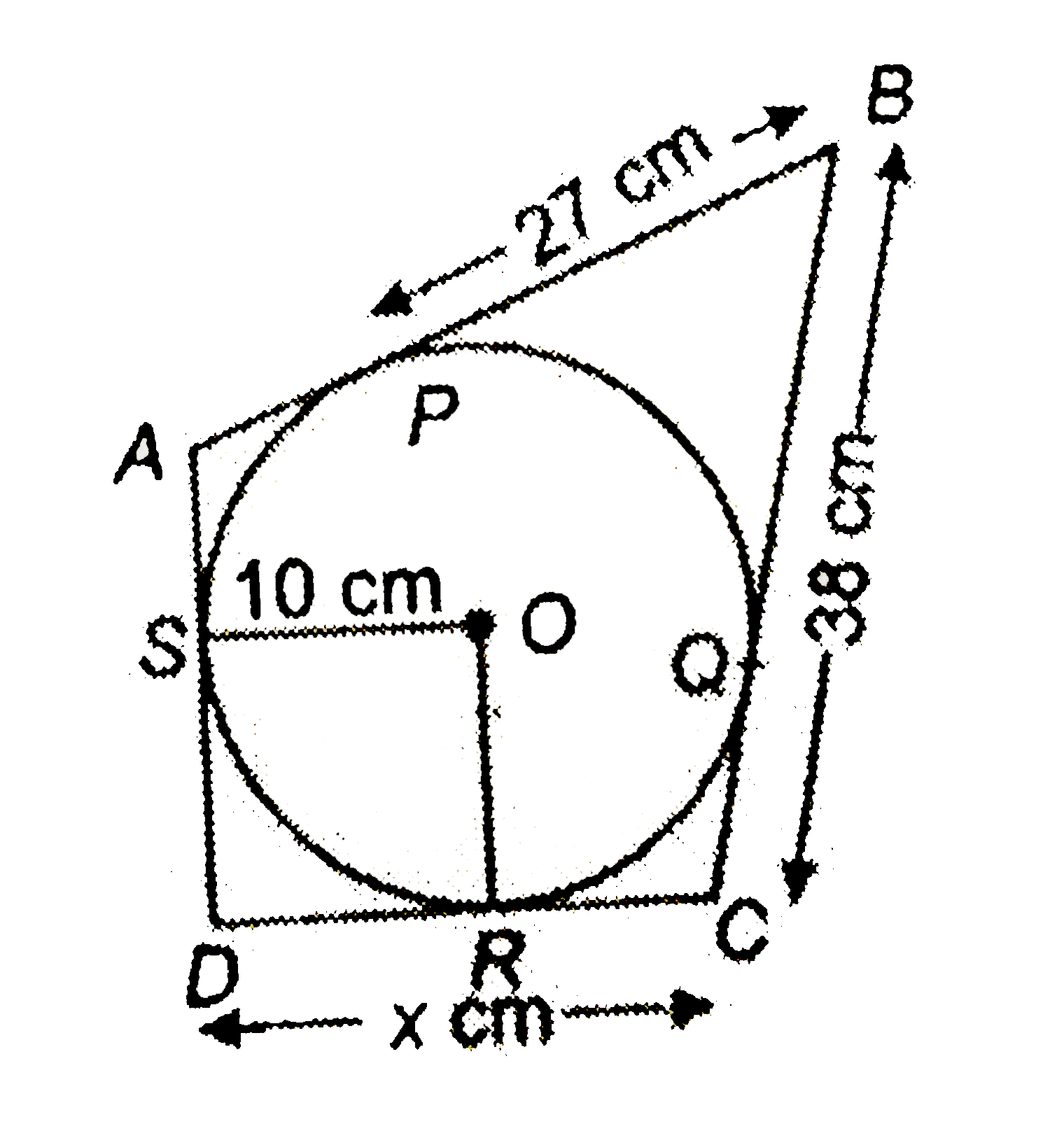
View SolutionIn the figure, quadrilateral ABCD is circumscribing a circle with centre O and AD⊥AB. If radius of incircle is 10cm, then the value of x is
View SolutionFind the perimeter and area of the quadrilateral ABCD in which AB=21cm
View SolutionIn figure, a quadrilateral ABCD is drawn to circumscribe a circle such that its sides AB, BC, CD and AD touch the circle at P, Q, R and S respectively. If AB = x cm, BC = 7 cm, CR = 3cm and AS = 5cm, then x = ______.

View SolutionQuadrilateral ABCD circumscribes a circle. If AB = 8 cm, BC = 7 cm and CD = 6 cm, then the length of AD is:
View Solution
