In figure, two circles with centres A and B touch each other externally. PM= 15 cm is tangent to circle with centre A and QN= 12 cm is tangent to circle with centre B from external point Q. If PA= 17 cm and BQ= 13 cm, then the distance between the centres A and B of circles is 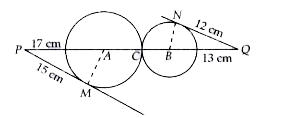
A
12cm
B
11cm
C
13cm
D
14cm
Text Solution
The correct Answer is:C
|
Topper's Solved these Questions
- CIRCLESBOOK - MTG IIT JEE FOUNDATIONCHAPTER - CIRCLESEXERCISE - Exercise (Match the following)
6 Videos
- CIRCLESBOOK - MTG IIT JEE FOUNDATIONCHAPTER - CIRCLESEXERCISE - Exercise (Assertion & Reason)
6 Videos
- CIRCLESBOOK - MTG IIT JEE FOUNDATIONCHAPTER - CIRCLESEXERCISE - Exercise (LEVEL -1)
60 Videos
- ARITHMETIC PROGRESSIONS BOOK - MTG IIT JEE FOUNDATIONCHAPTER - ARITHMETIC PROGRESSIONS EXERCISE - OLYMPIAD/HOTS CORNER
15 Videos
- CO-ORDINATE GEOMETRYBOOK - MTG IIT JEE FOUNDATIONCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - Olympiad/HOTS Corner
40 Videos
Similar Questions
Knowledge Check
If two circles with diameter 8cm and 6 cm touch externally, then the distance between their centres is ______.
A2 cmB7 cmC10 cmD14 cmTwo circles of radii 13 cm and 5 cm touch internally each other. Find the distance between their centres:
A18 cmB12 cmC9 cmD8 cmTwo circles touch externally. The sum of their areas is 130 πsq.cm. and the distance between their centres is 14cm. Find the radii of the circles.
A11 cm, 3 cmB14 cm, 5 cmC13 cm, 9 cmDNone of these
Two circles of radii 4cm and 14cm have a common external tangent of length 24cm .Find the distance between the centres of these circles (in cm ).
View SolutionDraw circles with centres A,B and C each of radius 3 cm, such that each circle touches the other two circles.
View SolutionIf two circles with radii 8 cm and 3 cm respectively touch externally, then find the distance between their centres.
View SolutionThe length of the tangent from an external point P to a circle of radius 5cm is 10cm. The distacne of the point from the centre of the circle is
View SolutionTwo circles of radii 13 cm and 5 cm touch internally each other. Find the distance between their centres:
View Solution
