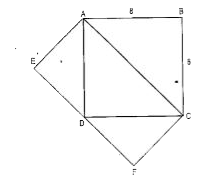
Two rectangles ABCD and DBEF are as shown in the figure. The area of rectangle DBEF (in square units) is _____.
Text Solution
The correct Answer is:12
|
Topper's Solved these Questions
- FOOTSTEPS TOWARDS JEE MAINBOOK - MTG IIT JEE FOUNDATIONCHAPTER - FOOTSTEPS TOWARDS JEE MAINEXERCISE - Section B (Numerical Value Type Questions)
9 Videos
- FOOTSTEPS TOWARDS CBSE BOARDBOOK - MTG IIT JEE FOUNDATIONCHAPTER - FOOTSTEPS TOWARDS CBSE BOARDEXERCISE - PART-B (Section-V)
2 Videos
- INTRODUCTION TO GRAPHS BOOK - MTG IIT JEE FOUNDATIONCHAPTER - INTRODUCTION TO GRAPHS EXERCISE - OLYMPIAD/HOTS CORNER
5 Videos
Similar Questions
Knowledge Check
ABCD is a rectangle of dimensions 8 units and 6 units AEFC is a rectangle drawn in such a way that diagonal AC of the first rectangle is one side and side opposite to it is touching the first rectangle at D as shown in the figure. What is the ratio of the area of rectangle ABCD to that of AEFC ?
 A2B3/2C1D8/9
A2B3/2C1D8/9AC, a diagnonal of the rectange ABCD , measure 5 units. The area of the rectangle is 12 sq. units. What is the perimeter of the rectangle?
A7B14C17D20In the figure below, all of the small square are equal in area, and the area of rectangle ABCD is 1 square unit. Which of the following expressions represents the area, in square units, of the shaded region?
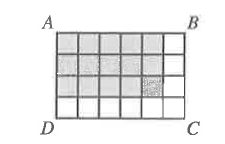 A16⋅14B16⋅34C16⋅56D56⋅34
A16⋅14B16⋅34C16⋅56D56⋅34
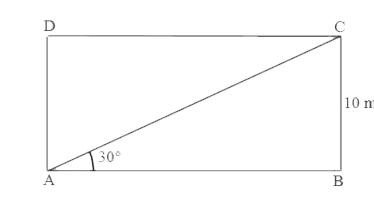
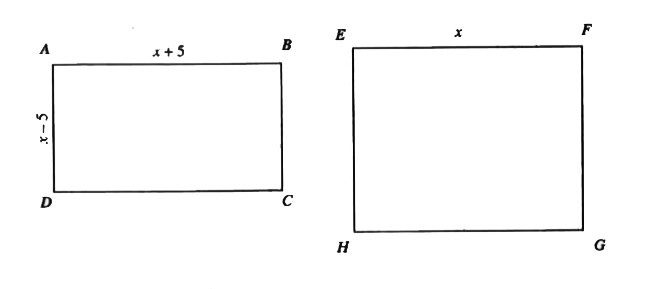
In the given figure find the perimeter of rectangle ABCD.
View SolutionIf a rectangle is inscribed in an equilateral triangle of side length 2√2 as shown in the figure, then the square of the largest area of such a rectangle is_______.

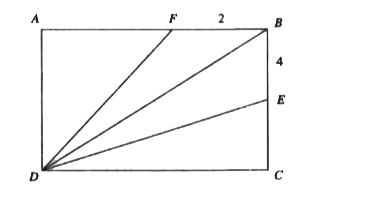
View SolutionIn the figure, ABCD is a rectangle, and F and E are points on AB and BC respectively. The area of ΔDFB is 9 and the area of ΔBED is 24. What is the perimeter of the rectangle?
View SolutionIn the figure, the area of rectangle ABCD is 45. what is the area of the square EFGH?
View SolutionThe perimeter of a rectangle and a square are 160 m each. The area of the rectangle is less than that of the square by 100 sq. m. The length of the rectangle is (a) 30m (b) 40m (c) 50m (d) 60m
View Solution

