Charge on capacitor is increased by 2C nad energy stored becomes 144%. Find the initial charge.
10
12
14
16
To solve the problem, we need to find the initial charge q on the capacitor given that the charge is increased by 2C and the energy stored becomes 144% of the initial energy.
1. Understanding the Energy Stored in a Capacitor:
The energy U stored in a capacitor is given by the formula:
U=q22C
where q is the charge on the capacitor and C is the capacitance.
2. Initial Energy Calculation:
Let the initial charge on the capacitor be q. Therefore, the initial energy stored in the capacitor is:
U=q22C
3. Charge After Increase:
When the charge is increased by 2C, the new charge becomes:
q′=q+2
4. New Energy Calculation:
The new energy stored in the capacitor after the increase in charge is:
U′=(q+2)22C
5. Energy Increase Condition:
According to the problem, the new energy U′ is 144% of the initial energy U:
U′=1.44U
6. Substituting the Energy Expressions:
Substitute the expressions for U and U′:
(q+2)22C=1.44⋅q22C
7. Canceling Common Terms:
Since 2C is common in both sides, we can cancel it out:
(q+2)2=1.44q2
8. Expanding the Left Side:
Expand the left side:
q2+4q+4=1.44q2
9. Rearranging the Equation:
Rearranging gives:
0=1.44q2−q2−4q−4
0=0.44q2−4q−4
10. Multiplying Through by 100:
To eliminate the decimal, multiply the entire equation by 100:
0=44q2−400q−400
11. Using the Quadratic Formula:
We can use the quadratic formula q=−b±√b2−4ac2a where a=44,b=−400,c=−400:
b2−4ac=(−400)2−4⋅44⋅(−400)
=160000+70400=230400
q=400±√23040088
=400±48088
12. Calculating the Roots:
This gives two potential solutions:
q1=88088=10andq2=−8088 (not valid as charge cannot be negative)
13. Final Answer:
The initial charge q on the capacitor is:
q=10 C
The energy U stored in a capacitor is given by the formula:
U=q22C
where q is the charge on the capacitor and C is the capacitance.
Let the initial charge on the capacitor be q. Therefore, the initial energy stored in the capacitor is:
U=q22C
When the charge is increased by 2C, the new charge becomes:
q′=q+2
The new energy stored in the capacitor after the increase in charge is:
U′=(q+2)22C
According to the problem, the new energy U′ is 144% of the initial energy U:
U′=1.44U
Substitute the expressions for U and U′:
(q+2)22C=1.44⋅q22C
Since 2C is common in both sides, we can cancel it out:
(q+2)2=1.44q2
Expand the left side:
q2+4q+4=1.44q2
Rearranging gives:
0=1.44q2−q2−4q−4
0=0.44q2−4q−4
To eliminate the decimal, multiply the entire equation by 100:
0=44q2−400q−400
We can use the quadratic formula q=−b±√b2−4ac2a where a=44,b=−400,c=−400:
b2−4ac=(−400)2−4⋅44⋅(−400)
=160000+70400=230400
q=400±√23040088
=400±48088
This gives two potential solutions:
q1=88088=10andq2=−8088 (not valid as charge cannot be negative)
The initial charge q on the capacitor is:
q=10 C
Topper's Solved these Questions
Similar Questions
A charged capacitor is connected to an inductor . At a particular instant, energy stored in inductor is 8/9 times of initial energy stored In capacitor . What is the ratio of initial charge on capacitor to that with instantaneous charge on capacitor ?
View SolutionIf the charge on a body is increased by an amount of 6C ,the energy stored in it increases by 44% .The original charge on the body ?
View Solution
Knowledge Check
If the charge on a capacitor is increased by 2C, then the energy stored in it increases by 21 %. The original charge on the capacitor is
A10 CB20 CC30 CD40 CThe charge on a 48 μF capacitor is increased from 0.1 C to 0.5 C. the enerrgy stored in the capacitor increases by
A250 JB2500 JC2.5×106JD2.42×10−6JIf a 4μF capacitor is charged to 1KV, then energy stored is conductor is
A1JB8JC4JD2J
The capacitance of a charged capacitor is C and the energy stored in it is U. What is the value of charge on the capacitor?
View SolutionA battery of 10V is connected to a capacitor of capacity 0.lF. The battery is now removed and this capacitor is connected to a second uncharged capacitor. If the charges are distributed equally on these two capacitors, find the total energy stored in the two capacitors. Find the ratio of final energy to initial energy stored in capacitors.
View SolutionA capacitor of unknown capacitance is connected across a battery of V volts . The charge stored in it is 360μC . When potential across the capacitor is reduced by 120 V , the charge stored in it becomes 120 μC . Calculate :
What will be the charge stored in the capacitor , if the voltage applied had increased by 120 V ?
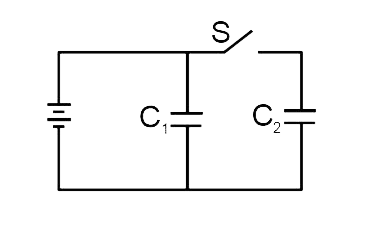
View SolutionTwo capacitors of equal capacitance (C1=C2) are shown in the figure. Initially, while the switch S is open, one of the capacitors is uncharged and the other carries charge Q0. The energy stored in the charged capacitor is U0. Sometimes after the switch is colsed, the capacitors C1andC2 carry charges Q1andQ2, respectively, the voltages across the capacitors are V1andV2, and the energies stored in the capacitors are U1andU2. Which of the following statements is INCORRECT ?
View SolutionIf a 4μF capacitor is charged to 1 kV, then energy stored in the capacitor is
View Solution
