If the metal has threshold frequency of 5×1014Hz the find out the work function of metal.
To find the work function of the metal given its threshold frequency, we can follow these steps:
1. Understand the relationship between work function and threshold frequency: The work function (φ) of a metal is related to its threshold frequency (ν₀) by the equation:
φ=hν₀
where h is Planck's constant.
2. Identify the given values:
- Threshold frequency, ν₀=5×1014Hz
- Planck's constant, h=6.63×10−34J s
3. Substitute the values into the equation:
φ=(6.63×10−34J s)×(5×1014Hz)
4. Calculate the work function:
- First, multiply the constants:
φ=6.63×5×10−34×1014
- Calculate 6.63×5=33.15
- Combine the powers of ten:
φ=33.15×10−34+14=33.15×10−20J
5. Convert to electron volts: To convert joules to electron volts, we use the conversion factor 1eV=1.6×10−19J:
φ=33.15×10−201.6×10−19eV
6. Perform the division:
φ≈2.07eV
Final Answer
The work function of the metal is approximately 2eV.
φ=hν₀
where h is Planck's constant.
- Threshold frequency, ν₀=5×1014Hz
- Planck's constant, h=6.63×10−34J s
φ=(6.63×10−34J s)×(5×1014Hz)
- First, multiply the constants:
φ=6.63×5×10−34×1014
- Calculate 6.63×5=33.15
- Combine the powers of ten:
φ=33.15×10−34+14=33.15×10−20J
φ=33.15×10−201.6×10−19eV
φ≈2.07eV
The work function of the metal is approximately 2eV.
Topper's Solved these Questions
Similar Questions
Find the frequency of light which ejects electrons from a metal surface. Fully stopped by a retarding potential of 3V, the photoelectric effect begins in this metal at a frequency of 6×1014Hz. Find the work function for this metal. (Given h=6.63×10−34Js).
View SolutionThe threshold frequency of a material is 2×1014 Hz. What is its work function in eV ?
View Solution
Knowledge Check
A photon of energy 8eV is incident on a metal surface of threshold frequency 1.6×1015Hz, then the maximum kinetic energy of photoelectrons emitted is (h=6.6×10−34Js)
A4.8eVB2.4eVC1.4eVD0.8eVThe threshold frequency for a photosensitive metal is 3×1014Hz. The work function of the metal is approximately equal to [h=6.66×10−34Js]
A0.5×10−19 JB1.0×10−19JC1.5×10−19JD2.0×10−19J
Find the frequency of light which ejects electrons from a metal surface stopped by a retarding potential of 3.3 V. if photoelectric emission begins in this metal at a frequency of 8×1014Hz, calculate the work function (in eV) for this metal.
View SolutionFind the frequency of light which ejects electrons from a metal surface , fully stopped by a retarding potential of 3.3 V. If photo electric emission begins in metal at a frequency of 8×1014Hz, calculate the work function (in eV) for this metal.
View SolutionIf a photoemissive surface has a threshold frequency of 3×1014 Hz, calculate the energy of the photons in eV.
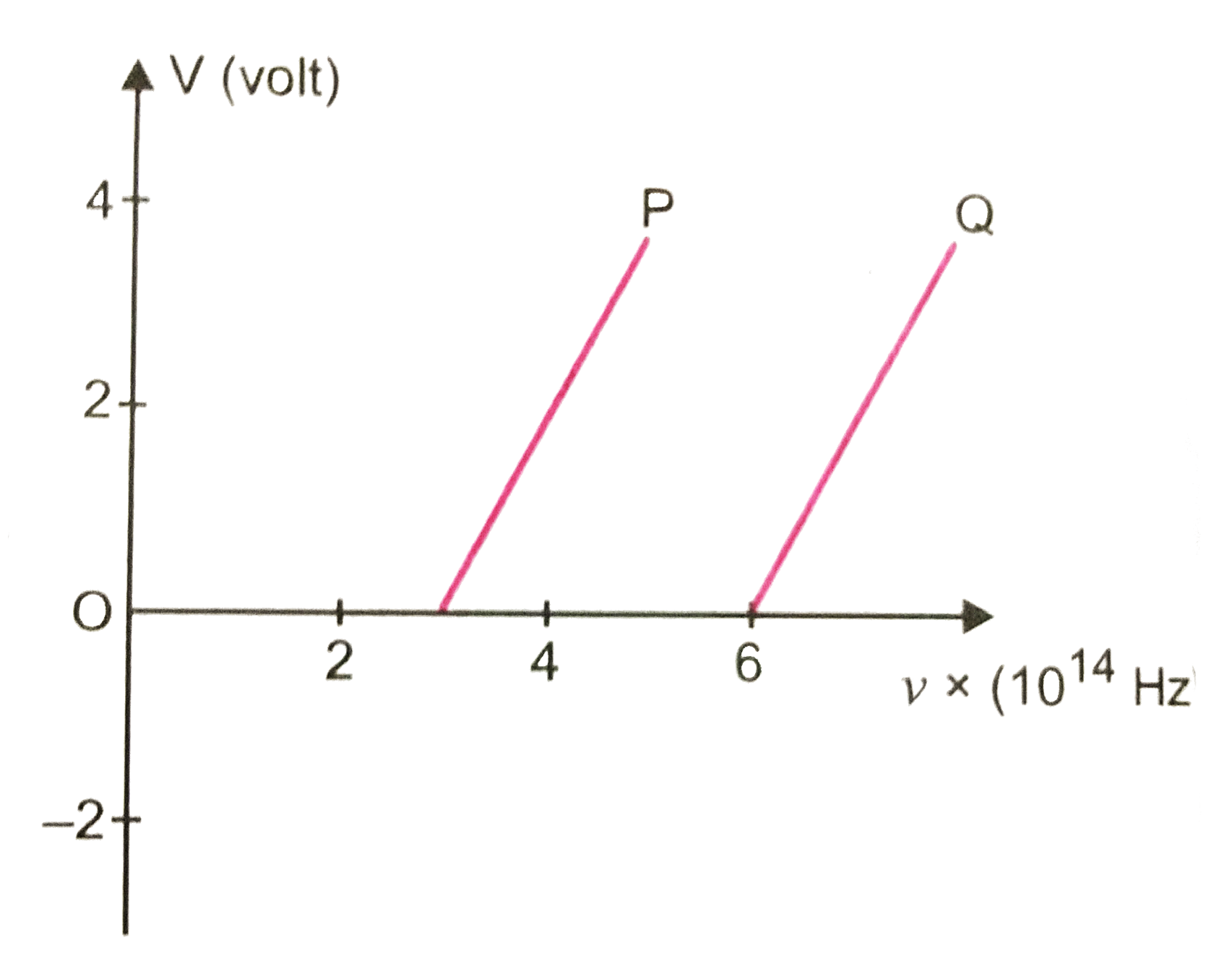
View SolutionIn the study of a photoelectric effect the graph between the stopping potential V and frequency v of the incident radiation on two different metals P and Q is shown in fig.
(i) which one of two metals have higher threshold frequency
(ii) Determine the work function of the metal which has greater value
(iii) Find the maximum kinetic energy of electron emitted by light of frequency 8×1014Hz for this metal.
View SolutionDetermine the de Broglie 's wavelenght of electron emitted by a metal whose threshold frequency is 2.25×1014Hz when exposed to visible radiation of wavelenght 500nm.
View SolutionThe minimum frequency for photoelectric effect on a metal is 7×1014 Hz, Find the work function of the metal.
View Solution
