Relation between time period of two satelites is TA=2TB then RARB is ?
R3A=4R3B
R2A=2R2B
R2A=3R2B
R2A=12R2B
To solve the problem, we need to use Kepler's third law of planetary motion, which states that the square of the time period (T) of a satellite is directly proportional to the cube of the semi-major axis (R) of its orbit. The relationship can be expressed mathematically as:
T2∝R3
This implies:
T2AT2B=R3AR3B
Given in the problem, we have the relationship between the time periods of two satellites:
TA=2TB
Step 1: Square the time period relationship
We start by squaring both sides of the equation:
T2A=(2TB)2=4T2B
Step 2: Substitute into Kepler's law
Now, we substitute this into the Kepler's law relationship:
T2AT2B=R3AR3B
Substituting T2A:
4T2BT2B=R3AR3B
Step 3: Simplify the equation
This simplifies to:
4=R3AR3B
Step 4: Rearranging the equation
Now, we can rearrange this equation to find the ratio of the radii:
R3A=4R3B
Step 5: Taking the cube root
Taking the cube root of both sides gives us:
RA=√(3)4RB
Step 6: Finding the ratio RARB
Thus, the ratio of RA to RB is:
RARB=√(3)4
Conclusion
The final answer is:
RARB=√(3)4
We start by squaring both sides of the equation:
Now, we substitute this into the Kepler's law relationship:
This simplifies to:
Now, we can rearrange this equation to find the ratio of the radii:
Taking the cube root of both sides gives us:
Thus, the ratio of RA to RB is:
The final answer is:
Topper's Solved these Questions
Similar Questions
A mass is suspended separately by two springs and the time periods in the two cases are T1 and T2. Now the same mass is connected in parallel (K=K1+K2) with the springs and the time is suppose TP. Similarly time period in series is TS, then find the relation between T1,T2 and TP in the first case and T1,T2 and TS in the second case.
View SolutionWhat is the relation between the period of rotation (RT) and periond of revolution (RV) of moon?
View Solution
Knowledge Check
Relation between time period (T) and frequency (V)
AV=1TBV=TCV=1T2DVT=1If g∝1R3 (instead of 1R2), then the relation between time period of a satellite near earth's surface and radius R will be
AT2∝R3BT∝R2CT2∝RDT∝RWhich of the following graph depicts relation between time period (T) and radius of orbit (r) of a planet ?
A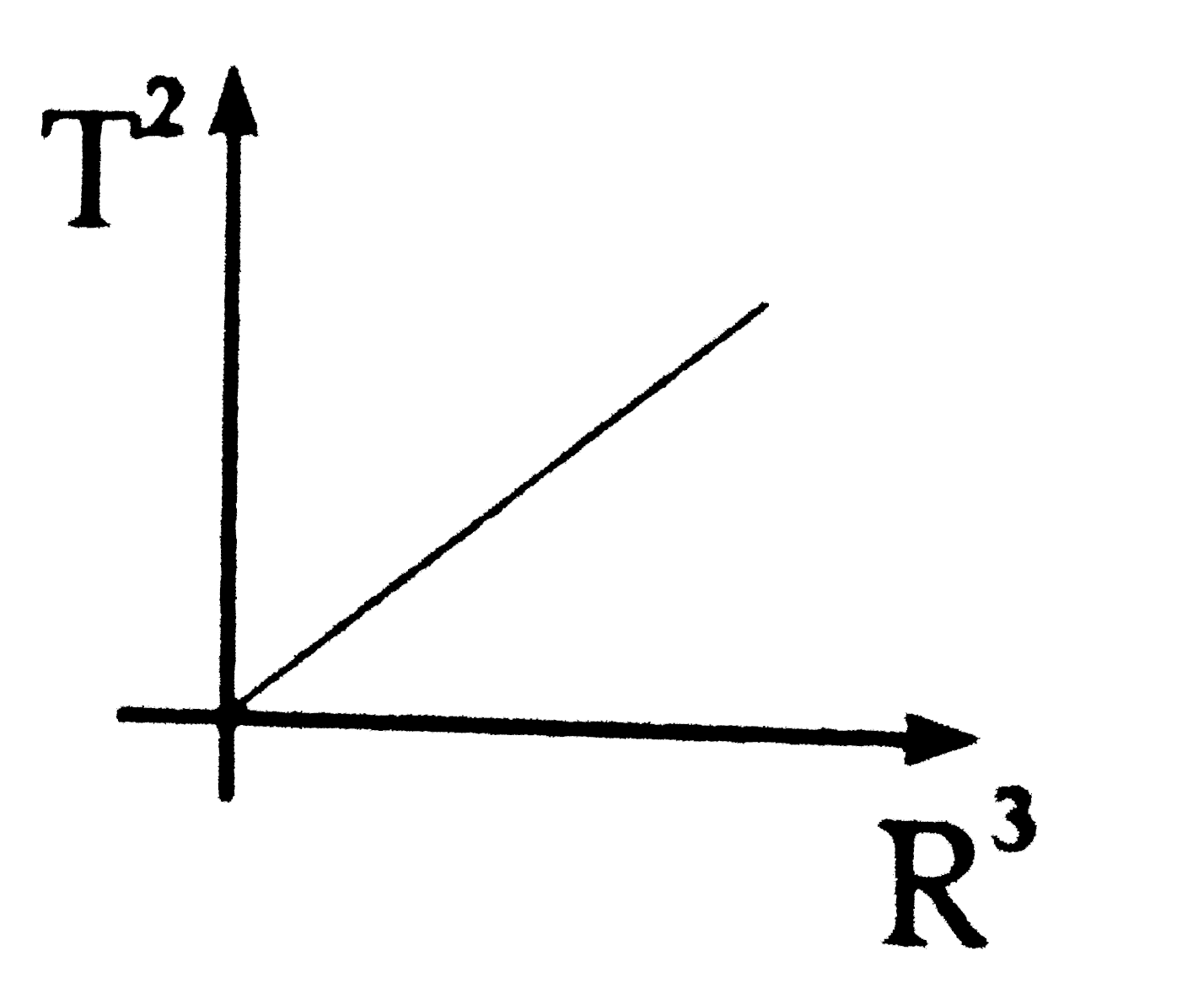 B
B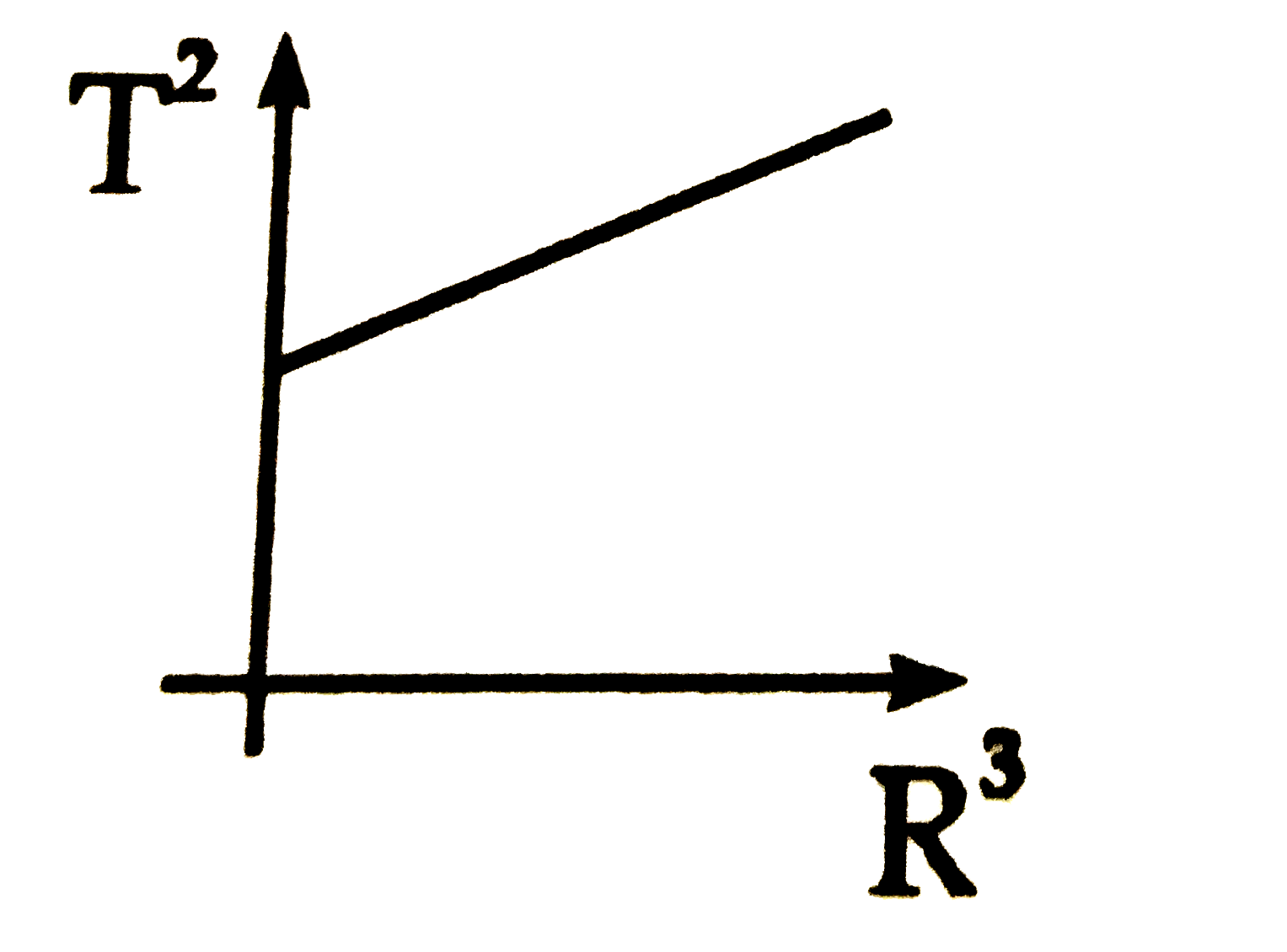 C
C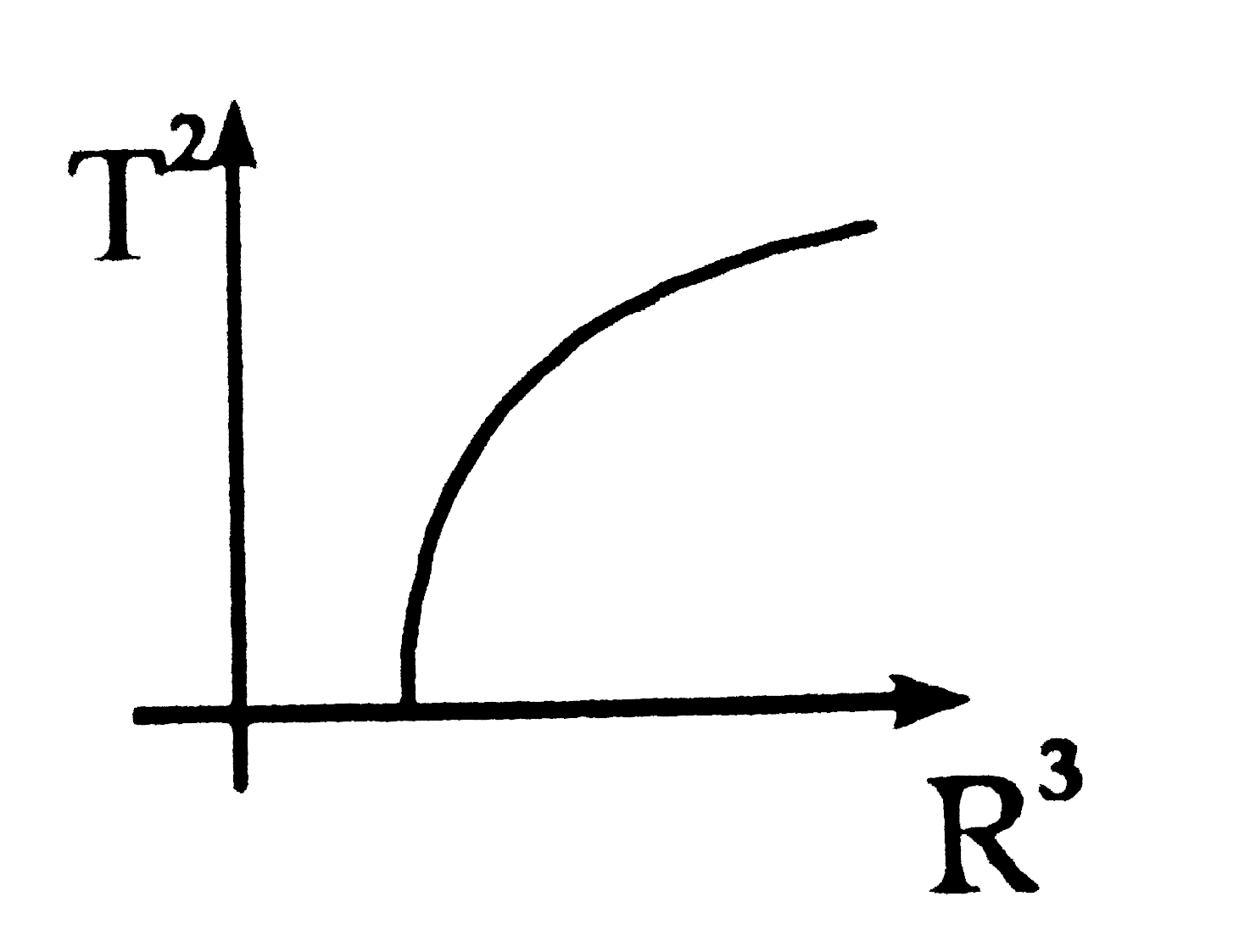 D
D
The relation between time t and distance x is t=ax2+bx where aandb are constants. The acceleration is
View SolutionA particle is moving in a circle of radius R with constant speed. The time period of the particle is T. In a time t=T6
Average velocity of the particle is…..
View SolutionA particle is moving in a circle of radius R with constant speed. The time period of the particle is T. In a time t=T6
Average speed of the particle is ……
View SolutionWhat is the relation between period of rotation (RT) of earth and period of revolution (Rr) for a geostationary satellite?
View SolutionTwo satellites A and B of masses m1 and m2(m1=2m2) are moving in circular orbits of radii r1 and r2(r1=4r2), respectively, around the earth. If their periods are TA and TB, then the ratio TA/TB is
View Solution
