Three identical particle each of mass 100g are placed at vertices of an equilateral triangle of side length 13cm. Find force on one of the mass.
a.100√3G169
b.150√3G169
c.110√3G189
d.170√3G229
To find the force on one of the masses placed at the vertices of an equilateral triangle, we can follow these steps:
Step 1: Understand the Setup
We have three identical particles, each with a mass m=100g=0.1kg, placed at the vertices of an equilateral triangle with a side length of a=13cm=0.13m.
Step 2: Calculate the Gravitational Force Between Two Masses
The gravitational force F between any two masses m1 and m2 separated by a distance r is given by Newton's law of gravitation:
F=Gm1m2r2
In our case, since all masses are identical and equal to m, the force between any two masses is:
F=Gm2a2
Step 3: Determine the Angle Between Forces
Since we are looking for the net force on one of the masses due to the other two, we need to consider the angle between the forces exerted by the other two masses. In an equilateral triangle, the angle between the lines connecting the mass to the other two masses is 60∘.
Step 4: Resolve Forces into Components
Let’s denote the force exerted on the mass at vertex A by the mass at vertex B as FAB and by the mass at vertex C as FAC. Both forces have the same magnitude F but act at an angle of 60∘ to each other.
The x-components of the forces will add up, while the y-components will also add up. The net force Fnet can be calculated using the formula for the resultant of two vectors:
Fnet=√F2AB+F2AC+2FABFACcos(60∘)
Since FAB=FAC=F:
Fnet=√F2+F2+2F2⋅12=√3F2=√3F
Step 5: Substitute the Value of F
Substituting F=Gm2a2:
Fnet=√3⋅Gm2a2
Step 6: Plug in the Values
Now we can substitute the values for G, m, and a:
- G=6.674×10−11N m2/kg2
- m=0.1kg
- a=0.13m
Calculating Fnet:
Fnet=√3⋅6.674×10−11⋅(0.1)2(0.13)2
=√3⋅6.674×10−11⋅0.010.0169
=√3⋅6.674×10−11⋅0.592
Calculating this gives us the final value for the net force on one mass.
Final Calculation
After performing the calculations, we find that:
Fnet≈100√3G169
Conclusion
Thus, the force on one of the masses is given by:
Fnet=100√3G169
We have three identical particles, each with a mass m=100g=0.1kg, placed at the vertices of an equilateral triangle with a side length of a=13cm=0.13m.
The gravitational force F between any two masses m1 and m2 separated by a distance r is given by Newton's law of gravitation:
Since we are looking for the net force on one of the masses due to the other two, we need to consider the angle between the forces exerted by the other two masses. In an equilateral triangle, the angle between the lines connecting the mass to the other two masses is 60∘.
Let’s denote the force exerted on the mass at vertex A by the mass at vertex B as FAB and by the mass at vertex C as FAC. Both forces have the same magnitude F but act at an angle of 60∘ to each other.
Substituting F=Gm2a2:
Now we can substitute the values for G, m, and a:
- m=0.1kg
- a=0.13m
After performing the calculations, we find that:
Thus, the force on one of the masses is given by:
Topper's Solved these Questions
Similar Questions
Three identical particles each of mass m are placed at the vertices of an equilateral triangle of side a. Fing the force exerted by this system on a particle P of mass m placed at the

(a) the mid point of a side
(b) centre of the triangle
View SolutionThree particles, each of mass m are fixed at the vertices of an equilateral triangle of side length a. The only forces acting on the particles are their mutual gravitational forces. Then answer the following questions.
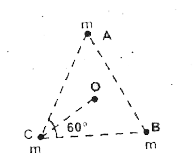
Force acting on particle C, due to particle A and B
View Solution
Knowledge Check
Three identical masses, each of mass 1kg, are placed at the corners of an equilateral triangle of side l. Then the moment of inertia of this system about an axis along one side of the triangle is
A3l2Bl2C34l2D32l2Three equal masses of 1 kg each are placed at the vertices of an equilateral triangle of side 1 m, then the gravitational force on one of the masses due to other masses is (approx.)
A{6.67(10−11)}NB10−3 NC[11.5(10−11)]ND[11.5(10−3)]N
Three particles, each of mass m are fixed at the vertices of an equilateral triangle of side length a. The only forces acting on the particles are their mutual gravitational forces. Then answer the following questions.
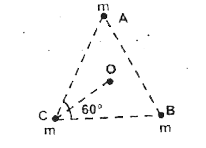
The gravitational potential at O is
View SolutionThree identical particles, each of mass m, are located in space at the vertices of an equilateral triangle of side length a. They are revolving in a circular orbital under mutual ravitational attraction. Find the speed of each particle. Find the acceleration of the centre of mass of a system comprising of any two particles. Assume that one of the particles suddenly loses its ability to exert gravitational force. Find the velocity of the centre of mass of the system of other two particles after this.
View SolutionThree particles each of mass 12 g are located at the vertices of an equilateral triangle of side 10 cm. Calculate the moment of inertia of the system about an axis passing through the centroid and perpendicular to the plane of the triangle?
View SolutionThree particles, each of mass 200 g are kept at the corners of an equilateral triangle of side 10 cm. Find the moment of inertia of the system about an axis
a. joining two of the particles and
passing through lone of the particle and perpendicular to the plane of the particles.
View SolutionThree identical point objects each of mass m are placed at the vertices of an equilateral triange of side l. What is the gravitational potential at the centre of the equilateral triangle due to the point masses?
View SolutionThree particles, each of mass m are situated at the vertices of an equilateral triangle ABC of side L figure. Find thee moment of inertia of the system about the line AX perpendicular to AB in the plane of ABC

View Solution